Arctan 公式 – 定义、公式、示例问题
三角学是一门与直角三角形的边和角的评估和演示有关的研究。三角学中的运算是通过边、角和三角比来进行的。这些三角比是三角函数的值,它是从给定三角形的边和角的比值中得出的。
三角学具有基本的三角函数,这些函数在各种角度下都有自己的标准三角比值。要知道的基本函数是正弦、余弦、正切、余切、余割和正割。并且,这些三角函数的逆函数用前缀“arc-”表示,例如 arcsin、arccos、arctan、arccot、arcsec 和 arccosec。
什么是反正切公式?
切线是函数,在直角三角形中,切线等于底边垂直(垂直/底边),并给出角度的值。
Arctan 是对正切的反函数的引用。象征性地,arctan 在三角方程中由 tan -1 x 表示。
假设角度 θ 的正切等于 x。
那么,x =tanθ
=> θ =tan -1 x
让我们取一个角度为 θ 的直角三角形 QPR。现在,正如我们研究的那样,切线等于垂直于底边。
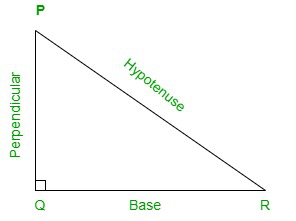
即tan θ =p/b
并且,通过使用相同的值,我们可以确定角度和反正切的值。
As, tan θ =p/b
=> θ =tan -1 (p/b)
arctan 公式列表
反正切公式可以从度数或弧度导出。这些公式有助于求解反三角方程。
- θ =arctan(垂直/底)
- arctan(-x)=-arctan(x) 对于所有 x∈ R
- tan(arctan x)=x ,对于所有实数
- arctan(1/x)=π/2 – arctan(x) = arccot(x);如果 x>0
(要么)
- arctan(1/x)=-π/2 – arctan(x) = arccot(x) -π;如果 x<0
- sin(arctan x)= x/ √(1+x 2 )
- cos(arctan x)=1/ √(1+x 2 )
- arctan(x)=2arctan(\frac{x}{1+\sqrt(1+x^2)})
- arctan(x)=\int^x_0\frac{1}{z^2+1}dz
\pi 也有一些标准的反正切公式。下面列出了这些公式。
- π/4 = 4 反正切(1/5) – 反正切(1/239)
- π/4 = arctan(1/2) + arctan(1/3)
- π/4 = 2 反正切(1/2) – 反正切(1/7)
- π/4 = 2 反正切(1/3) + 反正切(1/7)
- π/4 = 8 arctan(1/10) – 4 arctan(1/515) – arctan(1/239)
- π/4 = 3 arctan(1/4) + arctan(1/20) + arctan(1/1985)
正如我们上面研究的那样,arctan 的值可以通过度数或弧度得出。因此,下表说明了 arctan 的估计值。 arctan(x) (in degree) Arctan(x) (in radian) x -∞ -90° -π/2 -3 -71.565° -1.2490 -2 -63.435° -1.1071 -√3 -60° -π/3 -1 -45° -π/4 -1/√3 -30° -π/6 -1/2 -26.565° -0.4636 0 0° 0 1/2 26.565° 0.4636 1/√3 30° π/6 1 45° π/4 √3 60° π/3 2 63.435° 1.1071 3 71.565° 1.2490 ∞ 90° π/2
示例问题
问题 1. 评估 tan -1 (1)。
解决方案:
Given
tan-1(1)
And, the value 1 can also be written as
1 = tan(45°)
Now,
=>tan-1(1) = tan-1(45°)
=>45°
问题 2. 评估 tan -1 (0.577)。
解决方案:
Given
tan-1(0.577)
And, the value of 0.577 can also be written as
=>0.577=tan(30°)
Now,
=>tan-1(0.577)=tan-1(30°)
=>30°
问题 3. 计算 tan -1 (1.732)。
解决方案:
Given
tan-1(1.732)
And, the value of 1.732 can also be written as
=>1.732=tan(60°)
Now,
=>tan-1(1.732)=tan-1(60°)
=>60°
问题 4. 评估 tan -1 (0)。
解决方案:
Given
tan-1(0)
And, the value of 0 can also be written as
=>0 =tan(0°)
Now,
=>tan-1(0)=tan-1(0°)
=>0°