分段函数的定积分

想象一个图，上面画了一个函数，它可以是直线或曲线或任何东西，只要它是一个函数。现在，这只是图上的一个函数，图上可以同时出现两个函数吗？想象两个函数同时出现在图形上，比如一条直线和一条曲线，并且它们出现在 x 轴上的界限是相同的，这可以称为函数吗？不，单个输入的函数的两个值不是 Function 。那么，如何在一个图上排列两个函数呢？很简单，只要确保它们存在的限制是不同的而不是相同的，这些类型的函数在不同的 x 轴值上中断或两个或两个以上的函数定义在不同的限制被称为分段函数。

确定的整合

积分是找到函数和轴之间的区域（可以是基于函数的x轴或y轴）。积分可以是有限的或无限的，对于无限函数，存在到无穷大或从无穷大开始，此类函数的区域称为不定积分，而如果应用一些边界将函数限制在轴上存在的某个有限值，它将被称为定积分。

明确的整合有开始和结束的界限。假设极限从 a 到 b。从 a 到 b 的函数应如下所示，

Integration is given by ⇢ $\int^b_a{f(x)dx}$

示例：求函数y=4x 的图形下方的区域，边界定义为 x 轴上从 0 到 5。通过积分求解和简单地使用公式求解面积。

解决方案：

The function on the graph shall look like,

The above graph shows exactly what area is to be measured,

Integral of y=4x from 0 to 5 = $\int^5_0{4xdx}\\=4[\frac{x^2}{2}]^5_0\\=2[25-0]\\=50unit^2$

The area looks like a triangle and hence, another way of solving this is by simply finding the area of the triangle.

For height of the triangle, at x = 5, y = 4 × 5 = 20 units.

Base = 5 units, Height = 20 units.

Area of the triangle = 1/2 × 5 × 20

= 10 × 5

= 50 units²

分段函数的定积分

为了分段集成函数，需要在确切的断点处中断集成。打断积分会给不同的上下限提供两种不同的函数，并且很容易将它们分开积分。对于两个以上的函数也可以这样做，在这种情况下，断点和积分的数量会增加。

分段函数可以表示为，

$f(x)= \begin{cases} f_1(x), a\leq x \leq b\\ f_2(x), b<x \leq c \end{cases}$

$\int^c_af(x)dx= \int_a^bf_1(x)dx+ \int_b^cf_2(x)dx$

这里，极限在c处有一个断点，两个函数分别是f ₁ (x)和f ₂ (x)。

上述条件函数具有固定端点的定积分。

具有固定下端点、可变上端点的函数

$f(x)= \begin{cases} f_1(x), if x<a\\ f_2(x), if x>a \end{cases}$

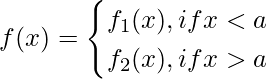
分段函数定积分的应用

下面是一些在数学中很常见的分段函数的例子，

• 模函数,|x|

Modulus function are the functions that break at a point, and they are represented as,

$|x|= \begin{cases} x, x\geq 0\\ -x, x<0 \end{cases}$

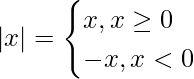
• 更大的整数函数，[x]

In definite integrals, if the value obtained is some decimal, the greater integer condition will bring out the integer part of the value, for example, [8.34] will give 8.

• 函数的小数部分，{x}

Fractional part of x is derived or obtained from greater integer of the function only.

It is defined as, {x} = x-[x]

示例问题

问题 1：一个条件函数给出为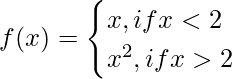， $f(x)= \begin{cases} x, if x<2\\ x^2, if x>2 \end{cases}$

求定积分$\int_0^4f(x)dx$

解决方案：

The above conditional function has two functions involved, one is a straight line and the other is a parabola, the break point of the limit is 2 and below 2, the function is a straight line x, above 2 or after 2, it is a parabola x².

Keeping this in mind the definite integral can be easily broken as,

$\int_0^4f(x)dx= \int_0^2xdx + \int_2^4x^2dx \\=[\frac{x^2}{2}]_0^2+[\frac{x^3}{3}]_2^4 \\=2+21.333-2.666\\=20.667$

问题 2：求定积分$\int_{-1}^1 f(x)dx$ ， 在哪里$f(x)= \begin{cases} 1, -1\leq x \leq0\\ 3+x^2, 0<x \leq 1 \end{cases}$

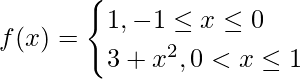
解决方案：

The above function has two different functions involved on different limits, the breakpoint of the limit is 0, and before to -1, the function is 1, and after 0 to 1, the function is 3+x².

Let’s solve,

$\int_{-1}^1f(x)dx= \int_{-1}^01dx + \int_0^13+x^2dx\\=[x]_{-1}^0+[3x]_0^1+[\frac{x^3}{3}]_0^1\\=1+[3-0]+[1/3-0]\\=1+3+1/3\\=4+1/3\\=\frac{13}{3}$

问题3：在定积分的帮助下找到下面给出的函数的区域，

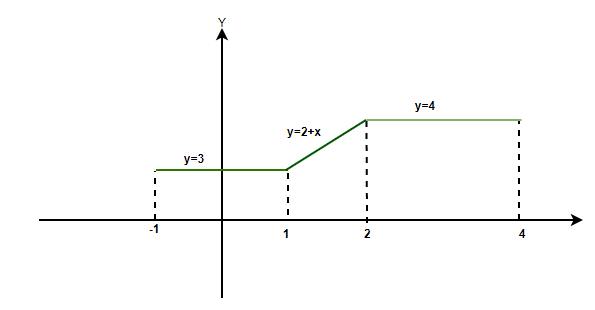
解决方案：

There are three different functions from -1 to 4, y=3, y=2+x, y=4. Let’s integrate these function with the help of piecewise integration of functions.

$\int_{-1}^4f(x)dx= \int_{-1}^13dx + \int_1^22+xdx + \int_2^44dx\\=[3x]_{-1}^1+[2x]_1^2+[\frac{x^2}{2}]_1^2+[4x]_2^4\\=6+3+2-0.5+16-8\\=19-0.5\\=18.5$

问题4：解决集成$\int_{-1}^1f(x)dx$当给定函数时，f(x)= |x|。

解决方案：

The integration for modulus of x or |x| can be solved only after knowing the meaning of |x|, |x| is defined as,

$|x|= \begin{cases} x, x\geq 0\\ -x, x<0 \end{cases}$

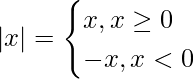
Hence, the function can be broken as,

$f(x)= \begin{cases} x, x\geq 0\\ -x, x<0 \end{cases}$

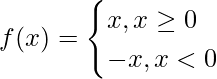
$\int_{-1}^1f(x)dx= \int_{-1}^0-xdx+\int_{0}^1xdx\\=-[\frac{x^2}{2}]_{-1}^0+[\frac{x^2}{2}]_0^1\\=-[0-1/2]+[1/2]\\=1/2+1/2 \\=1$

问题 5：评估给定的函数， $I= \int_0^3|2-x|dx$

解决方案：

The above function is a modulus function and hence, it will have break point, lets find out the break point for |2-x|,

As it is known that the modulus function will have 2 values, one positive and one negative, but it is important to find out where the values or the function as a whole starts to change,

The lower limit is 0, if 0 is put, the value obtained is 2 which is positive, now put 1, value obtained is 1, but if any value above 2 is put, the value if negative, hence, the break point is 2.

$f(x)= \begin{cases} 3-x, 0 \leq x \geq 2\\ x-3, 2<x \leq 3\end{cases}$

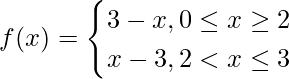
$\int_0^3|3-x|dx= \int_0^2(3-x)dx+\int_2^3(x-3)dx\\=[3x]_0^2-[\frac{x^2}{2}]_0^2+[\frac{x^2}{2}]_2^3-[3x]_2^3\\=6-2+9/2-2+9-6\\=9.5$