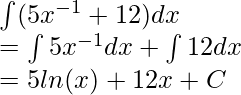积分

微积分以导数的概念为中心，导数衡量函数不同值的变化率。从几何上讲，它们是任意点切线的斜率。积分是微分的逆过程，积分是由和驱动的，就像导数计算差一样，积分计算不同函数的和，现在这个和可以是任何东西。它主要用于计算任意形状的面积和体积。让我们详细看看这些概念。

积分简介

考虑一个函数“f”，它在区间“I”中是可微的，这意味着它的导数存在于区间 I 中的所有点。现在，想到的问题是，如果函数在每个点的导数给出，是否可以再次生成函数？该函数将被称为反导数。我们生成这些函数的过程称为积分，而给出这些反导数的公式称为不定积分。

积分作为微分的逆

考虑下面给出的一些函数的导数，

$\frac{d}{dx}(x^2) = 2x \\ \frac{d}{dx}(sin(x)) = cos(x) \\ \frac{d}{dx}(cos(x)) = -sin(x)$

在上述等式中，考虑$\frac{d}{dx}(x^2) = 2x$ ，这里 x ²是函数f'(x) = 2x 的反导数。这也适用于上述衍生物。

请注意，如果将这些函数与常数相加，则导数没有区别，因为常数的导数为零。

$\frac{d}{dx}(x^2 + C) = 2x \\ \frac{d}{dx}(sin(x) + C) = cos(x) \\ \frac{d}{dx}(cos(x) + C) = -sin(x)$

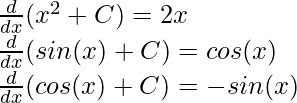
因此，可以得出结论，对于任何函数，它的反导数都是无限的。例如，对于函数f(x)，令其反导数为 F(x)，

$\int f(x) = F(x) + C$

$\int$表示积分。这将代表对任何函数的积分操作。下表表示与积分有关的符号和含义。

Symbol/Term/Meaning	Meaning
$\int f(x)dx$	Integral of f with respect to x
f(x) in $\int f(x)dx$	Integrand
x in $\int f(x)dx$	Variable of integration
Integral of f(x)	A function such that F'(x) = f(x)

一些标准函数的性质和积分

我们每天都会遇到很多函数，学习积分的性质以简化积分过程变得至关重要。还需要记住一些标准函数的积分，以便更容易求解通常由一些基本简单函数组成的复杂积分。下表列出了标准函数的一些积分。

Function	Integral
xn	$\frac{x^{n+1}}{n +1}$
sin(x)	-cos(x)
cos(x)	sin(x)
ex	ex
sec2(x)	tan(x)
$\frac{1}{x}$	ln(x)

积分的性质：

Property 1: $\int kf(x)dx = k\int f(x)dx$

Property 2: $\int(f(x) \pm g(x))dx = \int f(x)dx \pm \int g(x)dx$

Property 3: $\int(f_1(x) \pm f_2(x) \pm f_3(x) \pm ...)dx = \int f_1(x)dx \pm \int f_2(x)dx + ....$

积分的几何解释

积分通常用于计算曲线下的面积。尽管有可用的公式，但它们仅适用于标准形状。经常会遇到任意复杂的形状，不可能为每个形状开发和记住一个公式。因此，积分有助于概括计算面积和体积的方法。考虑一个函数f(x)，目标是计算函数的面积。

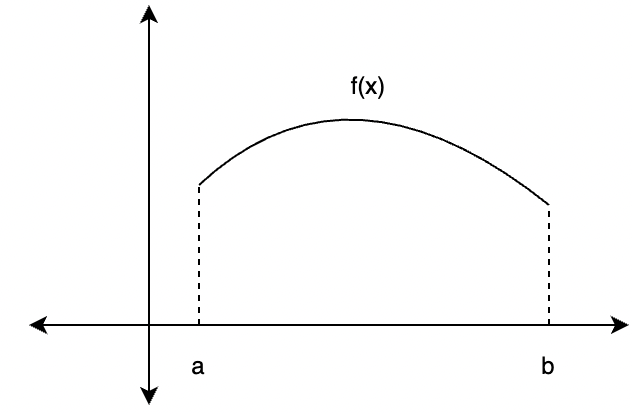
函数被分成矩形形状的不同部分，这些部分相加形成区域。每个矩形的宽度为$\Delta x$

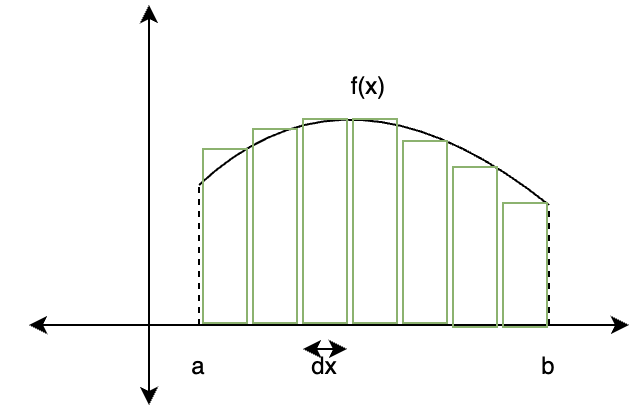
随着矩形数量的增加，这些矩形的宽度变得非常小，可以用“dx”表示。每个矩形的面积变为 f(x)dx。总面积是所有这些小矩形的面积之和，

$A(x) = \sum f(x)dx = \int f(x)dx$

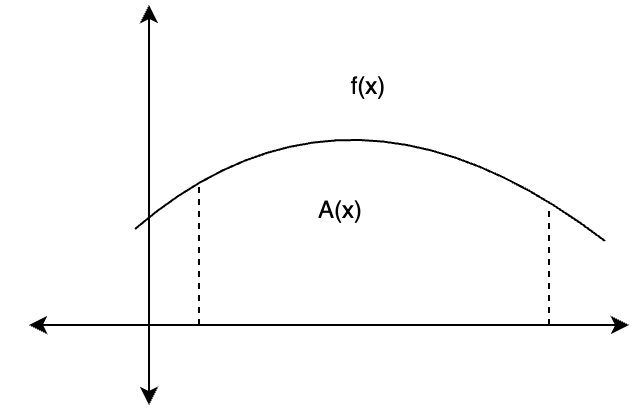
让我们看一些示例问题

示例问题

问题 1：求给定函数f(x) 的积分，

f(x) = 2sin(x) + 1

解决方案：

Given f(x) = 2sin(x) + 1

sin(x) is a standard function, and its integral is known. 

$\int f(x)dx$

⇒$\int (2sin(x) + 1)dx$

Using the property 2 mentioned above, 

$\int 2sin(x)dx + \int 1dx$

⇒$-2cos(x) + x + C$

问题 2：求给定函数f(x) 的积分，

f(x) = 2x ² + 3x

解决方案：

Given f(x) =2x² + 3x

2x² + 3x is a polynomial function, and it can be broken down in different parts. 

$\int f(x)dx$

⇒$\int (2x^2 + 3x)dx$

Using the property 1 mentioned above, 

$\int 2x^2dx + \int 3xdx$

$\frac{x^3}{3} + \frac{3x^2}{2} + C$

问题 3：求给定函数f(x) 的积分，

f(x) = x ³ + 3x ² + x + 1

解决方案：

Given f(x) = x³ + 3x² + x + 1

x³ + 3x² + x + 1 is a polynomial function, and it can be broken down in different parts. 

$\int f(x)dx$

⇒$\int (x^3 + 3x^2 + x + 1)dx$

Using the property 1 mentioned above, 

$\int (x^3 + 3x^2 + x + 1)dx \\ = \int x^3dx + \int 3x^2dx + \int xdx + \int1dx \\ = \frac{x^4}{4} + \frac{3x^3}{3} + \frac{x^2}{2} + x + C \\ = \frac{x^4}{4} + x^3 + \frac{x^2}{2} + x + C$

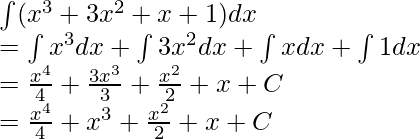
问题 4：求给定函数f(x) 的积分，

f(x) = 5x ^-2 + x + 1

解决方案：

Given f(x) = 5x^-2 + x + 1

Using the reverse power rule

$\int f(x)dx$

⇒$\int (5x^{-2} + x + 1)dx$

Using the property 1 mentioned above, 

$\int 5x^{-2}dx + \int xdx + \int 1dx \\ = \frac{-5}{x} +\frac{x^2}{2} + x + C$

问题 5：求给定函数f(x) 的积分，

f(x) = sin(x) + 5cos(x)

解决方案：

Given f(x) = sin(x) + 5cos(x)

sin(x) and cos(x) are standard functions, and its integral is known. 

$\int f(x)dx$

⇒$\int (sin(x) + 5cos(x))dx$

Using the properties 1 and 2 mentioned above, 

$\int sin(x)dx + 5\int cos(x)dx$

⇒$-cos(x) + 5sin(x) + C$

问题 6：求给定函数f(x) 的积分，

f(x) = 5x ^-2 + x ⁴ + x

解决方案：

Given f(x) = 5x^-2 + x⁴ + x

Using the reverse power rule

$\int f(x)dx$

⇒$\int (5x{-2} + x^4 + x)dx$

Using the properties 1 and 2 and power rule mentioned above, 

$\int (5x{-2} + x^4 + x)dx$

⇒$5\int x^{-2}dx + \int x^4dx + \int xdx$

⇒$\frac{-5}{x} + \frac{x^5}{5} + \frac{x^2}{2} + C$

问题 7：求给定函数f(x) 的积分，

f(x) = 5x ^-1 + 12

解决方案：

Given f(x) = 5x^-1 + 12

Using the reverse power rule

$\int f(x)dx$

⇒$\int (5x^{-1} + 12)dx$

Using the properties 1 and 2

$\int (5x^{-1} + 12)dx \\ = \int 5x^{-1}dx + \int 12dx \\ = 5ln(x) + 12x + C$