道路公式银行

对于在弯曲道路上高速行驶的车辆，道路倾斜是理想的解决方案。弯道时，道路外侧边缘被推高，高于内侧边缘，路面类似平缓倾斜的平面，以保证车辆的安全。这个过程被称为道路银行或道路银行。

换句话说，道路倾斜是指将道路的外边界（边缘）以特定角度（这里的角度称为倾斜角度）高于其内边界（边缘）的技术。

道路银行的必要性

• 车辆在弯道行驶时，必须提供向心力，否则有被切向抛掷的危险。
• 车轮与路面的摩擦力可以提供向心力，但此类路面的安全速度很低，不可靠。
• 在道路倾斜中，正常反作用力的水平分量提供了必要的向心力，保护了车辆免受事故的影响。

与道路银行相关的公式

1. The velocity of a vehicle on a curved banked road: v = $\sqrt{(\frac{Rg(μs + tanθ)}{(1−μs tanθ)}}$
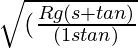
2. For a given pair of roads, and Tyre μ_s = tanλ, then the velocity of a vehicle on a curved banked road is: v = $\sqrt{Rg tan(θ + λ)}$
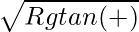
3. The safe velocity on an unbanked road is: v_max = $\sqrt{μ Rg}$
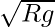
4. The expression for the angle of banking of road is: θ = tan^-1 [v₀² / Rg]
5. Expression for the safe velocity on the banked road is: v_max = $\sqrt{rg tanθ}$

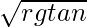
Derivation for all the formulae is given below,

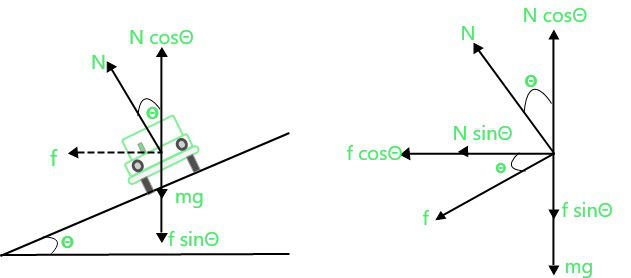
推导

Consider the above figure, where

mg = weight of vehicle

N = Normal reaction

f = Frictional Force

f cosθ = Horizontal component of Frictional Force

f sinθ = Vertical component of Frictional Force

N cosθ = vertical component of Normal Reaction

N sinθ = Horizontal component of Normal Reaction

考虑质量为m的车辆以速度v沿着以角度 θ 倾斜的弯曲坡道行驶。设f为车辆轮胎与路面之间的摩擦力。

作用在车辆上的力是，

1. 重量mg垂直向下
2. 正常反作用力N通过重心向上反作用力并垂直于倾斜的道路/表面。

车辆轮胎与路面之间的摩擦力可分解为，

1. f cosθ – 沿水平方向
2. f sinθ – 沿垂直向下方向

正常反应可以分解为两个组分，

1. N cosθ – 正常反应的垂直分量
2. N sinθ – 正常反应的水平分量

正常反应的分量 N cosθ 由车辆重量mg和摩擦力分量 f sinθ 平衡，

N cosθ = mg + f sinθ

∴ mg = N cosθ – f sinθ ⇢ (1)

水平分量 N sinθ 以及摩擦力的分量 f cosθ 提供了必要的向心力。

N sinθ + f cosθ = mv² / R

Or, mv² / R = N sinθ + f cosθ ⇢ (2)

Dividing Eq (2) by Eq (1),

v² / Rg = N sinθ + f cosθ / N cosθ – f sinθ ⇢ (3)

摩擦力的大小取决于给定路面的车辆速度和车辆的轮胎。设v _max表示车辆的最大速度，在这个速度下产生的摩擦力f _m应该是

f_m  = μ_sN ⇢ (4)

v²_max / Rg =  N sinθ + f cosθ / N cosθ – f sinθ ⇢ (5)

From Eq (4) and Eq (5), we get v_max

∴  v_max = $\sqrt{\frac{Rg[( μs + tanθ)}{(1 - μs tanθ) ]}}$ ⇢ (6)

对于弯曲的水平道路，θ = 0°，因此等式（6）变为，

v_max = √{μ Rg} ⇢ (7)

现在，如果我们比较公式（6）和公式（7），可以发现车辆在有坡度的道路上的最大安全速度大于弯曲的水平道路/水平道路的最大安全速度。

If μ_s = 0, then Eq (6) becomes

v_max = v₀ = $\sqrt{\frac{Rg (0 + tanθ)}{1 - 0 tanθ }}$

v₀ = $\sqrt{Rg tanθ}$ ⇢ (8)

在这个速度下，不需要摩擦力来提供必要的向心力。如果车辆在有坡度的道路上以这种速度行驶，会有一点水和轮胎撕裂。 v ₀称为最佳速度。

From Eq (8), i.e.

v₀ = $\sqrt{Rg tanθ}$  

tanθ = v₀² / Rg

θ = tan^-1[v₀² / Rg] ⇢ (9)

Note: This formula [Eq (9)] for angle of banking does not involves mass of vehicle m. Thus the angle of banking is independent of mass of vehicle.

示例问题

问题 1：求汽车在半径 100m 的曲线上安全行驶的最大速度，轮胎与路面的摩擦系数为 0.2。 （取 g = 9.8 m/s ² ）

解决方案：

Given data: 

• Radius (r) = 100m
• Coefficient of friction (μ) = 0.2
• Gravity (g) = 9.8 m/s²
• Formula: v= √(μrg)

v = √(μrg)

= √(0.2 × 100 × 9.8)

= √196

v = 14 m/s

Thus maximum speed of car is 14 m/s.

问题 2：计算汽车在半径 30m 的弯道上与水平面成 30° 倾斜的道路上安全行驶的最大速度。 （取 g = 9.8m/s ² ）

Given data:

• Radius (r) = 30m
• Angle of banking of road (θ) = 30°
• Gravity (g) = 9.8 m/s²
• Formula: v= √rg tanθ

v= $\sqrt{rg tanθ}$

= $\sqrt{30 × 9.8 × tan 30° }$

= $\sqrt{30 × 9.8 × 0.5774}$

v = 13.03 m/s

Thus the maximum speed of car is 13.03 m/s

问题 3：求自行车及其骑手在平地上绕半径 10m 的弯道以 18km/hr 行驶时与垂线的夹角。 （取 g = 9.8 m/s ² ）

Given data:

• Radius (r) = 10 m
• Max speed of rider (v) = 18 km/hr
• Gravity (g) = 9.8 m/s²
• Formula: tanθ = v² / rg

v = 18 km/hr

= 18 × 1000 / 3600

= 5 m/s

Tangent angle of banking is tanθ = v² / rg

= 5 × 5 / 10 × 9.8

= 5 / 2 × 9.8

tanθ = 0.251 

θ = tan^-1 (0.2551)

θ = 14° 19^‘

Thus the angle which the bicycle and rider make is 14° 19^‘  

问题 4：一辆重 4400kg 的面包车在没有坡度的道路上以 60km/hr 的速度绕过半径 200m 的水平曲线。为防止打滑，摩擦系数的最小值应该是多少？对于这个速度，道路应该以什么角度倾斜。

Given data:

• Weight of van (m) = 4400kg
• Radius (r) = 200m
• Speed of van (v) = 60km/hr
• Gravity (g) = 9.8m/s²

v = 60km/hr

= 60 × 1000 / 3600

= 50 / 3 m/s

To prevent skidding, mv² / r = μmg

μ = v² / rg

= (50 / 3)² / 200 × 9.8

= 25 / 18 × 9.8

μ = 0.1417

Banking  angle  tanθ  is  = v² / rg

= (50 / 3)² / 200 × 9.8

tanθ = 0.1417

θ = tan^-1(0.1417)

θ = 8° 4^‘     

Thus the coefficient of friction is 0.1417 and the angle of banking is 8° 4^‘.

问题5：圆形跑道，半径为500m，坡度为100。假设车辆轮胎与路面的摩擦系数为0.25。计算避免汽车打滑的最大速度和避免轮胎磨损的最佳速度。 （取 g = 9.8 m/s ² ）

Given data:

• Radius (r) = 500m
• Angle (θ) = 10°
• Coefficient of Friction (μ) = 0.25

 since, θ = 10°

 tan10° = 0.1763

On the banked racecourse track, the maximum speed to avoid slipping,

v_max = $\sqrt{(\frac{Rg(μs + tanθ)}{(1−μs tanθ)}}$

= $\sqrt{\frac{500 × 9.8(0.25 + 0.1763)}{(1 - (0.25 × 0.1763))}}$

= √{500 × 9.8 × 0.4263 / 0.9559}

= √2185

v_max = 46.75 m/s

The optimum speed of the vehicle on track is given by v_opt = $\sqrt{rg tanθ}$

= $\sqrt{500 × 9.8 × 0.1763}$

= √863.9

v_opt = 29.39 m/s   

问题 6：求曲率半径为 1500m 的铁路轨道的倾斜角。如果火车的最高速度是15m/s。如果两条轨道之间的距离为 1.8m，则计算外轨道相对于内轨道的高程。

Given data:

• Radius (r) = 1500m
• Velocity (v) = 15m/s
• Distance between two tracks (l) = 1.8m

Tangent angle of Banking ,

tanθ = v² / rg

= 15 × 15 / 1500 × 9.8

= 0.015306

θ = tan^-1(0.0153)

θ  =  0° 52‘

Consider the above figure,

sinθ = h / l

h = l × sinθ  

= 1.8 × sin(0° 52‘)

= 1.8 × 0.0157

h = 0.02826

The  banking angle is 0° 52‘ and the elevation of the outer track over inner track is 0.02826.

问题7：一列米轨列车以60km/Hr的速度沿着曲率半径500m的弯道在某地行驶。求外轨高于内轨的标高，使轨上没有侧压（取g=9.8m/s ² ）

Given data:

• Velocity (v) = 60Km/Hr = 50 / 3 m/s
• Radius (r) = 500m
• l = 1m

In case of Banked railway track,

tanθ = v² / rg

= ( 50 / 3 )² / 500 × 9.8

= 0.05670

θ = tan^-1(0.05670)

θ = 3° 15‘

Elevation of the outer rail above the inner rail,

h = l sinθ

= (1) sin(3° 15‘)

h = 0.0567m