简谐运动中的速度和加速度

简谐运动是一种周期性运动，它在一定时间段后自我重复。在现实生活中几乎随处可见，例如，与弹簧相连的物体在做简谐运动。了解物体的位置、速度和加速度等式至关重要。这些方程帮助我们从 SHM 中推断出有关对象的信息并预测其行为。让我们详细了解如何从 SHM 中提取此信息。

周期性和振荡运动

考虑一个昆虫试图爬上墙壁的例子，这种昆虫爬到一个高度，然后又掉下来。请注意，这种昆虫的运动会在一段时间后重复。这种运动称为周期运动。周期性运动在一定的时间间隔后重复。在图中，显示了一些表示周期性运动的图表。该图有一个重复的模式，这意味着对象的 y 位置周期性地增加然后减少。

周期性运动和振荡运动可能看起来相同，但它们有细微的差别。每个振荡运动都是周期性运动，但反之则不然。周期性运动只是在一定的时间间隔后重复自己，但振荡运动围绕平均位置来回移动。简谐运动是最简单的振荡运动类型。

简谐运动

简谐运动是一种周期性运动，物体围绕其平均位置来回移动。当作用在身体上的力与身体从其平均位置的位移成正比时，就会出现这种运动。在这种情况下，时间段保持不变。时间段用“T”表示，平均位置到极限位置的距离称为振幅，用 A 表示。摆动摆是简单谐波运动的一个例子。

物体在任何特定时间的位移 (x) 的一般方程由下式给出，

$x = A sin(\omega t + \phi)$

这里， $\omega = \frac{2 \pi}{T}$和$\phi$表示相移。

类似地，SHM 中物体的速度方程可以通过对这个方程求微分得到。

$v = A\omega cos(\omega t + \phi)$

然后，加速度方程变为，

$a = -A\omega^2 sin(\omega t + \phi)$

请注意，在上图中，SHM 中的所有三个值位移、速度和加速度都与 SHM 具有相同的时间段，但它们之间的相位为 90°。

示例问题

问题 1：SHM 的方程如下所示。

x(t) = 5cos(2t)

计算最大加速度和速度。

回答：

General equation of SHM is given by, 

$x = A sin(\omega t + \phi)$

In this case, A = 5, $\omega = 2$

Maximum velocity will be, 

$v = A\omega$

⇒ v = (5)(2) 

⇒ v = 10 m/s

Maximum acceleration will be, 

$a = -A\omega^2$

⇒ a = – (5)(2)² 

⇒ a = -20 m/s²

问题 2：SHM 的方程如下所示。

x(t) = 10sin(5t)

计算最大加速度和速度。

回答：

General equation of SHM is given by, 

$x = A sin(\omega t + \phi)$

In this case, A = 10, $\omega = 5$

Maximum velocity will be, 

$v = A\omega$

⇒ v = (10)(5) 

⇒ v = 50 m/s

Maximum acceleration will be, 

$a = -A\omega^2$

⇒ a = – (10)(5)² 

⇒ a = -250 m/s²

问题 3：SHM 的方程如下所示。

x(t) = 100sin(12t)

计算 t = 的时间和速度$\frac{\pi}{24}$ 

回答：

General equation of SHM is given by, 

$x = A sin(\omega t + \phi)$

In this case, A = 100, $\omega = 12$

We know, 

$\omega = \frac{2\pi}{T}$

Plugging the values into the equation, 

$12 = \frac{2\pi}{T}$

⇒ $T = \frac{\pi}{6}$

Equation for velocity will be found by differentiating the given equation, 

x(t) = 100sin(12t) 

⇒$\frac{d}{dt}(x(t)) = \frac{d}{dt}(100sin(12t) )$

⇒ $v(t) = 1200cos(12t)$

At t = $\frac{\pi}{24}$

$v(t) = 1200cos(12t) \\ = v(t) = 1200cos(12(\frac{\pi}{24})) \\ = v(t) = -1200cos(\frac{\pi}{2}) \\ = v(t) = 0$

问题 4：SHM 的方程如下所示。

x(t) = 100sin(2t + 5)

计算 t = 的时间和速度$\frac{\pi}{2}$ 

回答：

General equation of SHM is given by, 

$x = A sin(\omega t + \phi)$

In this case, A = 100, $\omega = 2$

We know, 

$\omega = \frac{2\pi}{T}$

Plugging the values into the equation, 

$2 = \frac{2\pi}{T}$

⇒ $T = \pi$

Equation for velocity will be found by differentiating the given equation, 

x(t) = 100sin(2t + 5) 

⇒$\frac{d}{dt}(x(t)) = \frac{d}{dt}(100sin(2t + 5) )$

⇒ $v(t) = 200cos(2t + 5)$

At t = $\frac{\pi}{2}$

$v(t) = 200cos(2t + 5) \\ = v(t) = 1200cos(2(\frac{\pi}{4}) + 5) \\ = v(t) = -1200cos(\frac{\pi}{2}) \\ = v(t) = 0$

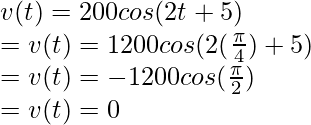
问题 5：SHM 的方程如下所示。

x(t) = 2sin(t – 5)

计算 t = 的时间周期、加速度和速度$5 + \frac{\pi}{4}$ 

回答：

General equation of SHM is given by, 

$x = A sin(\omega t + \phi)$

In this case, A = 2, $\omega = 1$

We know, 

$\omega = \frac{2\pi}{T}$

Plugging the values into the equation, 

$1 = \frac{2\pi}{T}$

⇒ $T = 2\pi$

Equations for velocity and accelerations will be found by differentiating the given equation, 

x(t) = 2sin(t – 5) 

⇒$\frac{d}{dt}(x(t)) = \frac{d}{dt}(2sin(t - 5) )$

⇒ $v(t) = 2cos(t - 5)$

At t = $\frac{\pi}{4} + 5$

$v(t) = 2cos(t - 5) \\ = v(t) = 2cos(\frac{\pi}{4} + 5 - 5) \\ = v(t) = 2cos(\frac{\pi}{4}) \\ = v(t) = \sqrt{2}$

$v(t) = 2cos(t - 5)$

⇒$\frac{d}{dt}(v(t)) = \frac{d}{dt}(2cos(t - 5) )$

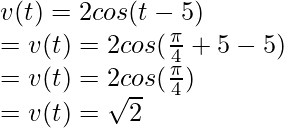
⇒ a(t) = -2sin(t – 5)

At t = $\frac{\pi}{4} + 5$

$a(t) = -2sin(t - 5) \\ a(t) = -2sin(5 + \frac{\pi}{4} - 5) \\ a(t) = -2sin(\frac{\pi}{4}) \\ a(t) = -\sqrt{2}$