大圆距离公式
可以在球体表面上绘制的最大圆是大圆。大圆是包含球体直径的球体区域,也是球体表面上任意两个位置之间的最短距离。它也被称为罗马尼亚圈。
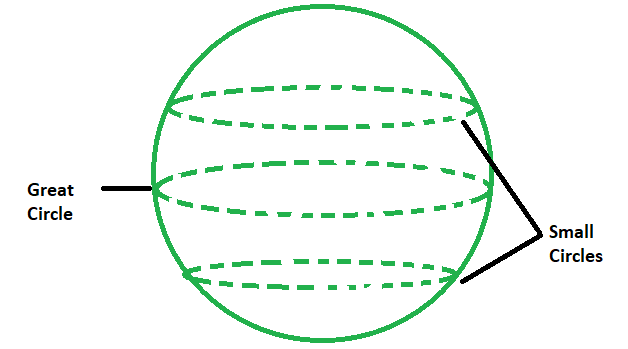
下图描绘了一个球体和可以在其表面绘制的不同半径和大小的圆。
大圆的应用
大圆公式的应用被用于飞机或船舶的导航,这是应用它的少数例子之一。因为地球是球形的,所以大圆公式通过确定球体内的最短距离来帮助导航。
如下所示,大圆是与主球体本身共享中心的圆。球体和大圆的直径重合。

大圆公式
大圆公式如下:
d = rcos-1[cos a cos b cos(x-y) + sin a sin b]
where,
r depicts the earth’s radius,
a and b depict the latitude
while the longitudes are depicted by x and y.
示例问题
问题1.假设一个球体的半径是4.7公里,纬度是(45°,32°),经度是(24°,17°),求大圆的距离。
解决方案:
The great circle formula is given by: d = rcos-1[cos a cos b cos(x-y) + sin a sin b].
Given: r = 4.7 km or 4700 m, a, b= 45°, 32° and x, y = 24°,17°.
Substituting the values in the above formula, we have:
d = 4700 cos-1(0.52 × 0.83 × 0.75) + (0.85 × 0.32)
= 4700 × 0.99
⇒ d = 4653 m
问题 2. 假设一个球体的半径是 5 公里,纬度是(25°,34°),经度是(48°,67°),求大圆的距离。
解决方案:
The great circle formula is given by: d = rcos-1[cos a cos b cos(x-y) + sin a sin b].
Given: r = 5 km, a, b= 25°, 34° and x, y = 48°,67°.
Substituting the values in the above formula, we have:
d = 5 cos-1(0.913 × 0.83× 0.945) + (0.406 × 0.556)
= 5 × 2.793
⇒ d = 94.928 km or 94928 m
问题 3. 假设一个球体的半径是 10 公里,纬度是(55°,86°),经度是(28°,70°),求大圆的距离。
解决方案:
The great circle formula is given by: d = rcos-1[cos a cos b cos(x-y) + sin a sin b].
Given: r = 10 km, a, b= 55°, 86° and x, y = 28°,70°.
Substituting the values in the above formula, we have:
d = 10 cos-1(0.573 × 0.0707× 0.743) + (0.819 × 0.997)
= 10 × 0.5602
⇒ d = 5.62 km
问题 4. 假设一个球体的半径为 7 公里,纬度为(55°,86°),经度为(28°,70°),求大圆的距离。
解决方案:
The great circle formula is given by: d = rcos-1[cos a cos b cos(x-y) + sin a sin b].
Given: r = 7 km, a, b= 55°, 86° and x, y = 28°,70°.
Substituting the values in the above formula, we have:
d = 7 cos-1(0.573 × 0.0707× 0.743) + (0.819 × 0.997)
= 7 × 0.5602
⇒ d = 3.93 km
问题 5. 假设一个球体的半径为 4 公里,纬度为(25°,34°),经度为(48°,67°),求大圆的距离。
解决方案:
The great circle formula is given by: d = rcos-1[cos a cos b cos(x-y) + sin a sin b].
Given: r = 4 km, a, b= 25°, 34° and x, y = 48°,67°.
Substituting the values in the above formula, we have:
d = 4 cos-1(0.913 × 0.83× 0.945) + (0.406 × 0.556)
= 4 × 2.793
⇒ d = 11.17 km
问题 6. 假设一个球体的半径是 16 公里,纬度是(45°,32°),经度是(24°,17°),求大圆的距离。
解决方案:
The great circle formula is given by: d = rcos-1[cos a cos b cos(x-y) + sin a sin b].
Given: r = 16 km, a, b= 45°, 32° and x, y = 24°,17°.
Substituting the values in the above formula, we have:
d = 16 cos-1(0.52 × 0.83 × 0.75) + (0.85 × 0.32)
= 16 × 0.99
⇒ d = 15.84 km
问题 7. 假设一个球体的半径是 18 公里,纬度是(45°,32°),经度是(24°,17°),求大圆的距离。
解决方案:
The great circle formula is given by: d = rcos-1[cos a cos b cos(x-y) + sin a sin b].
Given: r = 18 km, a, b= 45°, 32° and x, y = 24°,17°.
Substituting the values in the above formula, we have:
d = 18 cos-1(0.52 × 0.83 × 0.75) + (0.85 × 0.32)
= 18 × 0.99
⇒ d = 17.82 km