距离公式和截面公式–三维几何

在任何工程师或科学家的工具包中，功能最强大的工具之一就是3D几何体。 3D几何图形可用于模拟现实世界中的量，例如速度，流体流动，电信号和许多其他物理量。在本文中，我们将讨论两个重要的公式距离和截面以及一些重要的示例。一个人可以用3个坐标(x，y和z)来理解任何3D空间。以下是3D空间的简单表示。

距离公式及其在3D几何中的应用

距离公式表示XYZ空间中任意两点之间的距离。

公式：

For two points P1(x₁, y_1, z₁) and P2(x₂, y_2, z₂)

Distance (d) = $\sqrt{(x_2-x_1)^2+(y_2-y_1)^2+(z_2-z_1)^2}$

此距离公式用于计算任何3D空间中两个点之间的距离。当我们知道平面中两个点的坐标(以有序对(x，y，z)的形式)时，我们可以通过替换距离公式轻松获得两个点之间的距离。

两点之间的距离：公式和求解的示例

假设在3D空间中有两个点P(x ₁ ，y _1， z ₁ )和Q(x ₂ ，y _2， z _2)。为了找到它们之间的距离，公式是

Distance (d) = $\sqrt{(x_2-x_1)^2+(y_2-y_1)^2+(z_2-z_1)^2}$

示例1：找到点P(1，–3，4)和Q(– 4，1，2，2)之间的距离?

解决方案：

Using the formula to calculate the distance between point P and Q, 

Distance (d) = $\sqrt{(x_2-x_1)^2+(y_2-y_1)^2+(z_2-z_1)^2}$

d = $\sqrt{(-4-1)^2+(1+3)^2+(2-4)^2} \\ =\sqrt{25+16+4} \\ =\sqrt{45} \\ =3 \sqrt{5}$

示例2：表明点P(–2、3、5)，Q(1、2、3)和R(7、0，–1)共线吗?

解决方案：

We know that points are said to be collinear if they lie on a line.

$PQ = \sqrt{(1+2)^2+(2-3)^2+(3-5)^2} = \sqrt{9+1+4} = \sqrt{14}\\ QR = \sqrt{(7-1)^2+(0-2)^2+(-1-3)^2} = \sqrt{36+4+16} =2 \sqrt{14}\\ PR = \sqrt{(7+2)^2+(0-3)^2+(-1-5)^2} = \sqrt{81+9+36} =3 \sqrt{14}$ 

As PQ + QR = PR  

Hence P, Q and R are collinear.

点到直线的距离：含义，公式和示例

点到线的距离是点到线的垂直距离。假设我们必须找到点P(x ₀ ，y _0， z ₀ )与直线l的距离，则公式为：

Distance (d) = $\frac{ |\overline{P0P1}* \overline{s}| }{ |\overline{s}| }$

Where ‘s’ is the directing vector of line l.

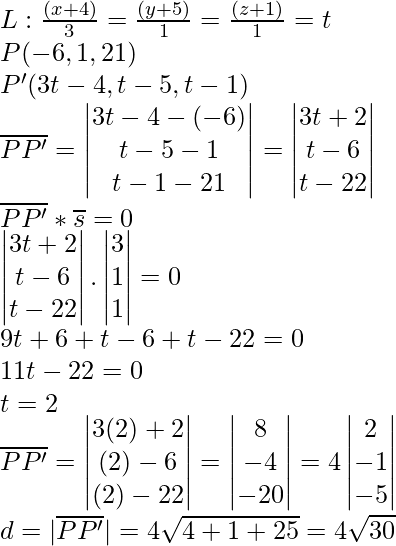
示例：找到从点P(-6，1，21)到直线的距离$\frac{x+4}{3}=\frac{y+5}{1}=\frac{z+1}{1}$ ?

解决方案：

$L: \frac{(x+4)}3 = \frac{(y+5)}1 = \frac{(z+1)}1 = t \\ P(-6,1,21)\\ P'(3t-4,t-5,t-1) \\ \overline{PP'} = \begin{vmatrix}{3t-4-(-6)} \\ {t-5-1} \\ {t-1-21}\end{vmatrix} = \begin{vmatrix}{3t+2} \\ {t-6} \\ {t-22}\end{vmatrix} \\ \overline{PP'}* \overline{s} = 0 \\ \begin{vmatrix}{3t+2} \\ {t-6} \\ {t-22}\end{vmatrix} . \begin{vmatrix}{3} \\ {1} \\ {1}\end{vmatrix} = 0\\ 9t+6+t-6+t-22=0 \\ 11t-22=0 \\ t=2 \\ \overline{PP'} = \begin{vmatrix}{3(2)+2} \\ {(2)-6} \\ {(2)-22}\end{vmatrix} = \begin{vmatrix}{8} \\ {-4} \\ {-20}\end{vmatrix} = 4\begin{vmatrix}{2} \\ {-1} \\ {-5}\end{vmatrix} \\ d = |\overline{PP'}| = 4 \sqrt{4+1+25} = 4 \sqrt{30}$

点到平面的距离：公式，方程式和示例

从点到平面的距离是从点到平面的垂直距离。如果Qx + Ry + Sz + T = 0是一个平面方程，则可以使用以下公式找到从点P(Px，Py，Pz)到平面的距离：

Distance (d) = 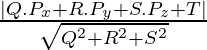$\frac{ |Q.P_x+R.P_y+S.P_z+T| }{ \sqrt{Q^2+R^2+S^2} }$

示例：要查找平面2x + 4y – 4z – 6 = 0与点P(0，3，6)之间的距离?

解决方案：

Using the formula: 

Distance (d) = 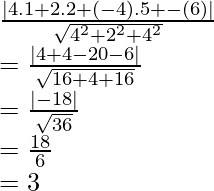$\frac{ |4.1+2.2+(-4).5+-(6)| }{ \sqrt{4^2+2^2+4^2} }\\ =\frac{ |4+4-20-6| }{ \sqrt{16+4+16} }\\ =\frac{ |-18| }{ \sqrt{36} }\\ =\frac{ 18 }{6}\\ =3$

平行线之间的距离：公式和示例

两条平行线之间的距离是一条线上的任何点到另一条线的垂直距离。假设有两条平行线y = mx + c ₁和y = mx + c ₂ ，则公式为：

Distance (d) =$\frac{|c_2-c_1|}{ \sqrt{1+m^2} }$

如果两条平行线的方程式为：

ax + by + d1 = 0和ax + by + d2 = 0，则公式为

Distance (d) = $\frac{|d_2-d_1|}{ \sqrt{a^2+b^2} }$

Where a and b are the coefficients of variables x and y in the line.

示例1：找出线y = 2x + 10和y = 2x + 12之间的距离? (注意：两条线彼此平行)

解决方案：

The lines y = 2x + 10 and y = 2x + 12 are in form y = mx + c.

Where c₁​ = 10, c₂ ​= 12, m = 2 

Using formula, the distance (d) =  $\frac{|12-10|}{ \sqrt{1+2^2} } = \frac{2}{\sqrt{5}}$

示例2：找到两条平行线4x + 3y + 6 = 0和4x + 3y – 3 = 0之间的距离?

解决方案：

The lines given are 4x + 3y + 6 = 0 and 4x + 3y – 3 = 0. Both lines are in form ax + by + d = 0.

Hence, d₁ ​= 6, d₂ ​= −3, a = 4, b = 3 

Using formula for this case, distance (d) will be calculated as:

$d = \frac{|d2-d1|}{ \sqrt{a^2+b^2} } \\ d = \frac{|-3-6|}{ \sqrt{4^2+3^2} } \\ d = \frac{|-9|}{ \sqrt{16+9} } = \frac{9}{ \sqrt{25} } = \frac{9}{5}$

公式部分：定义，向量公式，大小写和示例

截面公式是可以在2D和3D空间中实现的概念。在三维系统中，我们必须选择一个坐标系。假设给定了两个点P(x1，y1，z1)和Q(x2，y2，z2)，并让点R(x，y，z)在内部以给定的m：n比率划分PQ。因此，可以使用以下公式计算在内部以m：n划分连接两个点P(x1，y1，z1)和Q(x2，y2，z2)的线段的点R的坐标，

$\left( \frac{mx_2 + nx_1}{m+n},\frac{my_2 + ny_1}{m+n},\frac{mz_2 + nz_1}{m+n} \right)$

如果点R在外部以m：n的比例划分PQ，则通过将n替换为–n来获得其坐标，其公式为：

$\left( \frac{mx_2 - nx_1}{m-n},\frac{my_2 - ny_1}{m-n},\frac{mz_2 - nz_1}{m-n} \right)$

情况1：中点的坐标：如果R是PQ的中点，则m：n = 1：1：

x = $\frac{x_1 + x_2}{2}$, y = $\frac{y_1 + y_2}{2}$ and z = $\frac{z_1 + z_2}{2}$

这些是连接P(x1，y1，z1)和Q(x2，y2，z2)的线段中点的坐标。

情况2：通过将k = $\frac{m}{n}$如下所示，

$\left( \frac{kx_2 + x_1}{k+1},\frac{ky_2 + y_1}{k+1},\frac{kz_2 + z_1}{k+1} \right)$

示例1：找到将点(1，–2，3)和(3，4，–5)相连的线段以2：3的比率划分的点的坐标(i)内部，(ii)外部?

解决方案：

(i) Let P (x, y, z) be the point that divides the line segment joining A(1, – 2, 3)

and B (3, 4, –5) internally in the ratio 2 : 3. Therefore

$\frac{2(3)+3(1)}{2+3} = \frac{9}{5} \\ \frac{2(4)+3(-2)}{2+3} = \frac{2}{5} \\ \frac{2(-5)+3(3)}{2+3} = \frac{-1}{5}$

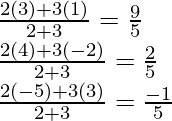
Thus, the required point is $(\frac{9}{5},\frac{2}{5},\frac{-1}{5})$

(ii) Let P (x, y, z) be the point which divides segment joining A (1, –2, 3) and

B (3, 4, –5) externally in the ratio 2 : 3. Then,

$\frac{2(3)+(-3)(1)}{2+(-3)} = -3 \\ \frac{2(4)+(-3)(-2)}{2+(-3)} = -14 \\ \frac{2(-5)+(-3)(3)}{2+(-3)} = 19$

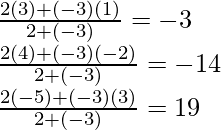
Therefore, the required point is (–3, –14, 19). 

示例2：使用截面公式，证明三个点(– 4，4，10)，(2，4，6)和(14，0，–2)是共线的吗?

解决方案：

Let A (– 4, 6, 10), B (2, 4, 6), and C(14, 0, – 2) be the given points. Let the

point P divides AB in the ratio k: 1. Then coordinates of the point P are

$(\frac{2k-4}{k+1},\frac{4k+6}{k+1},\frac{6k+10}{k+1})$

Let us examine whether, for some value of k, the point P coincides with point C.

On putting $\frac{2k-4}{k+1}=14\\ k=\frac{-3}{2}$, When k=$\frac{-3}{2}$then,

$\frac{4k+6}{k+1} =\frac{4 (-\frac{3}{2})+6}{(-\frac{3}{2})+1}=0 \\ and\\ \frac{6k+10}{k+1} =\frac{6 (-\frac{3}{2})+10}{(-\frac{3}{2})+1}=-2 \\$

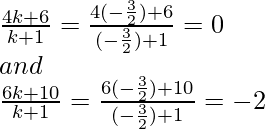
Therefore, C (14, 0, –2) is a point that divides AB externally in the ratio 3: 2 and is

same as P. Hence A, B, C are collinear.