谐波平均公式

统计处理数据分析并以有组织的方式呈现数据/值。使用集中趋势的概念来表示系列统计中的数据。集中趋势的度量是描述围绕中心值的一组数据的值。它是一组数据的中心。

集中趋势的三个指标，

1. 意思是
2. 中位数
3. 模式

现在让我们知道一种重要的平均值，称为谐波平均值。

谐波平均值

谐波平均值定义为所有数据值倒数的平均值的倒数。它对较大的值赋予较小的权重，对较小的值赋予较大的权重。这种调和平均数主要用于金融领域。

假设 x ₁ , x ₂ , x ₃ , x ₄ , ... x _n是给定数据，则给定数据的调和平均值可以通过以下公式计算：

Harmonic Mean (H.M) = $\frac{n}{(1/x_1)+(1/x_2)+(1/x_3)+(1/x_4)+...+(1/x_n)}$

证明

Harmonic mean is the inverse of arithmetic mean of reciprocal data terms.

So the arithmetic mean for the data x₁, x₂, x₃, …, x_n is,

Arithmetic mean = $\frac{x_1+x_2+x_3+...+x_n}{n}$

In harmonic mean we consider reciprocal of data values.

So the arithmetic mean of reciprocal data 1/x₁, 1/x₂, 1/x₃, …, 1/x_n  is,

Arithmetic mean for reciprocal data = $\frac{(1/x_1)+(1/x_2)+(1/x_3)+...+(1/x_n)}{n}$ ->(1)

It is known that Harmonic mean is inverse of arithmetic mean of reciprocal data values from-(1)

Harmonic Mean = Arithmetic Mean of Reciprocal data^-1

=$(\frac{(1/x_1)+(1/x_2)+(1/x_3)+...+(1/x_n)}{n})^{-1}$

= $\frac{n}{(1/x_1)+(1/x_2)+(1/x_3)+...+(1/x_n)}$

加权谐波平均值

它类似于谐波平均值，但除此之外，这是权重。如果权重等于 1，则它与谐波平均值相同。加权谐波平均值是针对给定的一组权重 w ₁ ,w ₂ ,w ₃ ,w ₄ ,...,w _n和数据 x ₁ , x ₂ , x ₃ , x ₄ , ..., x _n计算的，

加权调和平均值 = 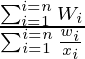$\frac{\sum_{i=1}^{i=n}W_i}{\sum_{i=1}^{i=n}\frac{w_i}{x_i}}$

让我们看看手段类型之间的关系，

算术平均值、调和平均值和几何平均值之间的关系

For the data x₁, x₂, x₃, x₄, …, x_n,

Arithmetic Mean = (x₁ + x₂ + x₃ + x₄ +….+ x_n)/n

Harmonic Mean = n/((1/x₁) + (1/x₂) + (1/x₃) + (1/x₄) + … + (1/x_n))

Geometric mean = n√(x₁ + x₂ + x₃ + x₄ + … + x_n)

From these formulas we can write it as,

Geometric mean = √(Arithmetic Mean × Harmonic Mean)

Geometric mean² = Arithmetic Mean × Harmonic Mean

From the Above formula,

Harmonic Mean = Geometric mean²/Arithmetic Mean

让我们看几个找到调和平均值的例子，

示例问题

问题 1：数据 10、20、5、15、10 的调和平均值是多少。

解决方案：

Given data

10, 20, 5, 15, 10

n = 5 (As total size is 5)

Harmonic mean= $\frac{n}{(1/x_1)+(1/x_2)+(1/x_3)+(1/x_4)+...+(1/x_n)}$

= $\frac{5}{(1/10)+(1/20)+(1/5)+(1/15)+(1/10)}$

= 5/(0.1 + 0.05 + 0.2 + 0.06 + 0.1)

= 5/0.51

= 9.8

Hence Harmonic mean for the given data is 9.8

问题 2：数据 100、50、75、25 的调和平均值是多少。

解决方案：

Given data

100, 50, 75, 25

n = 4 (As total size is 4)

Harmonic mean= $/frac{n}/{(1/x_1)+(1/x_2)+(1/x_3)+(1/x_4)+...+(1/x_n)}$

= $\frac{4}{(1/100)+(1/50)+(1/75)+(1/25)}$

= 4/(0.01+0.02+0.013+0.04)

= 4/0.083

= 48.2

Hence Harmonic mean for the given data is 48.2

问题 3：数据 1、5、3 的调和平均值是多少。

解决方案：

Given data

1, 5, 3

n = 3 (As total size is 3)

Harmonic mean= $\frac{n}{(1/x_1)+(1/x_2)+(1/x_3)+(1/x_4)+...+(1/x_n)}$

= $\frac{3}{(1/1)+(1/5)+(1/3)}$

= 3/(1 + 0.2 + 0.33)

= 3/1.53

= 1.96

Hence Harmonic mean for the given data is 1.96

问题 4：如果算术平均值为 10，几何平均值为 7，那么调和平均值是多少。

解决方案：

Given, 

Arithmetic Mean (A.M) = 10

Geometric Mean (G.M) = 7

Harmonic Mean = G.M²/A.M

= 7²/10

= 49/10

= 4.9

Hence Harmonic mean from given Arithmetic and geometric mean is 4.9

问题 5：如果算术平均值为 20，谐波平均值为 15，几何平均值是多少。

解决方案：

Given

Arithmetic Mean (A.M) = 20

Harmonic Mean (H.M) = 15

Geometric Mean = √(Arithmetic Mean × Harmonic Mean)

= √(20 × 15)

= √300

= 17.32

Hence Geometric mean from given Arithmetic and Harmonic mean is 17.32

问题 6：给定数据的加权调和平均值是多少。

Weight(w)	Data(x)
1	20
2	30
3	10
2	15

解决方案：

Find Summation of Weights i.e., ∑w_i, 1/x and w/x and ∑w/x

Harmonic Mean_w = ∑w/∑w/x

= 8/0.53

= 15.09

Weighted Harmonic mean for the given data is 15.09

问题 7：给定数据的加权调和平均值是多少。

Weights(w)	x	1/x	w/x
1	20	0.05	0.05
2	30	0.03	0.06
3	10	0.1	0.3
2	15	0.06	0.12
∑w=8			∑w/x=0.53

解决方案：

Find Summation of Weights i.e., ∑w_i, 1/x and w/x and ∑w/x

x	w	1/x	w/x
10	2	0.1	0.2
15	3	0.066	0.198
20	4	0.05	0.2
25	5	0.04	0.2
30	1	0.033	0.033
	∑w = 15		∑w/x = 0.831

Harmonic Mean_w = ∑w/∑w/x

= 15/0.831

= 18.05

Weighted Harmonic mean for the given data is 18.05