📌  相关文章
📜  James A. Garfield 的勾股定理证明

📅  最后修改于: 2022-05-13 01:54:14.036000             🧑  作者: Mango

James A. Garfield 的勾股定理证明

毕达哥拉斯定理是直角三角形的定理，也称为毕达哥拉斯定理。它用于显示直角三角形三角形边上的连接。根据这个定理，任何两个小边的平方和等于最大边的平方。直角三角形的小边垂直且底边，而最大的边称为斜边。

这个定理的发现与古希腊哲学家毕达哥拉斯有关，因此被称为毕达哥拉斯定理。

毕达哥拉斯定理的表达式：

（底） 2 +（垂直） 2 =（斜边） 2

A 2 + B 2 = C 2

詹姆斯·加菲尔德总统对勾股定理的证明

This proof of the Pythagorean Theorem was given by President James A. Garfield's, who was the 20 th president and was elected in the year 1881, he really likes maths and gave this proof of the Pythagorean theorem.

让我们证明这个定理

第一步：画一个直角三角形，边有A、B、C。

步骤2：画另一个相同尺寸的三角形，但第一个三角形的A边应该与第二个三角形的B边形成一条直线。

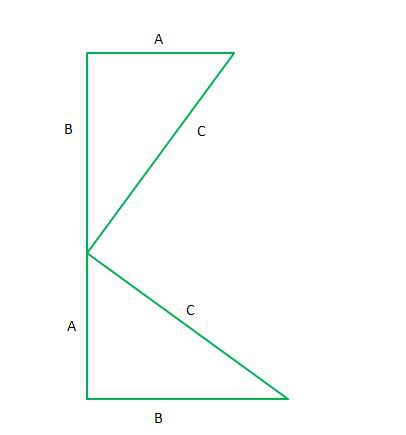
步骤3：现在标记两个三角形的角度，让我们取一个角度为θ，所以另一个角度将是90 - θ，因为它是一个直角三角形，第三个角度是90度，所有角度的总和一个三角形是180度。

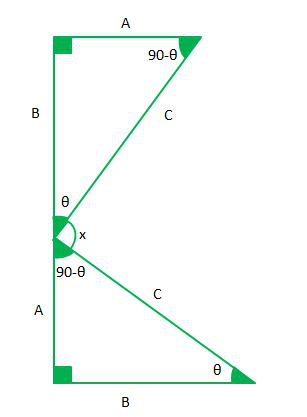
第 4 步：现在我们知道直线上的角度和是 180 度，所以使用它我们将找到角度 x。

θ + x + 90 – θ = 180°

x + 90 = 180°

x = 90°

因此，x 是 90° 角。

第5步：现在画一条直线，制作第三个三角形，两侧分别为C和C，并将三角形标记为1、2和3。

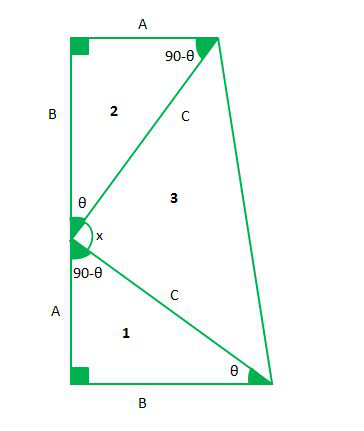
第6步：现在整个图形像一个梯形，所以我们将找到它的面积。

梯形面积 = 1/2 × (平行边之和) × (高)

在上图中，平行边是A和B，梯形的高度是A+B。

面积 = 1/2(A+B)×(A+B)

第7步：同样的面积也可以通过将3个直角三角形的面积相加来计算。

  • 直角三角形的面积 = 1/2（底）×（高）
  • 整个图形的面积=第一个三角形的面积+第二个三角形的面积+第三个三角形的面积
  • 整个图形的面积 = 1/2(A)×(B) + 1/2(A)×(B) + 1/2(C)×(C)
  • 整个图形的面积 = A×B + C 2 /2

第 8 步：两个区域应该相等，所以将它们彼此相等。

1/2(A+B)×(A+B) = A×B + C 2 /2

(A+B) 2 = 2(A×B) + C 2

A 2 + B 2 + 2(A×B) = 2(A×B) + C 2

A 2 + B 2 = C 2

因此我们证明了勾股定理

示例问题

问题 1：求一个高 31 厘米、底边 480 厘米的直角三角形的斜边的长度？

解决方案：

问题2：高10厘米，平行边长8厘米和12厘米的梯形的面积是多少。

解决方案：

问题 3：斜边为 10 厘米，底边为 6 厘米的直角三角形的面积是多少。

解决方案：

问题 4：求一个直角三角形的斜边长度，其高为 69 厘米，底为 260 厘米？

解决方案：

问题5：一个梯形高20厘米，平行边长5厘米和18厘米的面积是多少。

解决方案：