证明 2 cos x – 2 cos ³ x = sin x sin 2x

三角学名称本身表示它是一门处理三角形几何的学科，当需要找到何时给定一些边并且我们需要边之间的关系或边之间的角度时，它非常有用。在三角学中，我们有不同的比率，即 sin A、cos A、tan A、cot A、sec A、cosec A 借助这些比率，可以获得三角形边之间的关系和边之间的角度。

三角函数

三角函数定义了边和角之间的关系，例子有 sin A, cos A, tan A, cot A, sec A, cosec A。不同三角函数之间的关系是三角恒等式。恒等式对于检验三角方程中的不等式非常有用。

例子是，

• Tan A= sin A/cos A
• 罪 A = 1/cosec A
• cos A= 1/秒 A
• 棕褐色 A= 1/婴儿床 A

证明 2 cos x – 2 cos ³ x = sin x sin 2x

为了解决上述问题陈述需要基本恒等式，让我们看一下在这种情况下所需的 6 个三角函数的一些基本恒等式，

证明中使用的先决条件身份

• sin 2x = 2 × sin x × cos x
• 罪² x + cos ² x = 1
• 1 – cos ² x = 罪² x
• sin x = 1/cosec x
• cos x – cos y = $-2\frac{sin(x-y)}{2}\frac{sin(x+y)}{2}$

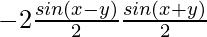
给定三角方程

2 cos x – 2 cos ³ x= sin x sin 2x

LHS = 2 cos x – 2 cos ³ x

RHS = 罪 x 罪 2x

从 LHS 导出证明

Given LHS

2 cos x – 2 cos³x

Step-1

Taking the 2 cos x which is common in both the terms

= 2cos x (1-cos² x)

Step-2

Substituting 1-cos²x which is equal to sin²x

= 2cos x(sin² x)

= (sin x) × (2 × sinx × cos x)

Step-3

Substituting 2 × sin x × cos x which is sin 2x

= sin x × sin 2x

从步骤 3 可以得出结论，LHS =sin x × sin 2x 等于 RHS，因此，

罪 x × 罪 2x=罪 x × 罪 2x

左轴 = 右轴

因此证明。

从 RHS 获得证明

Given RHS

 sin x sin 2x

Step-1

Substituting the formula of sin 2x in the given RHS

= sin x × (2 sinx cos x)

= 2 × sin²x × cosx

Step-2

Substituting the formula of sin²x which is equal to 1-cos²x

= 2 × (1-cos²x) × cos x

= 2cos x – cos³x

从步骤 2 可以得出结论，RHS = 2cos x-cos ³ x 等于 LHS，因此，

2cos x-cos ³ x = 2cos x-cos ³ x

左轴 = 右轴

因此证明。

示例问题

问题一：求解三角恒等式：4cos ² x-4cos ⁴ x+cos ² 2x

解决方案：

• Taking 2cosx common in the first two terms we get

= 2cosx (2cosx-2cos³x) + cos²2x

• From the above derivation 2cosx – 2cos³x = sinxsin2x

= 2cosx(sinxsin2x) + cos²2x

• From the standard identity 2

= sin²2x + cos²2x

= 1

问题 2：求解三角恒等式：(1/ 8 × cosecx cosec2x cosx – 8 cosecx cosec2x cos ³ x)

解决方案：

• Taking the 4 cosecx cosec2x common in the denominator

= 1/4 cosecx cosec2x (2cosx -2cos³x)

• Using identity 4

= (sinx sin 2x)/4 × (2cosx -2cos³x)

• By the derived identity the equation becomes

= (sinx sin 2x) / (4 × sinx sin2x)

= 1/4 = 0.25

问题 3：求解三角恒等式：(cos 3x – cosx) / (4cos ³ x – 4cosx)

解决方案：

• Solving the numerator using the identity 5

= $-2\frac{\frac{sin(3x-x)}{2}\frac{sin(3x+x)}{2}}{4cos3x-4cosx}$

= -2sinx sin 2x / 4cos³x – 4cosx

• Solving the denominator by multiplying -1 and dividing by -1 to get the denominator to our derived identity

= 2 sin x sin 2x/2(2cos³x-2cosx)

= sin x sin 2x/(2cos³x-2cosx)

= sin x sin 2x/sin x sin 2x

= 1