第 12 课 NCERT 解决方案 - 数学第一部分 - 第 5 章连续性和可微性 - 练习 5.6
如果 x 和 y 由练习 1 到 10 中给出的方程参数连接,不消除参数,求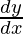
问题 1. x = 2at 2 , y = at 4
解决方案:
Here, x = 2at2, y = at4
![]()
= 2a ![]()
= 2a (2t)
= 4at
And, now
![]()
= a ![]()
= a (4t3)
= 4at3
Now, as
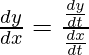
= ![]()
![]() = t2
= t2
问题 2. x = a cos (θ) , y = b cos (θ)
解决方案:
Here, x = a cos(θ), y = b cos(θ)
![]()
= a ![]()
= a (-sin(θ))
= – a sin(θ)
And, now
![]()
= b ![]()
= b (-sin(θ))
= – b sin(θ)
Now, as
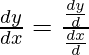
= ![]()
![]()
问题 3. x = sin( t) , y = cos (2t)
解决方案:
Here, x = sin(t), y = cos(2t)
![]()
= cos(t)
And, now
![]()
= -sin(2t) ![]()
= – 2sin(2t)
Now, as
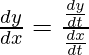
= ![]()
= ![]() (Using the identity: sin(2θ) = 2 sinθ cosθ)
(Using the identity: sin(2θ) = 2 sinθ cosθ)
![]() = – 4 sin(t)
= – 4 sin(t)
问题 4. x = 4t, y = 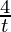
解决方案:
Here, x = 4t, y = 4/t
![]()
= 4 ![]()
= 4
And, now
![]()
= 4 ![]()
= 4 ![]()
= 4 ![]()
= 4![]()
= ![]()
Now, as
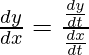
= ![]()
![]()
问题 5. x = cos (θ) – cos (2θ) , y = sin (θ) – sin (2θ)
解决方案:
Here, x = cos(θ) – cos(2θ), y = sin(θ) – sin(2θ)
![]()
= ![]()
= – sin(θ) – (-sin(2θ)) ![]()
= – sin(θ) + 2sin(2θ)
And, now
![]()
= ![]()
= cos(θ) – (cos(2θ)) ![]()
= cos(θ) – (2 cos(2θ)
Now, as
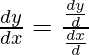
![]()
问题 6. x = a (θ – sin (θ) ), y = a (1 + cos (θ) )
解决方案:
Here, x = a (θ – sin(θ)), y = a (1 + cos(θ))
![]()
= a (![]() )
)
= a (1 – cos(θ))
And, now
![]()
= a (![]() )
)
= a (0 + (- sin (θ)))
= – a sin (θ)
Now, as

=![]()
= ![]() (Using identity: sin(2θ) = 2 sinθ cosθ and 1- cos(2θ) = 2 sin2θ)
(Using identity: sin(2θ) = 2 sinθ cosθ and 1- cos(2θ) = 2 sin2θ)
![]() = – cot(θ/2)
= – cot(θ/2)
问题 7. x = 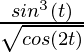 , y =
, y = 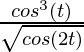
解决方案:
Here, x = 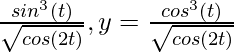
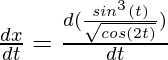
= 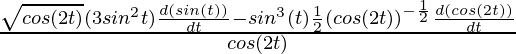
= 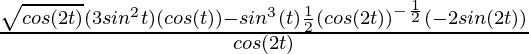
=
= 
= 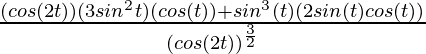
= 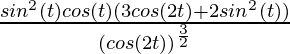
And, now
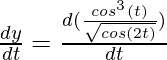
=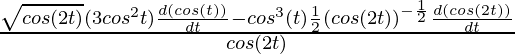
= 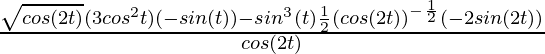
= 
= 
= 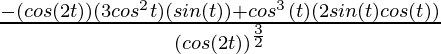
= 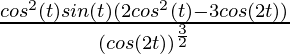
Now, as

= 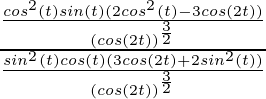
= ![]()
= ![]()
= ![]()
![]()
![]() = – cot 3(t)
= – cot 3(t)
问题 8. x = a (cos (t) + log tan 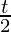 ), y = 一个罪( t)
), y = 一个罪( t)
解决方案:
Here, x = a (cos(t) + log tan ![]() ), y = a sin(t)
), y = a sin(t)
![]()
= a (![]() )
)
= a (-sin(t) +  )
)
= a (-sin(t) + ![]() )
)
= a (-sin(t) + 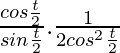 )
)
= a (-sin(t) + ![]() )
)
= a (-sin(t) + ![]() ) (Using identity: 2 sinθ cosθ = sin(2θ))
) (Using identity: 2 sinθ cosθ = sin(2θ))
= a (![]() – sin(t))
– sin(t))
= a (![]() )
)
= a (![]() )
)
=![]()
And, now
![]()
= a ![]()
= a cos(t)
Now, as
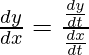
= 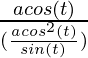
![]() = tan(t)
= tan(t)
问题 9. x = a sec (θ) , y = b tan (θ)
解决方案:
Here, x = a sec(θ), y = b tan(θ)
![]()
= a (![]() )
)
= a (sec(θ) tan(θ))
= a sec(θ) tan(θ)
And, now
![]()
= b (![]() )
)
= b (sec2(θ))
Now, as
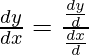
![]()
![]()
![]()
![]()
问题 10. x = a (cos (θ) + θ sin (θ) ), y = a (sin (θ) – θ cos (θ) )
解决方案:
Here, x = a (cos(θ) + θ sin(θ)), y = a (sin(θ) – θ cos(θ))
![]()
= a (![]() )
)
= a (- sin(θ) + (θ.![]() ) + sin(θ).
) + sin(θ).![]() )
)
= a (- sin(θ) + (θ.(cos(θ) + sin(θ).1))
= a (- sin(θ) + θ cos(θ) + sin(θ))
= aθ cos(θ)
And, now
![]()
= a (![]() )
)
= a (cos (θ) – (θ.![]() ) + cos(θ).
) + cos(θ).![]() )
)
= a (cos(θ) – (θ.(-sin (θ) + cos(θ).1))
= a (cos(θ) + θ sin(θ) – cos(θ))
= aθ sin(θ)
Now, as
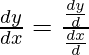
= ![]()
![]() = tan(θ)
= tan(θ)
问题 11. 如果 x = 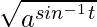 , y =
, y = 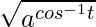 , 显示
, 显示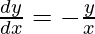
解决方案:
Here, Let multiply x and y.
xy = (![]() )
)
= (![]() )
)
= (![]() ) (Using identity: sin-1θ + cos-1θ =
) (Using identity: sin-1θ + cos-1θ = ![]() )
)
Let’s differentiate w.r.t x,
![]()
x.![]() + y.
+ y.![]() = 0
= 0
x.![]() + y = 0
+ y = 0
![]()
Hence, Proved !!!