定理–从外部点到圆的切线长度相等–圆| 10级数学

切线是从外部点绘制的直线，该点在圆的圆周上恰好一个点处接触圆。圆的切线可以是无限的。这些切线遵循某些特性，这些特性可用作在圆上执行数学计算的恒等式。

这里，在本文中，我们将学习一种这样的特性，即从外部点到圆的切线的长度相等。

证明：从外点到圆的切线长度相等

如图所示，令PQ和PR为绘制到中心O的圆的两个切线。

建造

画一条线段，从中心O到外部点P {即P是两个切线的相交点}

现在∆POR和∆POQ 。

为了证明它们具有相同的长度，我们将首先证明两个三角形是相似的。

我们知道切线与圆的半径成直角。

在这里，OR和OQ是圆的半径

因此， ∠OQP=∠ORP= 90°
现在，很明显，三角形∆POQ和∆POR都是直角三角形，并且它们之间有一个共同的斜边OP。

证明

现在证明三角形∆POQ和∆POR之间的相似性

这里，

QPQO =∠PRO= 90°
它们之间的共同斜边OP。

并且OQ = OR [圆的半径]。
因此，根据RHS相似性规则

∆POQ〜∆POR

因此，两个三角形彼此相似。

所以，
OP / OP = PQ / PR = OQ / OR

PQ / PR = 1 {因为OP / OP = 1}；

因此，PQ = PR；

因此，证明从外点到圆的切线长度相等。

基于定理的样本问题

问题1：从一个外部点在3 cm面积的圆上绘制两个切线。如果圆心和外点之间的距离为5 cm，则求出由圆的两个半径和两个切线形成的四边形的面积。

解决方案：

It is taken note that if PO is joined, then ΔPQO will be right-angled at Q, and so the Pythagoras Theorem applies: 

Given that : 
OQ = 3 cm 
OP = 5 cm 
Using Pythagoras we can find the OP: 
OP^2 = OQ^2 + PQ^2 
25 = 9 + QP^2 
Qp = 4 cm. 

由于这两个切线的长度相同，因此我们知道三角形是全等的，因此两个三角形的面积相同。

Therefore, 

Total Area = 2 times the area of the triangle 
Area = 2 * (1/2) * 4 * 3 
Area = 12 cm^2 

Hence the total Area is 12 cm^2. 

问题2：从圆S内的点P可以在圆S上绘制多少切线?解释您的发现。

解决方案：

The answer is none. But the important part here is the explanation. 
Suppose If PA is a tangent to Circle S from an internal point P, then the points P, O and A will form a right-angled triangle with hypotenuse OP. 
We know that OA is a radius of circle S, since P is inside S, OP must be less than OA ( from the rule of the hypotenuse in a right-angled triangle). Thus, the above-assumed triangle cannot exist. 
Hence, proved that no tangent can be drawn from an interior point P to circle S. 

问题3：在四边形ABCD上刻一个圆，证明AB + CD = AD + BC。
该图仅供参考。

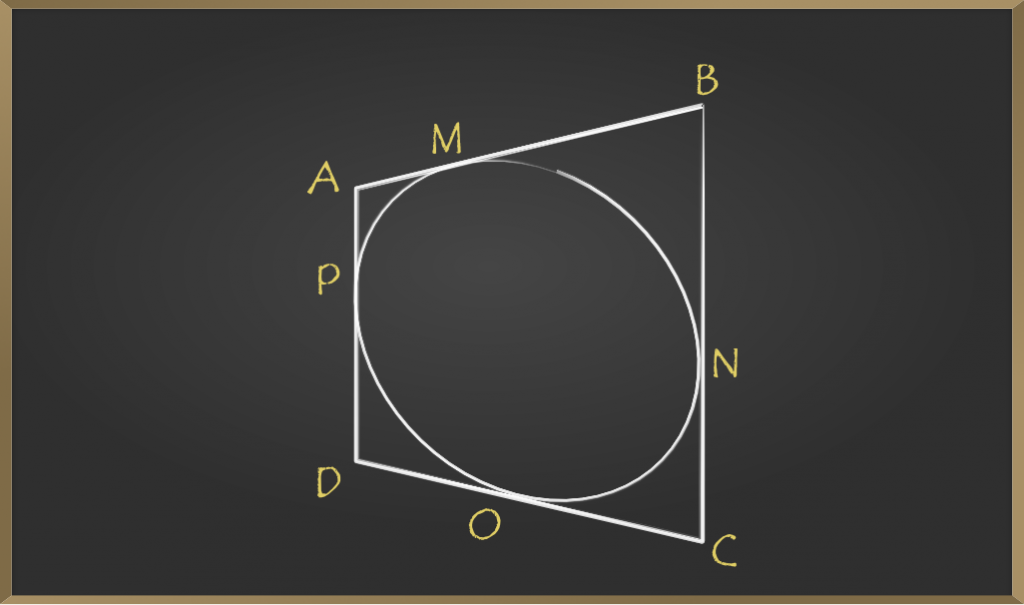
解决方案：

We Already know that the length of Tangents drawn from point A will be the same. 
From this, we infer that, 
AP = AM —(1) 

Similarly, for tangents drawn from point B, 
BN = BM —(2) 

In the same manner for the Tangents drawn from point C, 
CN = CO —(3) 

In the same manner for the Tangents drawn from point D, 
DP = DO —(4) 

Adding equations (1),(2), (3) ,(4), 
We have : 
AM + BM + CO + DO = AP + BN + CN + DP 

Now; 
⇒ AP + PD + BN + NC = AM + MB + DO + OC 
⇒ AD + BC = AB + CD 

Hence proved. 

问题4：从一个外部点B，将切线BC和BD绘制到一个以A为中心的圆上，使得每个切线的长度为4 cm，AB = 5 cm。圆的半径是多少?

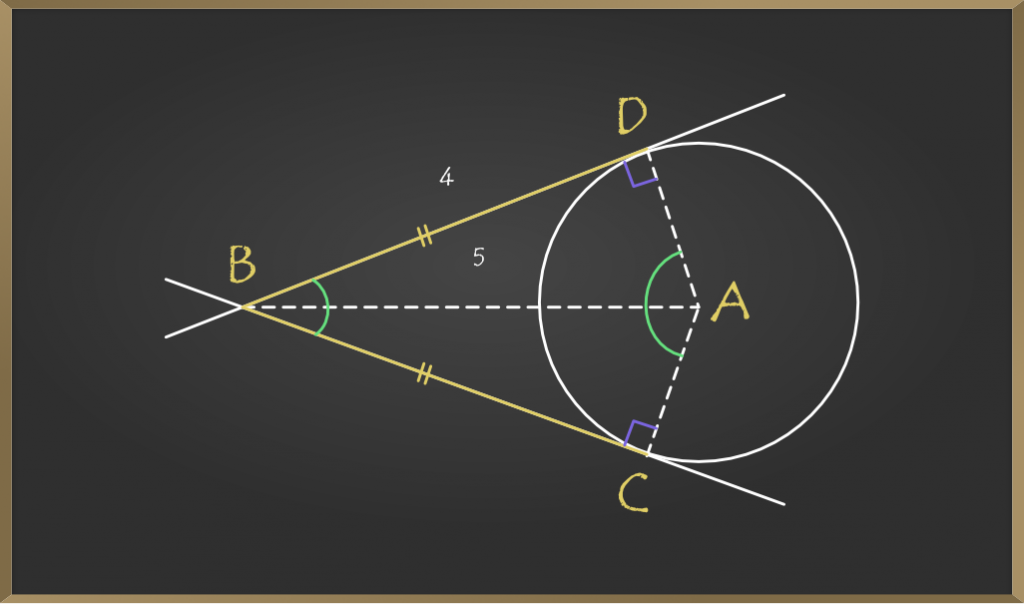
解决方案：

It is taken note that if AB is joined, then ΔABC will be right-angled at C, and so the Pythagoras Theorem applies: 

AB² = AC² + BC² 
AC² = AB² – BC² = 25 – 16 = 9

Hence, AC = 3