几何是处理不同形状和图形的数学的重要组成部分。三角形是几何的重要组成部分,中点定理指向三角形的中点。
什么是中点定理?
该定理指出:“连接三角形两侧的中点的线段平行于三角形的第三侧,并且是其一半”
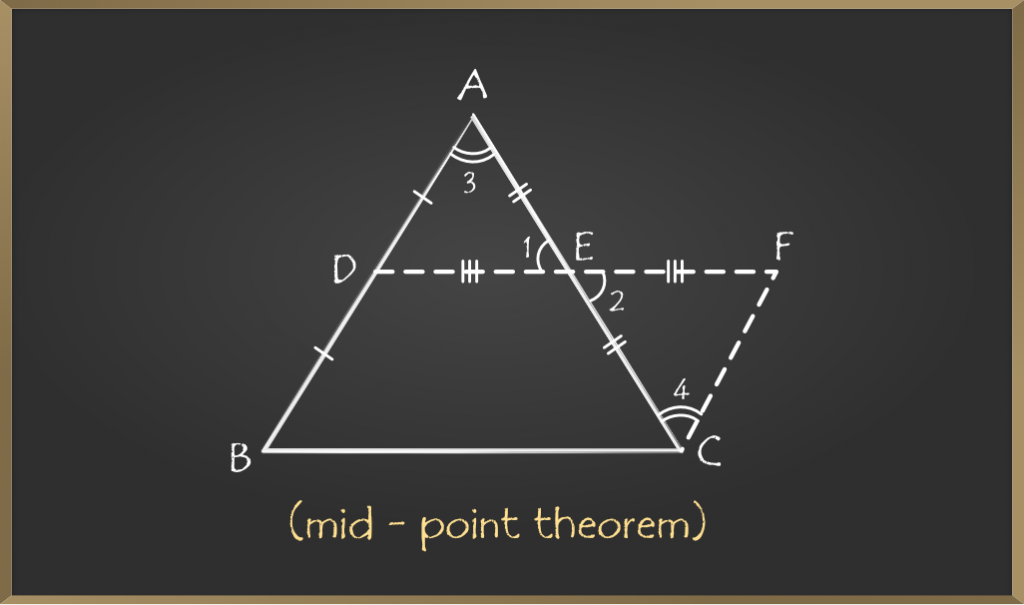
中点定理的证明
三角形ABC,其中D是AB的中点,E是AC的中点。
证明: DE∥BC和DE = 1/2(BC)
建造
将线段的连接点D和E延伸到F,使DE = EF,然后连接CF。
证明
In ∆AED and ∆CEF
DE = EF (construction)
∠1 = ∠2 (vertically opposite angles)
AE = CE (E is the mid-point)
△AED ≅ △CEF by SAS criteria
所以,
∠3 =∠4 (c.p.c.t)
But these are alternate interior angles.
So, AB ∥ CF
AD = CF(c.p.c.t)
But AD = DB (D is the mid-point)
Therefore, BD = CF
In BCFD
BD∥ CF (as AB ∥ CF)
BD = CF
BCFD是平行四边形,因为一对相对的边平行且相等。
所以,
DF∥ BC (opposite sides of parallelogram)
DF = BC (opposite sides of parallelogram)
As DF∥ BC, DE∥ BC and DF = BC
But DE = EF
So, DF = 2(DE)
2(DE) = BC
DE = 1/2(BC)
因此,证明了连接三角形两边中点的线平行于第三边,并且是第三边的一半。
中点定理的反义词是什么?
穿过平行于三角形底边的三角形的一侧的中点绘制的线将三角形的第三侧等分。
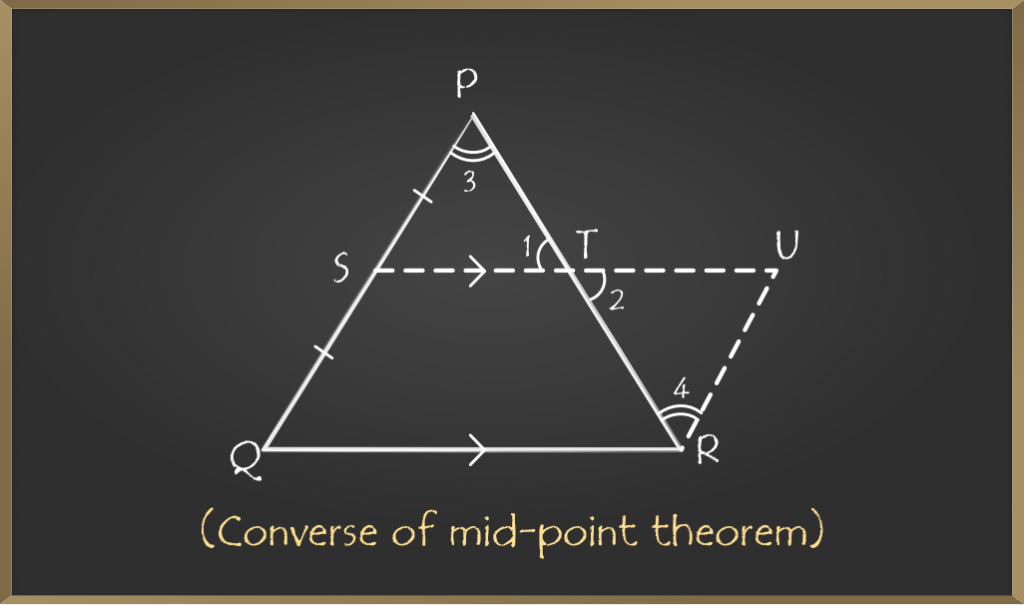
定理证明
在三角形PQR中,S是PQ和ST∥QR的中点
证明: T是PR的中点。
建造
通过R画一条与PQ平行的线并将ST延伸到U。
证明
ST∥ QR(given)
So, SU∥ QR
PQ∥ RU (construction)
Therefore, SURQ is a parallelogram.
SQ = RU(Opposite sides of parallelogram)
But SQ = PS (S is the mid-point of PQ)
Therefore, RU = PS
在△PST和△RUT中
∠1 =∠2(vertically opposite angles)
∠3 =∠4(alternate angles)
PS = RU(proved above)
△PST ≅ △RUT by AAS criteria
Therefore, PT = RT
T is the mid-point of PR.
中点定理的样本问题
问题1: l,m和n是三条平行线。如图所示,p和q是两条相交的平行线,分别位于A,B,C,D,E和F处,如果AB:BC = 1:1,则求出DE与EF之比。

给定: AB:BC = 1:1
查找: DE:EF
构造:加入AF,使其与G处的线m相交。
In △ACF
AB = BC(1:1 ratio)
BG∥ CF(as m∥n)
Therefore, by converse of mid-point theorem G is the midpoint of AF(AG = GF)
Now, in △AFD
AG = GF(proved above)
GE∥ AD(as l∥m)
Therefore, by converse of mid-point theorem E is the mid-point of DF(FE = DE)
So, DE:EF = 1:1(as they are equal)
问题2:在下面给出的图中,L,M和N分别是三角形PQR的边PQ,QR和PR的中点。
如果PQ = 8厘米,QR = 9厘米,PR = 6厘米。找出由L,M和N连接而成的三角形的周长。
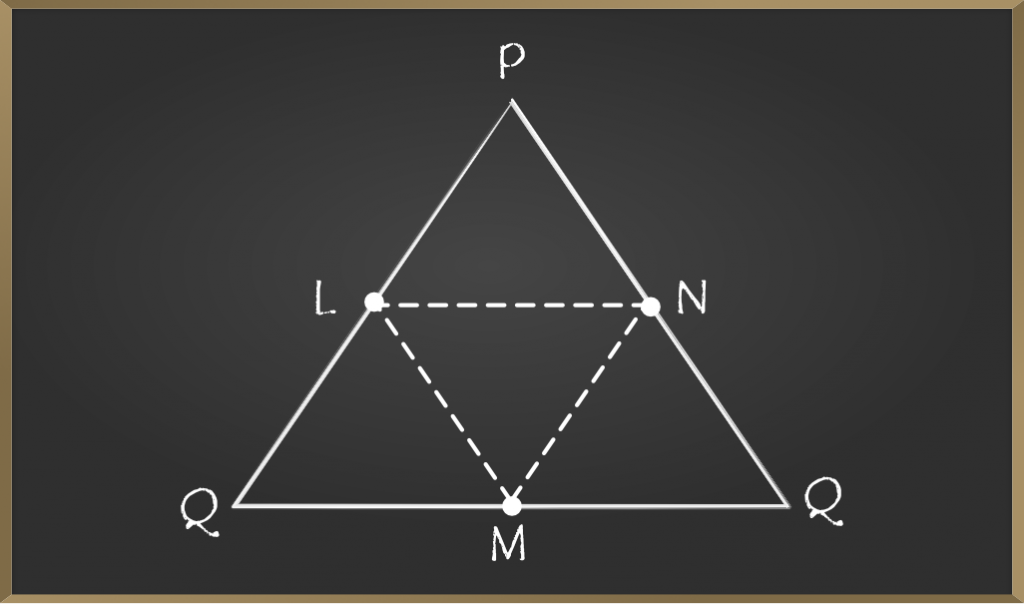
解决方案:由于L和N是中点
By mid-point theorem
LN ∥ QR and LN = 1/2 * (QR)
LN = 1/2 × 9 = 4.5cm
Similarly, LM = 1/2 * (PR) = 1/2×(6) = 3cm
Similarly, MN = 1/2 * (PQ) = 1/2 × (8) = 4cm
Therefore, the perimeter of △LMN is LM + MN + LN
= 3 + 4 + 4.5
= 11.5cm
Perimeter is 11.5cm
结果:通过连接三角形侧面的中点形成的三角形的周长是三角形的一半。