算术是数字的游戏,每个数字都分为一组或另一组,例如:存在复合数字,偶数,奇数,质数。质数是可以作为每个数字的一部分的质数。如果将一个数字分解为较小的数字,则存在于该数字中的最小数字就是质数。
质数
Prime numbers are the numbers that have only and only 2 factors. They are 1 and the number itself. For example: 2,3,5,7,11,13 and so on.
所有自然数都可以写为其主要因子的乘积。例如:24 = 2×3×2×2或13 = 13×1,依此类推。我们可以说反之亦然吗?可以通过乘以质数来获得任何自然数吗?这个问题由T他基本运算定理,也被称为独特的质数分解定理回答。算术基本定理的主要意义在于,它揭示了素数分解的唯一性。
算术基本定理
让我们以一组质数为例,例如{3,2,7}。您认为我们可以从它们的乘法中得出多少个数字? 3×2 = 6,3×3×2 = 18,7×2 = 14,依此类推。因此,我们可以说,可以用这些素数生成无数个数。但这是否证明我们可以生成所有可能的数字?
是的,有无限多个可能的质数,从它们的乘法中,我们可以生成无限数,这就是算术基本定理的关键。为了进一步发展这个概念,让我们看一下数字的因式分解。
假设给定一个数字x = 36。

上图表示数字的因式分解树。 36 = 2×2×3×3。它是质数的乘积。如果我们继续尝试不同的数字,我们会发现所有数字都可以表示为质数。以更正式的方式
定理:
Every composite number can be expressed (factorized) as a product of primes, and this factorization is unique, apart from the order in which the prime factors occur.
This is called Fundamental Theorem of Arithmetic.
该定理表明,除了质数出现的顺序外,每个复合数都可以以“唯一”的方式重写为质数的乘积。
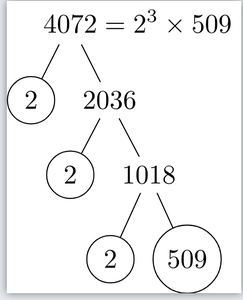
问题1:分解数字“ 4072”,并以树形表示。
回答:
问题2:分解数字“ 324”并以树形式表示。
回答:
问题3:分解数字“ 16048”,并以树形表示。
回答:
使用算术基本定理的LCM和HCF
- 被称为最高公因数的HCF是最大数,该最大数将给定的两个数相除。
- LCM是最低公倍数,是所有公质数的乘积,但具有最高的度数/幂。
例如:
问题1 :找到24和36的LCM和HCF?
解决方案:
The Prime factors of 24 = 2× 2×2×3
The prime factors of 36 = 2×2×3×3
HCF = 2×2×2×3, 2×2×3×3 = 2×2×3 = 12
LCM =
2×2×2×3×3 = 72
LCM和HCF也可以借助质因数分解找到,让我们看一些示例。
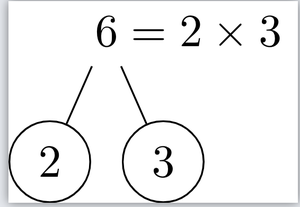
问题2:找到数字6和20的LCM和HCF。
回答:
Prime Factorization of 6 can be represented in the following way,
Prime Factorization of 20 can be represented in the following way,
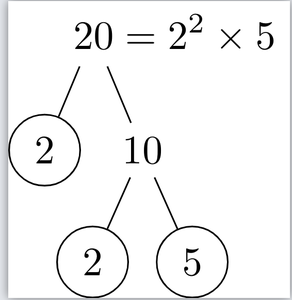
So, now we have prime factorization of both the numbers,
6 = 2 × 3
20 = 2 × 2 × 5
We know that
HCF = Product of the smallest power of each common prime factor in the numbers.
LCM = Product of the greatest power of each prime factor, involved in the numbers.
So, HCF(6,20) = 21
LCM(6,20) = 22 × 31 × 5
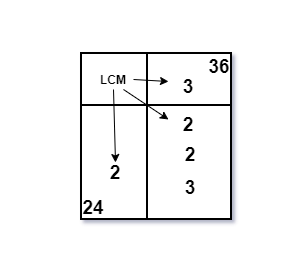
问题3:找到数字24和36的LCM和HCF。
回答:
Prime Factorization of 24:
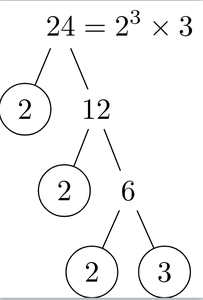
Prime Factorization of 36:

24 = 23 × 3 and 36 = 22 × 32
Based on the previous definitions,
HCF(24, 36) = 12
LCM(24, 36) = 72
Fact: In the above examples, notice that for any two numbers “a” and “b”. HCF × LCM = a × b.
问题4:假设对于两个数字“ a”和“ b”。给出的HCF为120,两个数字的乘积为3600。找到两个数字的LCM。
回答:
Given two numbers “a” and “b”.
LCM(a, b) is unknown while HCF(a, b) = 120 and a × b = 3600.
From the property studied above,
HCF(a, b) × LCM(a, b) = a × b
Plugging in the given values.
120 × LCM(a, b) = 3600
LCM(a, b) = 30