微积分基本定理

在数学中，微积分是一个分支，有助于理解与函数相关的值之间的变化。它广泛应用于物理、工程、医学、经济学、生物学、太空探索、统计学、药理学等领域。没有微积分，连房子都建不起来。

微积分的历史可以追溯到古埃及。历史学家说，埃及人用来计算锥体截头体体积的方法是积分法。他们知道积分的基本功能，并用它们来计算体积和面积。然而，现代微积分是由艾萨克·牛顿和戈特弗里德·威廉·莱布尼茨独立开发的。

微积分有两个分支：

1. 微积分：在微积分的这个分支中，我们将事物分成不同的部分，并研究它们如何从一个时刻到下一个时刻变化。例如，速度随时间的变化。
2. 积分微积分：在这个微积分分支中，我们将小块连接在一起以了解它们的整体行为。例如，在确定连接两个变电站所需的电力电缆长度时。

在本文中，我们将讨论连接微积分两个分支的微积分基本定理，但在此之前，我们首先需要了解面积函数。

面积函数

让我们考虑一个在区间[a, b]中连续的函数f(t) ，如下图所示：

现在，让我们在图上标记 a 和 b 之间的某个点 x。

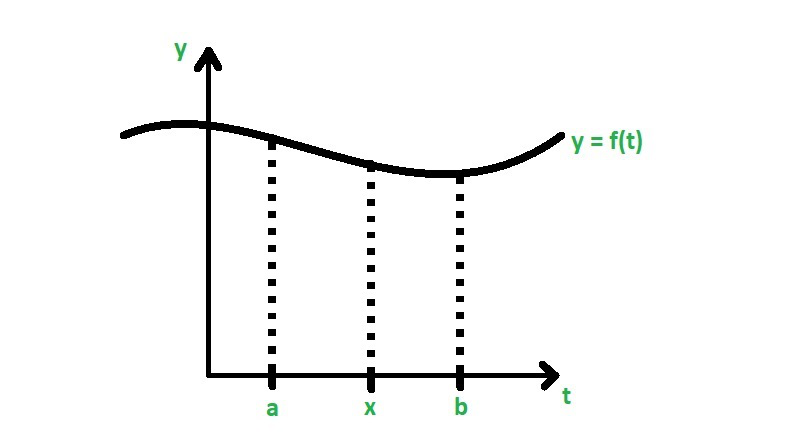
现在，我们必须找到区间[a, x]之间曲线y = f(t)下的面积。

因此，a 和 x 之间的曲线下面积是 f(t) dt 从 a 到 x 的定积分，为

A(x) = ∫_a^x f(t) dt

这里 A(x) 被称为面积函数，它有助于找到微积分的基本定理。或者换句话说，A(x)，即∫ _a ^x f(t) dt 是t 轴上以a 和b 为坐标的曲线y = f(t) 所包围的区域的面积。如果 x 是 [a, b] 区间之间的一个点，则∫ _a ^x f(t) dt 将表示阴影区域的面积，即 A(x)。

例子：

Let’s find out the value of A(x) for function y = 2x between x = 2 and x = 6.

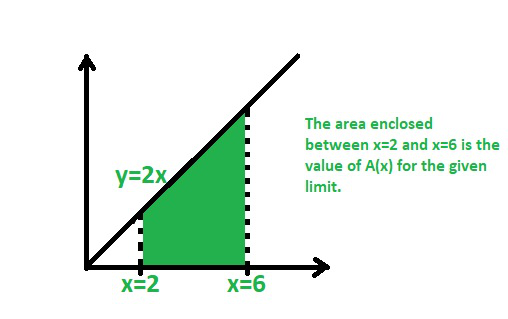
A(x) = ∫₂⁶ 2x dx = [x²]₂⁶ = 6² – 2² = 36 – 4 = 32 

微积分基本定理

微积分的基本定理是数学中强有力的定理。它建立了差异化和整合之间的关系。现在，这种关系为我们提供了一种无需计算面积或使用黎曼和即可评估确定内部的方法。基本定理分为两部分：

1. 第一基本定理
2. 第二基本定理

现在我们将一一详细讨论每个定理：

第一基本定理

第一个基本定理指出，如果 f(x) 是闭区间 [a, b] 上的连续函数，并且函数F(x) 定义为

dF/dx = d/dx(∫_a^x f(t) dt) = f(x)

或 F'(x) = f(x) 在 [a, b] 上

或者换句话说，如果 f 是闭区间 [a, b] 上的连续函数，并且面积函数是 A(x)，则

A'(x) = f(x) ∀ x ∈ [a, b]

证明：

According to the fundamental theorem of calculus, we have

F'(x) = ∫_a^x f(t) dt = f(x)           …(A)

Now, the equation (A) can be written as

F'(x) = lim _h⇢0 (F(x + h) – F(x)/h

= lim _h⇢0 1/h [( ∫_a^x+h f(t) dt – ∫_a^x f(t) dt )]       …(B)

Thus, equation (B) represents the area under the curve y = f(t) on the 

interval [x, x + h]. Now, equation (B) can be written as

F'(x) = lim _h⇢0 1/h (∫_x^x+h f(t) dt         …(C)

Now, according to the mean value theorem of the definite integral,

if there exists a c such that x ≤ c ≤ x + h then

f(c) = 1/h ∫_x^x+h f(t) dt

Thus,

F'(x) = lim _h⇢0 f(c)

Since c is in between x and x + h, therefore c⇢x as h⇢0. 

Also, since f(x) is continuous, therefore we have,

lim _h⇢0 f(c) = lim _c⇢x f(c) = f(x)

Thus,

F'(x) = lim _h⇢0 1/h ∫_x^x+h f(t) dt

= lim _h⇢0 f(c)

= f(x)

Hence, proved.

笔记：

• 每个连续函数f 都有一个反导数 F(x)。
• 微积分的基本定理将积分微积分和微积分联系起来。

使用微积分基本定理求导数：

让我们借助一个例子来讨论这个概念：

Let’s say we have a function F(x) = ∫₂₁^x √(t³) dt and we have to find F'(4).

So, according to the fundamental theorem of calculus, the given equation becomes

 F'(x) = √(x³)

Thus, F'(4) = √(4³) = 4^3/2 = (4^1/2)³ = (√4)³ = 2³ = 8.

This is how we solve questions when we have to apply the fundamental theorem of calculus.

用微积分基本定理求导数：x 在两个边界上：

让我们借助一个例子来讨论这个概念：

Suppose there’s a function y = f(t) = sin t/t which is continuous in [x, x²] and we have to find it’s antiderivative. Let’s consider the following diagram :

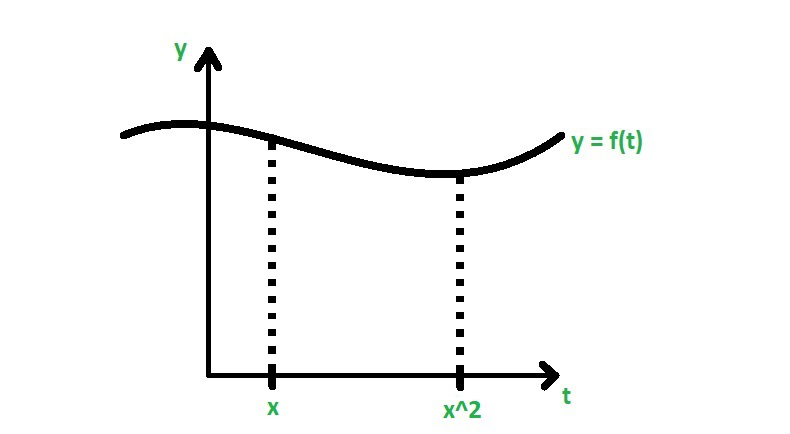
According to the fundamental theorem of calculus,

$\frac{dF}{dx} = \frac{d}{dx} ( \int_{x}^{x^2} \frac{sin t}{t} dt ) = f(t)$   …..(1)

Now, mark c on the graph somewhere in between x and x² on the graph.

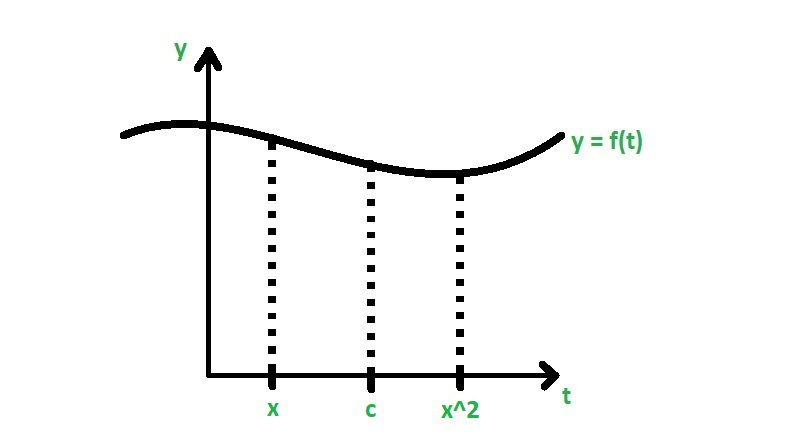
Now, equation (1) becomes

dF/dx = d/dx ( ∫_x^c sin t/t dt ) + d/dx ($\int_{c}^{x^2} \frac{sin t}{t} dt$)

= – d/dx ( ∫_c^x sin t/t dt ) + d/dx ($\int_{c}^{x^2} \frac{sin t}{t} dt$)

= – sin x/x + (sin x²/x²).2x

= (2 sin x² – sin x)/x

Thus, we get

F'(x) = (2 sin x² – sin x)/x

Hence, this is how we find derivatives of the functions where x is on both bounds.

微积分第二基本定理

微积分的第二个基本定理指出，如果 f(x) 在闭区间 [a, b] 上是连续的，并且 F(x) 是 f(x) 的反导数，那么

∫_a^b f(x) dx = F(b) – F(a)

第二个基本定理也称为评估定理。

• 这个定理说 ∫ _a ^b f(x) dx = "f" 在上限 b 的反导数 F 的值 – 与下限 a 相同的反导数。
• 在∫ _a ^b f(x) dx 表达式中，函数f(x) 必须定义明确且在 [a, b] 区间内连续。
• 这是一个非常有用的定理。它提供了一种估计定积分的方法，而无需找到总和的极限。
• 这里，在估计定积分时，主要操作是找到一个函数，其导数是积分方程，这个过程将加强微分和积分关系。

证明：

Let us consider M = x_i, i = 0, 1, …, n is a regular partition of [a, b]

Then 

F(b) – F(a) = F(x_n) – F(x₀)

= $\sum_{i=1}^n$[F(x_i) – F(x_{i – 1})]   …..(1)

Now, As we know that F is the antiderivative of f on the closed interval [a, b], 

So using the mean value theorem, we get

i = 0, 1, 2, …,n

Now we find the value of c_i in [x_i-1, x_i]

So, 

F(x_i) – F(x_{i – 1}) = F'(c_i)(x_i – x_i-1) = $\sum_{i=1}^n$f(c_i)△x

Now put this value in equation(1), we get

F(b) – F(a) =  $\sum_{i=1}^n$f(c_i)△x

Now taking limit on both sides, i.e., n -> ∞, we get

F(b) – F(a) = lim_n->∞ $\sum_{i=1}^n$f(c_i)△x = ∫^b_a f(x)dx

Hence proved

示例问题

问题1.计算函数的导数$F(x) = \int_{1.5}^x \sqrt{(t^2 + 3t)} dt$在 x = 3。

解决方案：

Given that $F(x) = \int_{1.5}^x \sqrt{(t^2 + 3t)} dt$

So, using first FTC, we get

F'(x) =  $= \frac{d}{dx} [ \int_{1.5}^x f(t) dt$= √(x² + 3x)

Therefore,

F'(3) = √(3² + 3.3) = √(9 + 9) = √(2.9) = 3√2.

问题2.计算函数的导数$F(x) = \int_{\frac{-\pi}{2}}^x \sqrt{(sin t + cos t)}dt$ x = π /2处的 dt 。

解决方案：

Given that $F(x) = \int_{\frac{-\pi}{2}}^x \sqrt{(sin t + cos t)}dt$

So, using first FTC, we get

F'(x) = $\frac{d}{dx} [\int_{\frac{-\pi}{2}}^x f(t) dt$ = f(x)

Hence,

F'(x) = √(sin x + cos x)

Therefore,

F'(π/2) = √(sin π/2 + cos π/2) = √(1 + 0) = 1.

问题 3. 求导数$F(x) = \int_{3}^{x^2} \frac{dt}{t^2}$ .

解决方案：

Given that $F(x) = \int_{3}^{x^2} \frac{dt}{t^2}$

So, let x² = u. 

Now, consider a new function,

G(u) = ∫₃^u dt/t² 

⇒ G'(u) = 1/u²

Since, F(x) = G(x²)

⇒ F'(x) = G'(x²).2x

⇒ F'(x) = (1/x⁴).2x

⇒ F'(x) = 2/x³.

问题 4. 求导数$F(x) = \int_{0}^{x^2} \sqrt{(1+t^3)}dt$ .

解决方案：

Given that $F(x) = \int_{0}^{x^2} \sqrt{(1+t^3)}dt$

So, let x² = u. 

Now, consider a new function,

G(u) = ∫₀^u √(1 + t³) dt

Using first FTC, we have

G'(u) = √(1 + u³)

As F(x) = G(x²)

⇒ F'(x) = G'(x²).2x

⇒ F'(x) = 2x √(1 + (x²)³)

⇒ F'(x) = 2x √(1 + x⁶).

问题 5. 求函数的导数$F(x) = \int_{1}^{x^2} (t^2+t) dt$ .

解决方案：

Given that $F(x) = \int_{1}^{x^2} (t^2+t) dt$

So, let x² = u. 

Now, considering new function,

G(u) =  ∫₁^u (t² + t) dt.

Using first FTC, we get

G'(u) = u² + u

Since, F(x) = G(x²)

⇒ F'(x) = G'(x²).2x

⇒ F'(x) = (x⁴ + x).2x

⇒ F'(x) = 2x⁵ + 2x².

问题 6. 评估积分$\int_{x}^{x^2} t dt$ .

解决方案：

Given that $\int_{x}^{x^2} t dt$

Now we find the anti-derivative of t

∫t dt = t² + C

Now using the second FTC, we get

∫_a^b f(x) dx = F(b) – F(a)

$\int_{x}^{x^2} t dt$ = F(x²) – F(x)

$\int_{x}^{x^2} t dt$ = ((t²)²) – (t²)

$\int_{x}^{x^2} t dt$ = (t⁴) – (t²)

$\int_{x}^{x^2} t dt$ = t²((t²) – 1)

问题 7. 评估积分$\int_{1}^{2} x dx$ .

解决方案：

Given that I = $\int_{1}^{2} x dx$

Now using the second FTC, we get

∫_a^b f(x) dx = F(b) – F(a)

$\int_{1}^{2} x dx$ = F(2) – F(1)

Now we find the anti-derivative of x

∫x dx = x² + C

$\int_{1}^{2} x dx$ = (2²) – 1

= 4 – 1

= 3

问题 8. 求积分 ∫ ₀ ² (x ² – x) dx。

解决方案：

Given I = ∫₀² (x² – x) dx

Using second FTC, we get

∫_a^b f(x) dx = F(b) – F(a)

∫₀² (x² – x) dx = F(2) – F(0)

Now we find the anti-derivative of x

∫₀² (x² – x) dx = x³/3 – x²/2

So, 

= [x³/3 – x²/2]₀²

= [8/3 – 4/2]

= [8/3 – 2]

= [8/3 – 6/3]

= 2/3.

问题 9. 计算积分 F(x) = ∫ ₀ ^x e ^t + e ^-t dt。

解决方案：

Given that F(x) = ∫₀^x e^t + e^-t dt

Using the first FTC, we get

F'(x) = e^x + e^-x.

问题 10. 求积分$\int_{0}^{ln 2} t.e^{-t} dt$ .

解决方案：

Given I = $\int_{0}^{ln 2} t.e^{-t} dt$

so we can also write as

I = $\int_{0}^{ln 2} t.d(e^{-t})$

Now apply integration by parts, we get

u = t, dv = d(e^-t)

So, du = 1, v = e^-t

So, the integral is

I = –$\int_{0}^{ln 2} t.d(e^{-t})$

=$[(te^{-t})|^{ln2}_0 - \int_0^{ln2} e^{-t} dt]$

= $[(te^{-t})|^{ln2}_0 + \int_0^{ln2} e^{-t} dt]$

=$-(te^{-t})|^{ln2}_0 -(e^{-t})|^{ln2}_ 0$

= $- [e^{-t}(t + 1)]|^{ln2}_0$

= -e^-ln2(ln2 + 1) + e⁰ .1

= 1/2(ln e – ln 2)

= 1/2ln e/2