复数的极坐标表示

我们对复数非常熟悉，我们知道形式为(x + iy)的数字，其中x＆y是实数，而i(iota)是虚数单位。到现在为止，我们大部分讨论了(x + iy)形式的复数，这是最常用的复数的代数形式或几何形式，但是也有其他形式的复数。我们将介绍复数的另一种重要形式，即极形式或三角形式。

复数的几何或代数形式

复数z =(x + iy)由Argand平面上的点P(x，y)表示，并且Argand / Complex平面上的每个点都表示唯一的复数。如果复数是纯实数，则它的虚部为Im(z)= 0，并且恰好位于实轴(X轴)上，而纯虚数的复数具有其实部Re(z)= 0且位于正好在假想轴(Y轴)上。

Note: If a point P (x, y) represents a complex number z on the Argand plane then the complex number Z = (x + i y) is known as the affix of the point P.

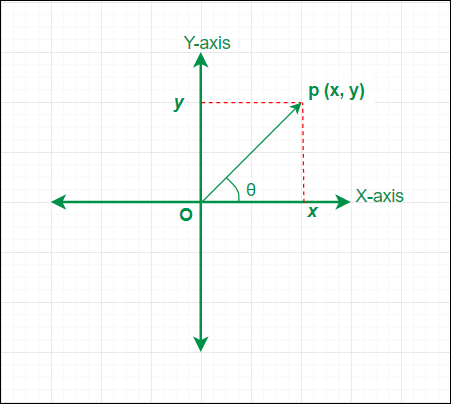
(z)在Argand平面上的几何表示

在上述线段的复数长度表示中，OP等于复数的模数，角度θ是OP在X轴正方向上沿逆时针方向形成的角度，称为由amp(z)或arg(z)表示的复数的幅度或自变量。

From the above figure, θ can be calculated as follows

tan(θ) = y / x

tan(θ) = |Im(z) / Re(z)|

θ = tan^-1{|Im(z) / Re(z)|}

Note: The angle θ can have infinite values in the multiple of 2π. But the unique value of θ that lies in the range (-π ≤ θ ≤ π) is called the principal argument or principal value of the amplitude. The point to be remembered is the value of the principal argument of a complex number (z) depends on the position of the complex number (z) i.e the quadrant in which the point P representing the complex number (z) lies.

让我们讨论不同的情况，以找出主体参数的值。令α为OP相对于X轴的锐角，θ为复数(z)的主要参数。

情况1.当复数z =(x + iy)位于第一象限，即x> 0＆y> 0时，则主参数的值(θ=α)。

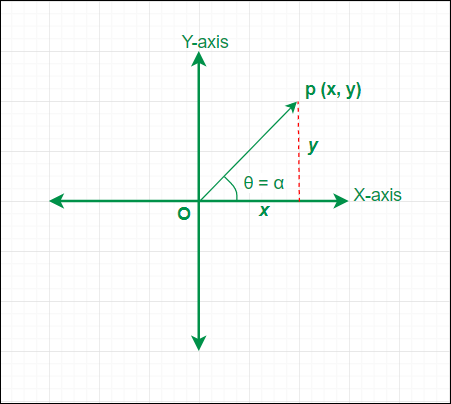
Z =(x + iy)在第一象限

情况2。当复数z =(x + iy)位于第二象限，即x <0＆y> 0时，则主参数的值(θ=π–α)。

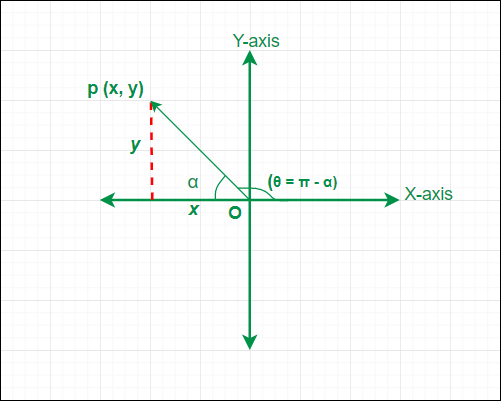
Z =(x + iy)在第二象限

情况3。当复数z =(x + iy)位于第三象限，即x <0＆y <0时，则主参数的值(θ=α–π)。

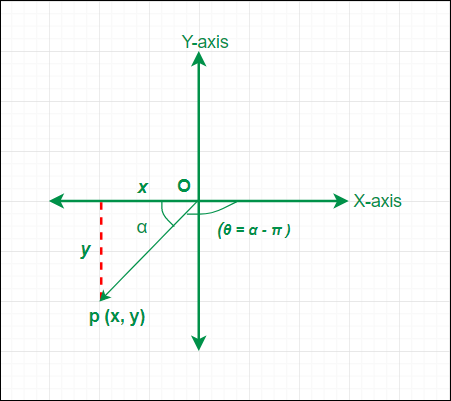
Z =(x + iy)在第三象限

情况4。当复数z =(x + iy)位于第四象限，即x> 0＆y <0时，则主参数的值(θ=-α)。

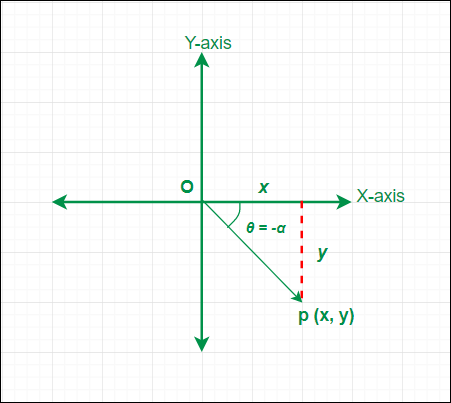
Z =(x + iy)在第四象限

复数的极性或三角形式

我们已经看到了复数(z)的几何形式，其中在Argand平面上由(x，y)表示，OP = | z |。且arg(z)=θ。现在，我们将使用复数的几何形式获得其极性形式。在极坐标或三角函数形式中，复数(z)由(r，θ)表示。其中r = | z | ＆θ＝ arg(z)。

z的极坐标形式(r，θ)

从上图可以发现OM = x = | z | cosθ和MP = y = | z |正弦θ;

Now put the values of x & y in z = (x + i y) and we get z = |z| cos θ + i |z| sin θ 

Taking |z| common, z = |z| (cos θ + i sin θ) 

And putting |z| = r ;

The polar form of a complex number (z) is given by

z = r (cos θ + i sin θ) ; 

If we take the general value of the argument arg(z) = 2n π + θ, then the polar form of z is given by

z = r [cos (2n π + θ) + i sin (2n π + θ)] ; where n is an integer.

当我们在z的极坐标形式的表达式中有θ时，根据四个象限中的主要自变量值θ再次会有四种不同的情况。让我们使用上面获得的主要自变量θ的结果来讨论它们。

• 情况1.当复数z位于第一象限时，则主参数的值(θ=α)。因此，z = r(cosα+ i sinα)的极性形式。
• 情况2。当复数z位于第二象限时，则主参数的值(θ=π–α)。因此，z = r [cos(π-α)+ i sin(π-α)]或z = r(-cosα+ i sinα)的极形式。
• 情况3。当复数z位于第三象限时，则主自变量的值(θ=α–π)。因此，z = r [cos(α-π)+ i sin(α-π)]或z = r(-cosα-i sinα)的极形式。
• 情况4。当复数z位于第四象限时，则主参数的值(θ=-α)。因此，z = r [cos(-α)+ i sin(-α)]或z = r(cosα– i sinα)的极性形式。

正如我们讨论了复数的形式，我们还了解到，可以很容易地从其几何/代数形式z(x，y)获得复数z(r，θ)的极性形式。让我们看一下将代数形式的复数转换为极数形式的一个示例问题。

例子

例1：如果(z = -i)或(z = 0 – i)是代数形式的复数，则其极性形式表示为

解决方案：

As z = (0 – i) ≈ P (0, -1), it lies on the Y-axis (θ = -α)

α = tan-1(Im(z) / Re(z))

α = tan-1(|(-1) / 0|) = tan^-1(|-∞|) = π / 2

θ = -α = -(π / 2)

r = |z| = √(0² + 1²) = 1

z = r (cos θ + i sin θ)

z = 1 [cos(π / 2) – i sin(π / 2)]

示例2：如果z =(1 + i)是代数形式的复数，则其极性形式表示为

解决方案：

As z = (1 + i) ≈ P (1, 1), it lies in the first quadrant then (θ = α)

α = tan^-1(Im(z) / Re(z))

α = tan^-1(1) = π / 4 

θ = α = π / 4

r = |z| = √(1² + 1²) = √2

z = r (cos θ + i sin θ)

z = √2 [cos(π / 4) + i sin(π / 4)]

例3：如果z =(-1 –√3i)是代数形式的复数，则其极坐标形式表示为

解决方案：

As z = (-1 – √3 i) ≈ P (-1, -√3), it lies in the third quadrant then (θ = α – π)

α = tan-1(Im(z) / Re(z))

α = tan-1(|-√3 /(-1)|) = tan-1(√3) = π / 3

θ = (α – π)

θ = (π  / 3) – π = – (2 π)  / 3

r = |z| = √((-1)² + (-√3)²) = √4 = 2

z = r (cos θ + i sin θ)

z = 2 [cos(-(2π) / 3) + i sin((-2π) / 3)]

z = 2 [cos(2π / 3) – i sin(2π / 3)] or

z = 2 [- cos(π / 3) – i sin(π / 3)]