了解四边形–多边形外角的度量

多边形是由直线组成的闭合，连接的形状。它可以是横跨二维的平面图或平面图。多边形是一个封闭的图形，可以包含3个以上的边。形成多边形的线称为边或边，它们相交的点称为顶点。在它们之间共享相同顶点的边称为相邻边。包围在相邻侧面内的角称为内角，外角称为外角。

外角

外部角度基本上是由多边形的任意边与所选边的相邻边的交点形成的。在一对相邻侧面内形成的内角和外角形成一个完整的180度角。

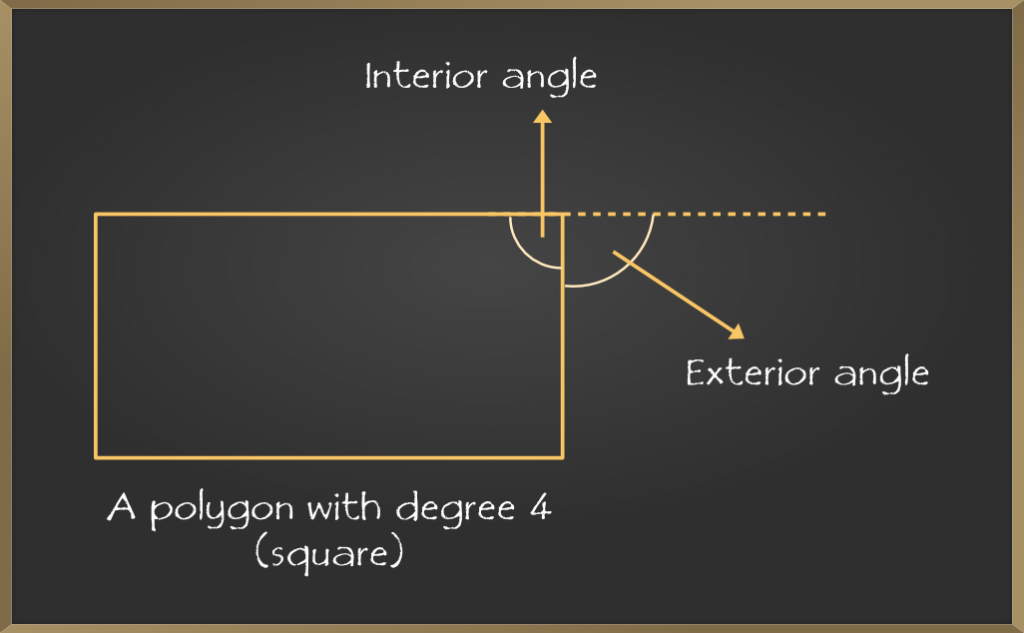
外部角度的度量

1. 它们形成在外部，即角的外部。
2. 在同一侧形成的外角和内角的相应总和= 180°。
3. 多边形的所有外角的总和与边的数量无关，并且等于360度，因为沿顺时针方向或逆时针方向旋转多边形需要一整圈。
4. 如果我们有n个边的正多边形，则每个外角的度量
   =(多边形所有外角的总和)/ n
   =(360度)/ n

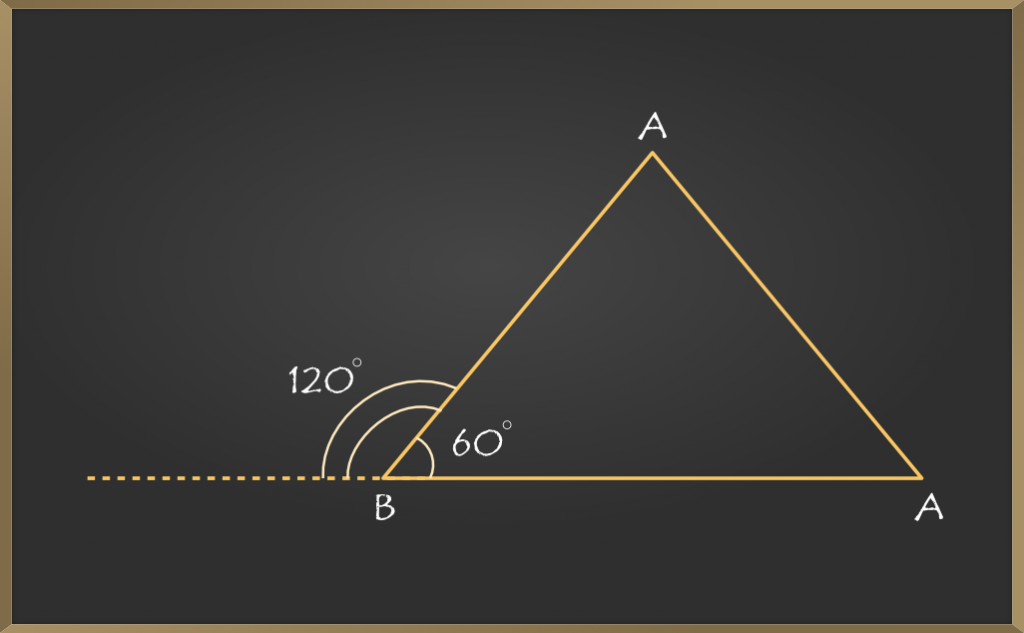
多边形外角和的定理

如果我们观察到一个凸多边形，那么每个顶点处存在的外角之和将为360°。以下定理将解释多边形的外角总和：

证明

让我们考虑一个具有n个边的多边形。外角之和为N。

The sum of exterior angles of a polygon(N) = 

Difference between {the sum of the linear pairs (180n)} – {the sum of the interior angles.(180(n – 2))}

N = 180n − 180(n – 2)     
N = 180n − 180n + 360
N = 360            

Hence, we have the sum of the exterior angle of a polygon is 360°.             

外角样例问题

示例1：找到标有x的外角。

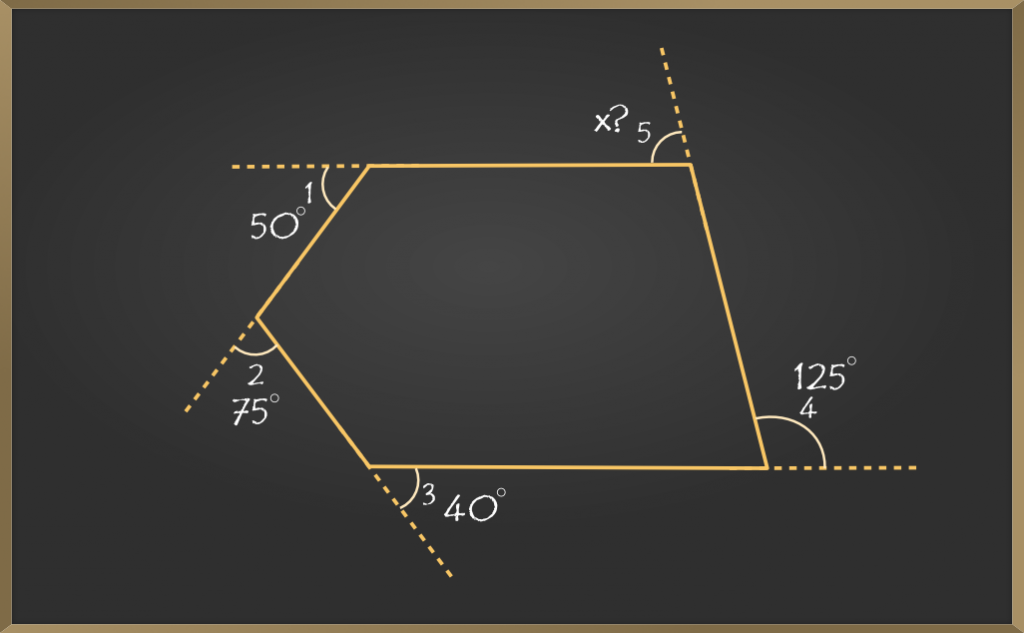
解决方案：

Since the sum of exterior angles is 360 degrees, the following properties hold:

∠1 + ∠2 + ∠3 + ∠4 + ∠5 = 360°
50° + 75° + 40° + 125° + x = 360°
x = 360°

示例2：确定四边形的每个外角。

解决方案：

Since, it is a regular polygon, measure of each exterior angle
=          360°          
Number of sides
=   360°  
       4
= 90°

示例3：找到每个外角等于60度的正多边形。

解决方案：

Since it is a regular polygon, the number of sides can be calculated by the sum of all exterior angles, which is 360 degrees divided by the measure of each exterior angle. 

Number of sides = Sum of all exterior angles of a polygon 
                                                         n
Value of one pair of side = 360 degree 
                                                          60 degree
                                                       = 6
Therefore, this is a polygon enclosed within 6 sides, that is hexagon.

示例4：查找此多边形的内角“ x，y”和外角“ w，z”吗?

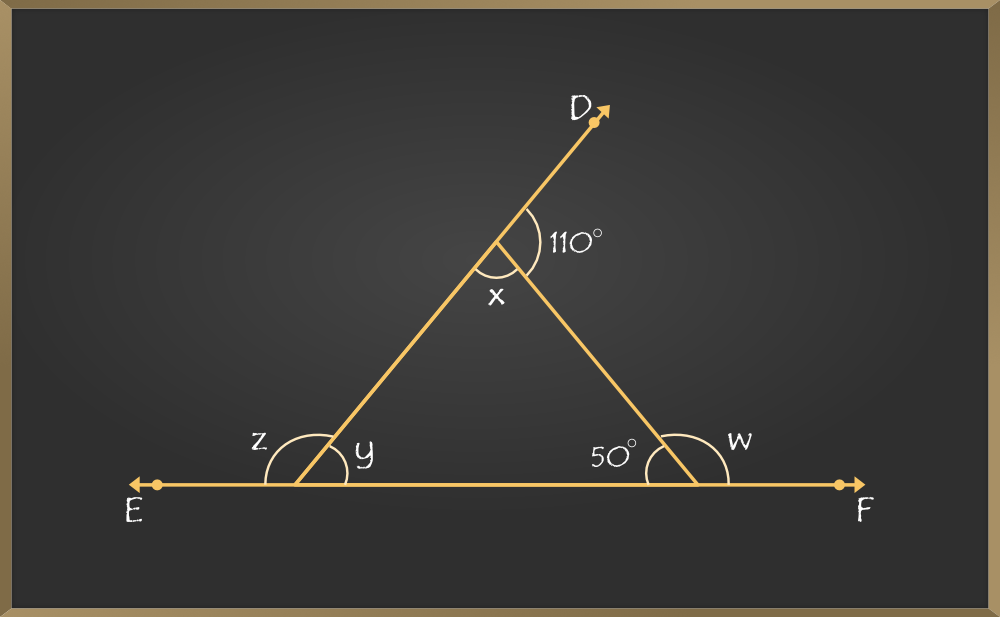
解决方案：

Here we have ∠DAC = 110° that is an exterior angle and ∠ACB = 50° that is an interior angle.

Firstly we have to find interior angles ‘x’ and ‘y’.
∠DAC + ∠x = 180°  {Linear pairs}
110°  + ∠x = 180°  
∠x = 180° – 110°  
∠x = 70°  
Now, 
∠x + ∠y + ∠ACB = 180° {Angle sum property of a triangle} 
70°+ ∠y + 50° = 180°  
∠y + 120° = 180° 
∠y = 180° – 120° 
∠y = 60° 

Secondly now we can find exterior angles ‘w’ and ‘z’.
∠w + ∠ACB = 180° {Linear pairs}
∠w + 50° = 180° 
∠w = 180° – 50° 
∠w = 130° 

Now we can use the theorem exterior angles sum of a polygon,
∠w + ∠z  + ∠DAC = 360° {Sum of exterior angle of a polygon is 360°}
130° + ∠z + 110° = 360° 
240° + ∠z = 360° 
∠z = 360° – 240° 
∠z = 120°