定理–循环四边形的对角之和为180°|第9类数学

在欧几里得几何中，环状四边形或内接四边形是其顶点都位于单个圆上的四边形。该圆称为外接圆或外接圆，并且顶点被称为是环状的。圆的中心及其半径分别称为外接圆心和外接圆半径。这些四边形的其他名称是循环四边形和弦四边形，后者是因为四边形的边是外接圆的弦。

Theorem Statement: The sum of the opposite angles of a cyclic quadrilateral is 180°. 

因此，根据定理陈述，在下图中，我们必须证明

∠BAD+∠BCD= 180 ^o

∠ABC+∠ADC= 180 ^o

证明：

Given:

A cyclic quadrilateral ABCD where O is the centre of a circle.

Construction:

Join the line segment OB and OD

Since, The angle substended by an arc at the centre is double the angle on the circle.

Therefore,

∠BAD = 1/2 (∠BOD)                         – equation 1

Similarly,

∠BCD = 1/2 (reflex of ∠BOD)           – equation 2

By adding equation 1 and 2, we get

∠BAD + ∠BCD = 1/2 (∠BOD + reflex of ∠BOD)

∠BAD + ∠BCD = 1/2 ( 360^o)             {Since , ∠BOD + reflex of ∠BOD form complete angle i.e 360 degree}

∠BAD + ∠BCD = 180^o

Similarly,

∠ABC + ∠ADC = 180^o

Hence proved, that the sum of opposite angles of a cyclic quadrilateral is 180° 

给定定理上的样本问题

问题1：在下图中，ABCD是一个循环四边形，其中inCBA = 91.64°，∠DAB= 102.51°，找到findADC和∠DCB?

解决方案：

By applying theorem, 

The sum of opposite angles of a cyclic quadrilateral is 180° 

We get,

∠ADC + ∠ABC = 180^o

∠ADC + 91.64^o =180^o

∠ADC =180^o – 91.64^o = 88.36 degree

And,

∠DCB + ∠BAD =180^o

∠DCB + 102.51^o = 180^o

∠DCB = 180^o – 102.51^o

∠DCB = 77.49^o

Hence ∠ADC = 88.36^o and ∠DCB = 77.49^o

问题2：在下图中，ABCD是一个循环四边形，其中∠BAD= 100°，而∠BDC= 50°找出∠DBC?

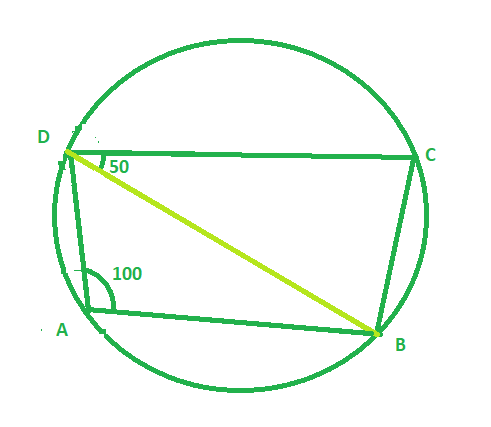
解决方案：

Given :

∠BAD = 100°  and ∠CDB = 50^o

∠BAD + ∠BCD = 180^o   (Since opposite angle of cyclic quadrilateral)

∠BCD = 180^o – 100^o = 80⁰

In ∆BCD ,

∠BCD + ∠CDB + ∠DBC = 180^o

80^o  + 50^o + ∠DBC = 180^o

∠DBC = 180^o  – 130^o

∠DBC = 50^o

Therefore ∠DBC = 50^o