从图表估计限制
极限的概念已经存在了数千年,古代文明的早期数学家使用极限来近似圆的面积。但正式的概念直到 19 世纪才出现。这个概念对于微积分是必不可少的,并且可以作为分析导数、连续性和可微性的基础。直观地说,极限让我们了解值函数在 x 的特定值处逼近。使用这个想法,也可以通过查看图表在一定程度上估计限制。让我们详细看看这些想法。
限制
函数在 x = a 处的极限可以认为是值函数在到达 x = a 时开始取值。点 x = a 可以从任何一侧接近,左侧或右侧。例如,让我们考虑一个函数f(x),那么函数在 x = a 处的极限表示为,
左极限表示为,
![]()
类似地,右手极限表示为,
![]()
- If f(x) gets closer and closer to a specific value L as x approaches a chosen value c from the right, then it is said that the limit of f(x) as x approaches c from the right is L.
- If f(x) gets closer and closer to a specific value L as x approaches a chosen value c from the left, then it is said that the limit of f(x) as x approaches c from the left is L.
- If the limit of f(x) as x approaches c is the same from both the right and the left, then it is said the limit of f(x) as x approaches c is L.
If f(x) never approaches a specific finite value as x approaches c, then we say that the limit does not exist. If f(x) has different right and left limits, then the two-sided limit () does not exist.
可以通过两种方法计算任何函数的极限:
- 代数法
- 图解法
代数方法涉及操纵函数,以便可以轻松计算其极限。图解法简单地涉及制作函数的图形,然后仅通过查看图形就可以估计极限。
从图表估计限制
考虑函数f(x),
f(x) = 3x + 4
这是一个直线方程。让我们看看这个函数在 x = 1 时的极限值是多少。下图显示了这个函数的图形,
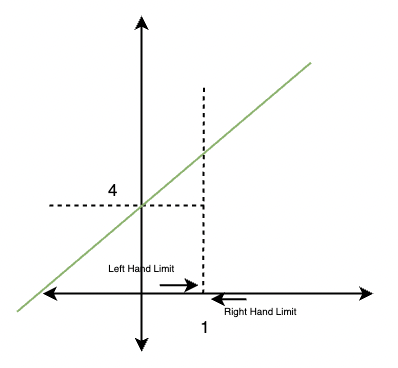
请注意,当我们从 x = 1 的左侧和右侧接近时,函数会移向单个值。这个值称为函数的极限。
无限的限制
有时,某些类型的函数会趋向无穷大。在这种情况下,极限值会上升到无穷大。例如,对于下面给出的函数f(x),
f(x) =
此函数在 x = 0 时的极限,
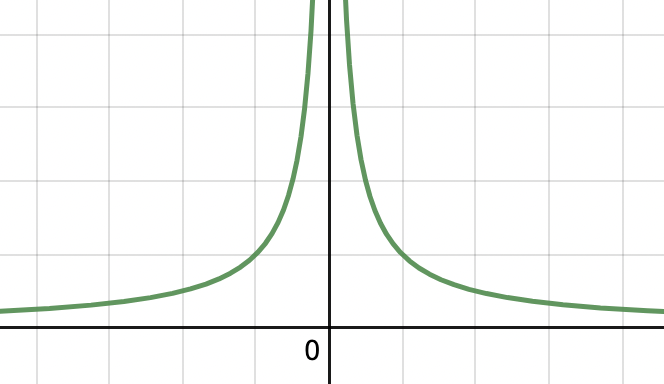
注意图中 x = 0 处的极限是无界的,并且会上升到无穷大。这样的限制称为无界限制。
单方面的限制
有时函数不是连续的,这意味着它们在从两个不同的方面接近时会告诉我们两个不同的限制。例如,最大整数函数本质上是不连续的,在这些不连续处,我们观察到两个不同的值。
f(x) =[x]
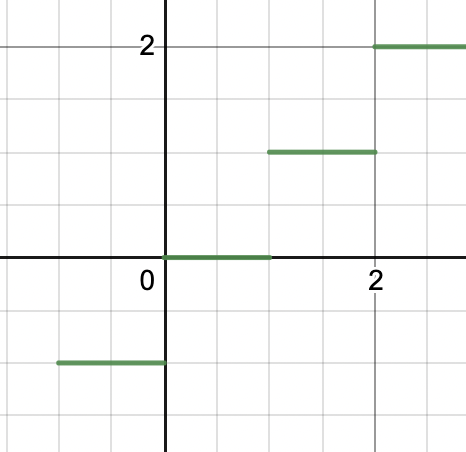
在 x = 1 时,从两个不同的侧面接近时有两个值。在这种情况下,大多数情况下我们被要求从一侧来判断价值。这些被称为单边限制。
限制和图形行为
限制可以说明函数在某个特定点的行为。那么应该如何使用呢?在已知函数不连续的情况下,很难仅使用导数来分析函数的图形。在这种情况下,可以使用限制来找到函数在不连续点处的值。例如,假设我们有一个函数f(x),
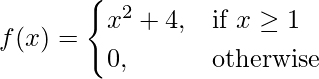
从函数定义中可以看出,这个函数是不连续的,所以导数在这些地方是没有用的。各个部分的图形形状是已知的,让我们计算不连续点 x = 1 处的函数值。
x = 1 处的左极限,
![]()
x = 1 处的右手极限,
⇒ ![]()
⇒ 5
因此,这两个限制的值是不同的。现在我们可以在图表上绘制函数。

示例问题
问题 1:找到![]()
回答:
Graph of x2 is an upward parabola which is centered at origin. The figure below shows the graph of the given function.
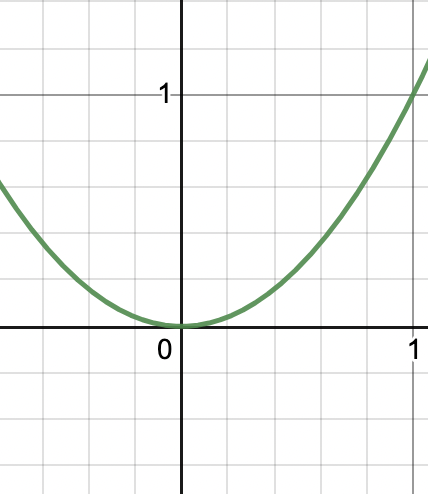
Notice that the function starts moving towards taking a value of 1 as one moves towards the value of x = 1.
Thus,
问题 2:找到![]()
回答:
Graph of log(x) is a saturating function. The figure below shows the graph of the given function.

Notice that the function starts moving towards taking a value of -∞ as one moves towards the value of x = 0. This is an example of unbounded limit mentioned above.
Thus,
问题 3:求函数在 x = 1 处的极限。
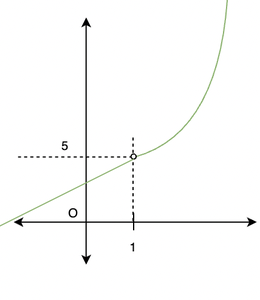
解决方案:
In the graph, the white dot at x = 1 indicates that the function is not defined at x = 1. That means, there should be no value of the function at x =1.
Limit allows us to calculate the values the function was approaching had it been defined at x =1.
So, in this case from graph it can be seen that the function is approaching value of 5.
![]()
问题 4:求函数f(x) = 的极限值![]() 在 x = 0。
在 x = 0。
解决方案:
The figure shows the graph for the given function

The figure makes it clear that there are two limits depending on from which side we are approaching the function.
Left Hand Side Limit:
While approaching zero from the negative side of the origin takes the function to negative infinity.
![]()
Right Hand Side Limit:
While approaching zero from the positive side of the origin takes the function to positive infinity.
问题 5:找到![]()
回答:
Graph of x2 is an upward parabola which is centered at origin. The figure below shows the graph of the given function.
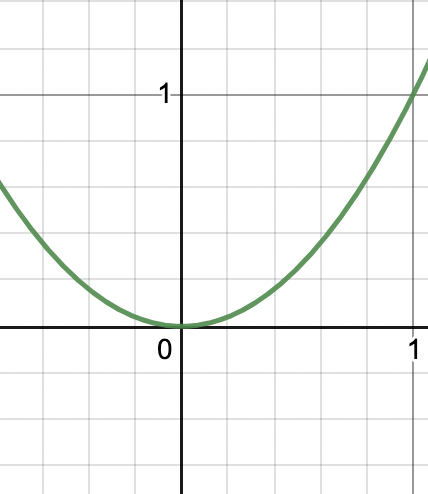
Notice that the function starts moving towards taking a value of 1 as one moves towards the value of x = 1.
Thus,
问题 5:找出给定函数在 x = 0 处的极限,
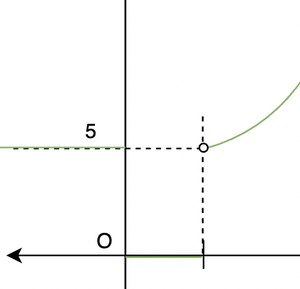
回答:
In the figure, the given function is not continuous at origin. That means there will be two different values of the limit – one from left-hand side and another from right-hand side.
Left- Hand Limit for the function
Right – Hand Limit for the function,