正弦和余弦公式

三角学是数学的一个分支，它与角度评估的概念有关。大多数情况下，这些角度是在直角三角形中测量的。三角函数是通过分别对直角三角形的边和角进行的三角运算得出的。

给定的文章涉及三角学的一个子主题。文章解释了正弦公式和余弦公式的规律，内容还包括对三角比及其三角值相对于各个角度的解释。

什么是三角比？

三角比是以数字形式给出的三角函数的值，并以相对于给定直角三角形的边和角的比率导出。直角三角形有三个边，即。比率所依赖的斜边、垂直和底边。在不同角度下给出不同数值的三角函数的六个基本函数是正弦、余弦、正切、余割、正割和余切。

这些函数在不同角度下的三角函数值如下：

Functions	0°	30°	45°	60°	90°
sinθ	0	1/2	1/√2	√3/2	1
cosθ	1	√3/2	1/√2	1/2	0
tanθ	0	1/√3	1	√3	∞
cotθ	∞	√3	1	1/√3	0
secθ	1	2√3	√2	2	∞
cosecθ	∞	2	√2	2√3	1

正弦定律公式

正弦公式或正弦规则被理解为给定三角形的边和等效角的比率。正弦定律公式通常用于评估斜三角形的未知边或角度。

The mathematical formula of the law of sines is given as

$\frac{a}{sinA}=\frac{b}{sinB}=\frac{c}{sinC}$

where, a, b and c are the sides of the triangle.

在使用正弦规则的三角运算中，我们通常至少考虑三角形的两个角。

正弦公式的推导

一个直角三角形被认为是证明正弦规则的公式。

所以，让我们假设一个三角形 ABC 有各自的边

AB = c

BC = 一个

并且，AC = b

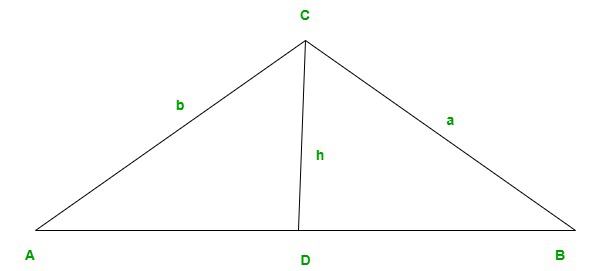
然后，从三角形的底边画一条垂直的CD，即AB。因此，给定三角形的高度将为 CD = h。

现在，绘制的垂线会将三角形分成两个直角三角形，即 CDB 和 CDA。

在这里，要评估 a/b=sinA/SinB

在 CDA 中

=>sinA=h/b

而且，在国开行

=>sinB=h/a

然后，

=>sinA/sinB=(h/b)/(h/a)

所以，

=> SinA/sinB=a/b证明。

类似地，其他函数如 sinB/sinC = b/c 也可以用同样的方法证明。

正弦规则的其他一些公式

• a:b:c = sinA:sinB:sinC
• a/b = sinA/sinB
• b/c = sinB/sinC

余弦公式定律

余弦定律或余弦规则是与给定三角形的边长和角余弦相关的表达式。余弦定律指出：“三角形任何一边的平方等于另一边的平方和之差，再乘以其他边的乘积和它们之间的余弦角。”

在数学上，余弦定律表示为

a² = b²+ c² – 2bc. cosA

b² = c² + a² – 2ca. cosB

c² = a² + b² – 2ab. cosC

where,

a,b, and c are the lengths of the sides

And, A, B, and C are the angles.

余弦定律的推导

让我们假设一个三角形 ABC 有它的各个边

AB = c

BC = 一个

并且，AC = b

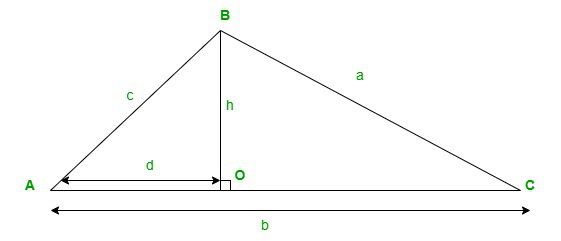
然后，从三角形的底边画一条垂线 OB，即 AC。因此，给定三角形的高度将为 OB=h。

现在，绘制的垂直线会将三角形分成两个直角三角形，分别是 ABO 和 BOC。

现在，在 ABO

sinA=BO/AB=h/c…………..(i)

和，

cosA=AO/AB=d/c ………….(ii)

从方程 (i) 和 (ii) 我们得到 h 和 d 的值，

h=c(sinA)

d=c(cosA)

通过使用 BOC 中的毕达哥拉斯定理

=>a ² =h ² +(bd) ²

代入 h 和 d 的值，我们得到

=>a ² =c ² sin ² A+b ² +c ² cos ² A-2bc。 COSA

=>a ² =c ² (sin ² A+cos ² A)+b ² -2bc.cosA

=> a ² =c ² +b ² -2bc。 cosA证明。

其他表达方式类似

b=c+a-2ca.cosB 和

c=a+b-2ab.cosC 也可以用同样的方法证明。

示例问题

问题 1：找到一个三角形的缺失边，它的两条边分别为 12 厘米和 8 厘米，它们之间的夹角为 60 度。

解决方案：

Given

Let the two sides b and c be 12cm and 8cm respectively and a be the missing side.

The angle between b and c is 60°

Now, by using the law of cosine formula

a²=b²+c²-2bc. cosA

=>a²=(12)²+(8)²-2(12)(8)cos60°

=>a²=144+64-192×0.5

=>a²=208-96

=>a²=112

=>a=10.58cm

问题 2：找到一个三角形的缺失边，它的两条边分别为 25 厘米和 10 厘米，它们之间的夹角为 30 度。

解决方案：

Given

Let the two sides b and c be 25cm and 10cm respectively and a be the missing side.

The angle between b and c is 30°.

Now, by using the law of cosine formula

a²=b²+c²-2bc. cosA

=>a²=(25)²+(10)²-2(25)(10)cos30°

=>a²=625+100-500×0.86

=>a²=725-430

=>a²=295

=>a=17.17cm

问题 3：找到一个三角形的缺失边，它的两条边分别为 21 厘米和 14 厘米，它们之间的夹角为 45 度。

解决方案：

Given

Let the two sides b and c be 21cm and 14cm respectively and a be the missing side.

The angle between b and c is 45°.

Now, by using the law of cosine formula

a²=b²+c²-2bc. cosA

=>a²=(21)²+(14)²-2(21)(14)cos45°

=>a²=441+196-588×.707

=>a²=637-415.7

=>a²=221.3

=>a=14.87cm

问题4：求a边的值，如果两个角为∠A=65°，∠B=40°，b=12cm。

解决方案：

Given

 ∠A=65° and ∠B=40° 

And, b=12cm

Now,

a/sinA=b/sinB

=>a/sin65°=12/sin40°

=>a/0.906=12/0.642

=>a=16.93cm.

问题5：求a边的值，如果两个角为∠A=72°，∠B=60°，b=6cm。

解决方案：

Given

∠A=72° and ∠B=60°

And, b=6cm

Now,

a/sinA=b/sinB

=>a/sin72°=6/sin60°

=>a/0.951=6/0.86

=>a=6.41cm.

问题6：求a边的值，如果两个角为∠A=48°，∠B=35°，b=16cm。

解决方案：

Given

∠A=48° and ∠B=35°

And, b=16cm

Now,

a/sinA=b/sinB

=>a/sin48°=16/sin35°

=>a/0.743=16/0.57

=>a=20.85cm.