📌  相关文章
📜  带电粒子在磁场中的运动

📅  最后修改于: 2022-05-13 01:57:37.494000             🧑  作者: Mango

带电粒子在磁场中的运动

这已经了解了电场和磁场的相互作用，以及在电场和磁场同时存在的情况下带电粒子的运动。我们还推导出了作用在带电粒子上的力的关系，在这种情况下由洛伦兹力给出。我们还了解了当载流导电棒暴露在磁场中时作用在其上的磁力。

但是，当带电粒子在磁场存在的情况下行进时会发生什么？我们如何定义这样一个粒子的轨迹？这将在本文中详细介绍。

当力作用于粒子时，如果力的一个分量指向粒子的运动方向，则称其产生功。在所讨论的情况下，当我们有一个带电荷的粒子在大小为 B 的均匀磁场中传播时，磁力垂直于粒子的运动。在这种情况下，我们声称磁力对粒子不起作用因此看不到粒子速度的变化。当粒子的速度 v 垂直于磁场方向时，我们可以写成，

F = q (v × B)

在这种情况下，磁力指向物体的圆周运动中心并起到向心力的作用。因此，如果 v 和 B 是垂线，则粒子描述了一个圆。在其他情况下，如果在磁场 B 的方向上存在速度分量，它的大小在运动过程中保持不变，因为它不受磁场的影响。此外，如前所述，由速度的垂直分量引起的运动本质上是圆形的。

带电粒子在磁场中的圆周运动

当带电粒子在磁场中移动时，它会受到力。如果这个场在带电粒子的运动中是均匀的，会发生什么？粒子走哪条路线？在本节中，我们将研究带电粒子的圆周运动以及带电粒子进入磁场时发生的其他运动。

最简单的例子是带电粒子垂直于均匀运动，如下图所示。如果场在真空中，则磁场是运动的决定因素。磁场中的带电粒子会沿着弯曲的路线行进，因为磁力垂直于运动方向。

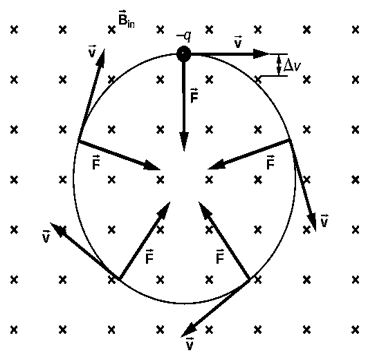
在磁场垂直于纸的区域中，带负电的粒子在纸的平面内行进。

粒子跟随这条弯曲的路径，直到它形成一个完整的圆圈。另一种思考方式是磁力始终垂直于速度，因此它对带电粒子不起作用。结果，粒子的动能和速度保持不变。速度不受影响，但方向是。

螺旋运动

当速度矢量不垂直于磁场矢量时，就会发生螺旋运动。

如图所示，当带电粒子垂直于均匀 B 场行进时，会出现最简单的情况。 （如果这发生在真空中，磁场是影响运动的最重要元素。）在这种情况下，磁力（洛伦兹力）提供了向心力，因为

Fc = mv 2 / r

因为，这里 sin θ = 1

那么，磁力：

F = qvB

现在，如果洛伦兹磁力提供向心力，则这些力必须等于：

qvB = mv 2 / r

将上面的表达式求解为 r，

r = mv / qB

质量为 m 且电荷为 q 的带电粒子以垂直于强度为 B 的磁场的速度 v 行进的路径的曲率半径用 r 表示，也称为回旋半径或回旋加速器半径。换句话说，它是在均匀磁场存在下带电粒子圆周运动的半径。

如果速度不垂直于磁场，则 v 是速度的垂直分量。因为平行于场的运动的磁力为 0，所以平行于场的速度分量不受影响。在后续关于螺旋运动的部分中，我们将研究这种情况的含义。

当粒子由于均匀磁场而经历圆周运动时，就会发生回旋共振。这个词来源于被称为回旋加速器的循环粒子加速器的名称，该加速器在 .

回旋加速器频率定义为粒子每秒围绕循环回路完成的循环次数，可以通过求解上述 v 并代入循环频率来计算，使得

f = v / 2πr

要么

f = qB / 2πm

因此，回旋加速器频率很容易以弧度每秒表示为：

ω = qB / m

磁镜

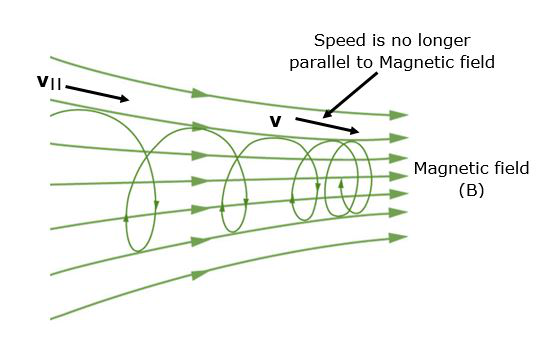
磁镜和螺旋运动

如果 v 表示粒子的旋转频率。因此，一转的时间跨度可表示为：

一转的时间， T = 2π / ω = 1 / v

一个粒子的间距是它在一个旋转中沿着磁场方向移动的距离。然后：

间距， p = v || T = 2πm v || /qB

哪里 v ||是平行于磁场的速度。

示例问题

问题 1：描述如果射频 (r f ) 场的频率加倍，带电粒子将如何在回旋加速器中移动。

解决方案：

问题 2：在质子、中子和电子中，当以垂直于磁场的相同速度推进时，哪个粒子可能具有最低的旋转频率？

解决方案：

问题 3：当质子在均匀磁场中运动时，它的速度会发生变化，但动能不会发生变化。为什么？

解决方案：

问题4：磁场可以加速带电粒子吗？有没有可能提高它的速度？

解决方案：

问题 5：什么是洛伦兹力？解释。

解决方案：