评估定积分

整合，顾名思义就是用来整合一些东西。在数学中，积分是用于积分函数的方法。积分的另一个词可以是求和，用于总结整个函数或以图形方式，用于找到曲线函数下的面积。众所周知，积分与微分相反，微分是将函数分解为较小函数的过程，而积分是将较小的位相加以获得曲线下的整个面积。

定积分

有两种可能的积分方式——定积分和不定积分。定积分用于给定有限制或边界的区域，即曲线是有限的，因此曲线下的面积也应是有限的，而不定积分用于没有上限或下限的函数，函数是本质上是无限的，因此，不定函数的上限和下限是 +∞ 和 -∞。

当函数f 在闭区间 [a, b] 中连续时，函数f(x) 从 a 到 b 的定积分。

$\int^{b}_{a}f(x)dx = lim_{n \to \infty} \sum_{i=1}^{n}f(x^*_i)\Delta x_i = F(b) - F(a)$ 

$\int_{a}^{b}f(x)dx=F(b)-F(a)$  

Where, a and b are the lower and upper limits.

F(x)是f(x)的积分，若对f(x)求微分，则得到F(x)。因此，可以说 F 是 f 的反导数。定积分也称为黎曼积分。

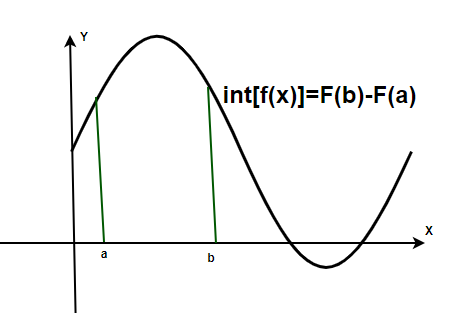
定积分的求值——性质

在数学中，简单的求和和加法很容易，但在评估复杂积分时，简单的计算和加法是不够的，因此需要积分。在评估定积分时，有时计算变得过于繁琐和复杂，因此为了使计算相对容易，提出了一些经验证明的性质。

属性一： $\int^{b}_{a}f(x)dx = \int^{b}_{a}f(t)dt$ 

This property can be proved by simply substituting the value of “t” in place of “x”.

属性 2： $\int^{b}_{a}f(x)dx = - \int^{a}_{b}f(x)dx$

Proof: Let F(x) be anti-derivative of the integral $\int f(x)dx$. 

So, we know 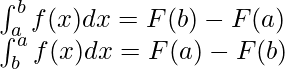$\int^{b}_{a} f(x)dx = F(b) - F(a) \\ \int^{a}_{b} f(x)dx = F(a) - F(b) \\$

Comparing these two equations, we can deduce that $\int^{b}_{a}f(x)dx = - \int^{a}_{b}f(x)dx$

Hence, Proved. 

属性 3： $\int^{b}_{a}f(x)dx = \int^{c}_{a}f(x)dx + \int_{c}^{b}f(x)dx$

Proof: $\int^{b}_{a}f(x)dx = F(b) - F(a) \\ \int^{c}_{a}f(x)dx = F(c) - F(a) \\ \int^{b}_{c}f(x)dx = F(b) - F(c) \\$

Adding the above two equations will give us the first equation, 

$\int^{c}_{a}f(x)dx + \int^{b}_{c}f(x)dx = F(c) - F(a) \\ + F(b) - F(c) \\ \int^{c}_{a}f(x)dx + \int^{b}_{c}f(x)dx = - F(a) \\ + F(b) \\ \int^{c}_{a}f(x)dx + \int^{b}_{c}f(x)dx = F(b) - F(a) \\ \int^{c}_{a}f(x)dx + \int^{b}_{c}f(x)dx = \int^{b}_{a}f(x)dx \\$

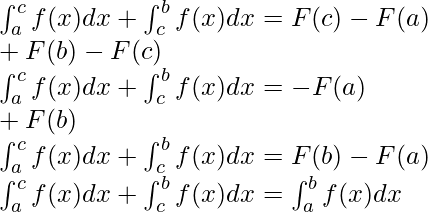
属性 4： $\int^{b}_{a}f(x)dx = \int^{b}_{a}f(a + b - x)dx$

Proof: Let’s assume t = a + b -x. Then dt = -dx, When x = a, t = b and wh

en x = b, t = a. 

Therefore, 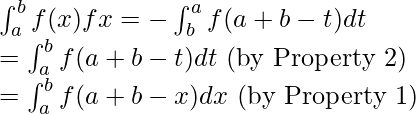$\int^{b}_{a}f(x)fx = -\int^{a}_{b}f(a+b-t)dt \\ = \int^{b}_{a}f(a + b -t)dt \text{ (by Property 2)} \\ = \int^{b}_{a}f(a + b -x)dx \text{ (by Property 1)} \\$

属性 5： $\int^{a}_{0}f(x)dx = \int^{a}_{0}f(a-x)dx$ 

Proof: This property is a particular case property 4, so it can also be proved that way. 

属性 6： $\int_{0}^{2a}f(x)dx = \int_{0}^{a}f(x)dx + \int_{0}^{a}f(2a - x)dx$

Proof: Using Property 3, we can write this as, 

$\int^{2a}_{0}f(x)dx = \int^{a}_{0}f(x)dx + \int_{a}^{2a}f(x)dx \\$

Let t = 2a  x in the second integral on the right-hand side. Then dt = – dx. When x = a, t = a and when x = 2a, t = 0. Also, x = 2a – t. Therefore, the second integral becomes

$\int^{2a}_{0}f(x)dx = -\int^{0}_{a}f(2a-t)dt = \int^{a}_{0}f(2a-t)dt = \int^{a}_{0}f(2a-x)dx$

Hence, \$\int^{2a}_{0}f(x)dx = \int^{a}_{0}f(x)dx + \int^{a}_{0}f(2a-x)dx$

属性 7： $\int^{2a}_{0} f(x)dx = \begin{cases} 2\int^{a}_{0}f(x)dx,& \text{if } f(2a-x)=f(x)\\ 0, & \text{} f(2a -x) = -f(x) \end{cases}$

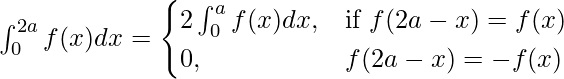
Proof: Using property 6, we have $\int^{2a}_{0}f(x)dx = \int^{a}_{0}f(x)dx + \int^{a}_{0}f(2a-x)dx$

Now, if f(2a – x) = f(x) then this equation becomes, 

$\int^{2a}_{0}f(x)dx = \int^{a}_{0}f(x)dx + \int^{a}_{0}f(x)dx = 2\int^{a}_{0}f(x)dx$

And if f(2a – x) = – f (x), then that equation becomes

$\int^{2a}_{0}f(x)dx = \int^{a}_{0}f(x)dx - \int^{a}_{0}f(x)dx = 0$

属性 8： $\int^{a}_{-a}f(x)dx = 2\int^{a}_{0}f(x)dx$如果它是偶函数，即如果 f(-x) = f(x)。

属性 9： $\int^{a}_{-a}f(x)dx = 0$ ，如果是奇函数，即f(-x) = -f(x)。

Proof: Using Property 3, we have, 

$\int^{a}_{-a}f(x)dx = \int_{-a}^{0}f(x)dx + \int^{a}_{0}f(x)dx$

Let t = – x in the first integral on the right-hand side. 

dt = – dx. When x = – a, t = a and when x = 0, t = 0. Also, x = – t.

So, we can rewrite the above expression as, 

$\int^{a}_{-a}f(x)dx = -\int_{a}^{0}f(-t)dt + \int^{a}_{0}f(x)dx \\ = \int^{a}_{0}f(-x)dx + \int^{a}_{0}f(x)dx$

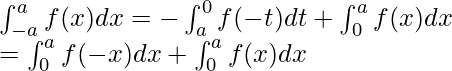
1. Now, if f is an even function i.e f(-x) = f(x), 

$\int^{a}_{-a}f(x)dx = \int^{a}_{0}f(-x)dx + \int^{a}_{0}f(x)dx \\ = 2\int^{a}_{0}f(x)dx$

2. If f is an odd function i.e f (–x) = – f(x). 

$\int^{a}_{-a}f(x)dx = \int^{a}_{0}f(-x)dx + \int^{a}_{0}f(x)dx \\ = 0$

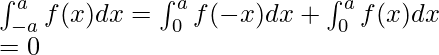
示例问题

问题1：评估， $\int_{1}^{3}x^2dx$

解决方案：

Integrating,

$\int_{1}^{3}x^2dx=[\frac{x^3}{3}]_{1}^{3} \\=1/3[(27)-(1)] \\=26/3 \\=8.66$

问题2：评估， $\int_{0}^{\pi/2}sinxdx$

解决方案：

Integrating the above given function,

$\int_{0}^{\pi/2}sinxdx=[cosx]_{0}^{\pi/2} \\=[cos\pi/2-cos0] \\=0-1 \\=-1$

问题3：评估， $\int_{-2}^{1}e^yxdx$

解决方案：

Integrating the function,

$\int_{-2}^{1}e^yxdx=e^y[\frac{x^2}{2}] \\e^y[\frac{x^2}{2}]_{-2}^{1} \\\frac{e^y}{2}[1-(-4)] \\\frac{e^y}{2}(5)$

问题 4：鉴于， $\int^{10}_{2}f(x)dx = 20, \int^{20}_{10}f(x)dx = -10$ .找到价值$\int^{20}_{2}f(x)dx$ .

解决方案：

 For solving this question, we will be using Property 3. 

$\int^{20}_{2}f(x)dx = \int^{20}_{10}f(x)dx + \int^{10}_{2}f(x)dx$

Now, we just have to plug in the value of the given definite integrals. 

$\int^{20}_{2}f(x)dx = \int^{20}_{10}f(x)dx + \int^{10}_{2}f(x)dx \\ = -10 + 20 \\ = 10$

问题 5：给定 f(x) = x ³ 。找到价值$\int^{-3}_{3}x^3dx$ .

解决方案：

 Now this answer can be calculated through usual integration method, it is fairly easy. But we can think of a property which can be used here to completely save your calculation and time. 

Property 8 and Property 9 can be used depending upon which kind of function f(x) = x³ is, even or odd. Let’s check whether f(x) is even or odd.

For an even function, f(-x) = f(x) and for an odd function f(-x) = -f(x). 

Here in our case, f(-x) = (-x)³ = -x³ = -f(x). So it is an odd function. 

Thus, Property 9 is applicable here, and through property 9 we can say that. 

$\int^{3}_{-3}f(x)dx = 0$

问题 6：给定， $\int^{2}_{10}f(x)dx = 20, \int^{20}_{10}f(x)dx = -10$ .找到价值$\int^{20}_{2}f(x)dx$ .

解决方案：

This question is similar to what we solved a little while ago, It also requires application of Property 3. But we need to do something about $\int^{2}_{10}f(x)dx = 20$. 

This problem can be solved by the application of Property 2. 

Using property 2, 

$\int^{2}_{10}f(x)dx = 20 \\ \int^{10}_{2}f(x)dx = -20$

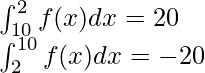
So, now plugging the values, 

$\int^{20}_{2}f(x)dx = \int^{20}_{10}f(x)dx + \int^{10}_{2}f(x)dx \\ = -20 -10 \\ = -30$

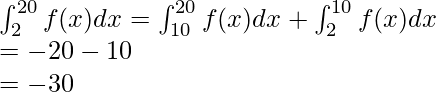
问题 7：评估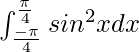， $\int^{\frac{\pi}{4}}_{\frac{-\pi}{4}}sin^2xdx$

解决方案：

 In previous questions we checked for an even or odd function. By a similar check, we can say that sin²x is an even function. 

So by the use property 8, we can rewrite it as, 

$\int^{\frac{\pi}{4}}_{\frac{-\pi}{4}}sin^2xdx = 2\int^{\frac{\pi}{4}}_{0}sin^2xdx \\ = 2\int^{\frac{\pi}{4}}_{0}\frac{1-cos2x}{2}dx \\ = \int^{\frac{\pi}{4}}_{0}(1-cos2x)dx = [x - \frac{1}{2}sin2x]^{\frac{\pi}{4}}_{0}\\ = \frac{\pi}{4} - \frac{1}{2}sin(\frac{\pi}{2}) = \frac{\pi}{4} - \frac{1}{2}$

问题 8：评估， $\int^{10}_{10}(x^4 + sinx)dx \\$

解决方案：

 This expression contains two terms. We know,

Sin(x) is an odd function and x⁴ is an even function. 

So, 

$\int^{10}_{-10}(x^4 + sinx)dx \\ = \int^{10}_{-10}x^4dx + \int^{10}_{-10}sinxdx \\ = 2\int^{10}_{0}x^4dx + 0 \\ = 2[\frac{x^5}{5}]^{10}_{0} \\ = 2(\frac{100000}{5}) \\ = 40000$