不正确的积分

从几何上讲，积分是计算曲线下面积或体积的方法。这些方法允许数学家计算任意复杂曲线下的面积。这些类型的积分称为定积分。定积分是建立在不定积分的思想之上的。这些不定积分只不过是函数的反导数。反导是微分的逆。让我们详细看看这些想法。

什么是不正确的积分？

不适当的积分是其中一个或两个边界都在无穷大或积分区间在积分区间内具有垂直渐近线的定积分。计算无穷大的面积似乎是一个棘手的问题，但通过一些巧妙的操作，这些问题是可以解决的。让我们考虑一个函数f(x)，那么在 x = a 和 x = b 之间由 x 轴包围的曲线下面积表示为，

$\int^{b}_{a}f(x)dx$

由于这里的两个极限都是有限的，所以这称为真积分。具有无限界的真积分将表示为，

$\int^{\infty}_{a}f(x)dx$要么$\int^{a}_{-\infty}f(x)dx$

考虑一个例子以获得更好的理解。

示例：计算以下定积分。

$\int^{\infty}_{1}\frac{1}{x^2}dx$

解决方案：

The graph of this function is shown the figure below. The goal is to calculate the mentioned area. Notice that the area is not diverging as the function is going towards zero asymptotically. 

$\int^{\infty}_{1}\frac{1}{x^2}dx$

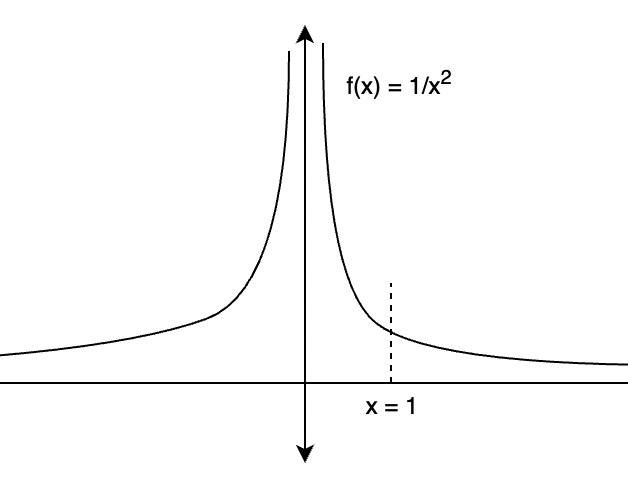
This can be re-written as, 

$\lim_{n \to \infty}\int^{n}_{1}\frac{1}{x^2}dx$

Now this is just a definite integral, to solve this second part of fundamental theorem of calculus can be used.

 $\lim_{n \to \infty}[-\frac{1}{x}]^{n}_{1} \\ = \lim_{n \to \infty}[1 - \frac{1}{n}] \\ = 1$

有时积分的极限范围都是无穷大。这样的积分称为具有两个无限界的不正确积分。

$\int^{\infty}_{-\infty}f(x)dx$要么$\int^{\infty}_{-\infty}f(x)dx$

发散不正确积分

在前面的函数中，计算到无穷大的区域的极限是有限的。但通常在某些情况下，积分不会收敛到有限值。直观地说，这意味着曲线下包围的区域不是有限的。考虑此类案例的示例，以更好地理解此类积分。

示例：计算以下积分。

$\int^{\infty}_{1}\frac{1}{x}dx$

解决方案：

The graph of this function is given below. 

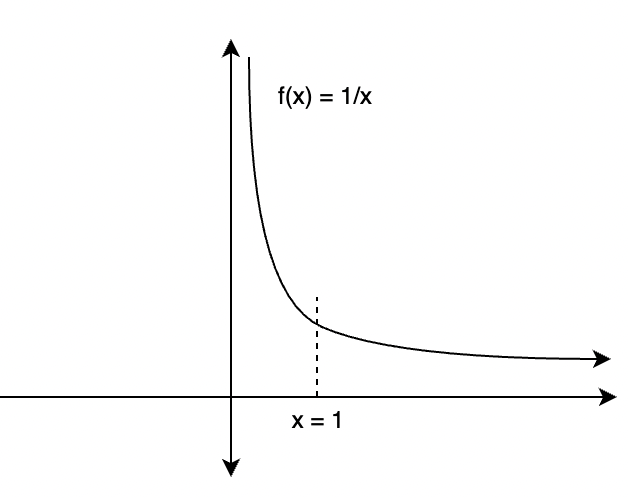
Let’s compute the area under this curve using the same method as above.

$\int^{\infty}_{1}\frac{1}{x}dx$

Rewriting the given integral. 

$\lim_{n \to \infty}\int^{n}_{1}\frac{1}{x}dx$

Now this is again just a definite integral, to solve this second part of fundamental theorem of calculus can be used.

$\lim_{n \to \infty}[ln(x)]^{n}_{1} \\ = \lim_{n \to \infty}[ln(n) - ln(1)] \\ = \lim_{n \to \infty} ln(n) = \infty$

Since this limit diverges. The area under the curve is infinite. 

让我们看看这些概念上的一些问题。

示例问题

问题 1：计算以下定积分。

$\int^{\infty}_{1}\frac{1}{x^3}dx$

解决方案：

$\int^{\infty}_{1}\frac{1}{x^3}dx$

This can be re-written as, 

$\lim_{n \to \infty}\int^{n}_{1}\frac{1}{x^3}dx$

Now this is just a definite integral, to solve this second part of the fundamental theorem of calculus can be used.

 $\lim_{n \to \infty}[-\frac{1}{2x^2}]^{n}_{1} \\ = \lim_{n \to \infty}[\frac{1}{2} - \frac{1}{n}] \\ = \frac{1}{2}$

问题 2：计算以下定积分。

$\int^{\infty}_{2}\frac{x + 1}{x^2}dx$

解决方案：

$\int^{\infty}_{2}\frac{x + 1}{x^2}dx$

This can be re-written as, 

$\lim_{n \to \infty}\int^{n}_{2}\frac{x + 1}{x^2}dx$

Now this is just a definite integral, to solve this second part of fundamental theorem of calculus can be used.

 $\lim_{n \to \infty}\int^{n}_{2}\frac{x + 1}{x^2}dx \\ = \lim_{n \to \infty}\int^{n}_{2}\frac{1}{x} + \frac{1}{x^2}dx \\ = \lim_{n \to \infty}[ln(x)]^n_2 + [\frac{-1}{x}]^n_{2} \\ = \lim_{n \to \infty}[ln(\infty) - ln(2)] + 1$

This limit evaluates to infinity. Thus, the area under the curve is infinite. 

问题 3：计算以下定积分。

$\int^{\infty}_{1}\frac{x + 1}{x^3}dx$

解决方案：

$\int^{\infty}_{1}\frac{x + 1}{x^3}dx$

This can be re-written as, 

$\lim_{n \to \infty}\int^{n}_{1}\frac{x + 1}{x^3}dx$

Now this is just a definite integral, to solve this second part of the fundamental theorem of calculus can be used.

 $\lim_{n \to \infty}\int^{n}_{1}\frac{x + 1}{x^3}dx \\ = \lim_{n \to \infty}\int^{n}_{1}\frac{1}{x^2} + \frac{1}{x^3}dx \\ = \lim_{n \to \infty}[\frac{-1}{x}]^n_1 + [\frac{-1}{2x^2}]^n_{1} \\ = \lim_{n \to \infty}[1 - \frac{1}{n}] + [\frac{1}{2} - \frac{1}{n}] \\ = 1 + \frac{1}{2} \\ = \frac{3}{2}$

This limit evaluates to infinity. Thus, the area under the curve is infinite. 

问题 4：计算以下定积分。

$\int^{\infty}_{1}\frac{1}{\sqrt{x}}dx$

解决方案：

$\int^{\infty}_{1}\frac{1}{\sqrt{x}}dx$

This can be re-written as, 

$\lim_{n \to \infty}\int^{n}_{1}\frac{1}{\sqrt{x}}dx$

Now this is just a definite integral, to solve this second part of fundamental theorem of calculus can be used.

 $\lim_{n \to \infty}[\frac{\sqrt{x}}{2}]^{n}_{1} \\ = \lim_{n \to \infty}[\frac{\sqrt{n}}{2} - \frac{1}{2}] \\ = \infty$

This area cannot be calculated. This is infinite. 

问题 5：计算以下定积分。

$\int^{\infty}_{1}\frac{1}{\sqrt{x + 3}}dx$

解决方案：

$\int^{\infty}_{1}\frac{1}{\sqrt{x + 3}}dx$

This can be re-written as, 

$\lim_{n \to \infty}\int^{n}_{1}\frac{1}{\sqrt{x + 3}}dx$

Now this is just a definite integral, to solve this second part of fundamental theorem of calculus can be used.

 $\lim_{n \to \infty}[\frac{\sqrt{x + 3}}{2}]^{n}_{1} \\ = \lim_{n \to \infty}[\frac{\sqrt{n + 3}}{2} - \frac{1}{2}] \\ = \infty$

This area cannot be calculated. This is infinite. 

问题 6：确定这个不正确的积分是收敛的还是发散的。

$\int^{\infty}_{1}cos(x)dx$

解决方案：

$\int^{\infty}_{1}cos(x)dx$

This can be re-written as, 

$\lim_{n \to \infty}\int^{n}_{1}cos(x)dx$

Now this is just a definite integral, to solve this second part of fundamental theorem of calculus can be used.

 $\lim_{n \to \infty}[sin(x)]^{n}_{1} \\ = \lim_{n \to \infty}[sin(n)- sin(1)]$

The limit is not defined, thus this integral is divergent. 

问题 7：确定这个不正确的积分是收敛的还是发散的。

$\int^{\infty}_{1}e^{-x} dx$

解决方案：

$\int^{\infty}_{1}e^{-x} dx$

This can be re-written as, 

$\lim_{n \to \infty}\int^{n}_{1}e^{-x}dx$

Now this is just a definite integral, to solve this second part of fundamental theorem of calculus can be used.

 $\lim_{n \to \infty}[-e^{-x}]^{n}_{1} \\ = \lim_{n \to \infty}[e- e^{-n}] \\ = e$