定积分面积

积分是微积分的一个组成部分。它们代表求和，对于不像标准函数那么简单的函数，积分帮助我们计算总和及其面积，并使我们能够灵活地使用我们想要使用的任何类型的函数。标准函数的面积是已知的，要保存和记住每种函数面积的公式并不容易。积分为此提供了普遍性，并提供了一种为任何一般函数计算这些东西的方法。定积分用于计算曲线下的面积。让我们详细研究一下这个概念。

定积分

定积分被定义为有限制的总和。这些积分的界限定义为它们的边界，它们计算给定函数的总和。这些限制称为开始值和结束值，定义为 [a, b] 其中 a 称为下限，b 称为总和的上限。定积分的计算方法是先计算 a 处的不定积分，然后计算 b 处的不定积分，然后将两者相减。

Given a function f(x) which is continuous between [a, b], this interval is divided into n sub-intervals of width $\Delta x$ and from each interval a point is chosen x^*_i. Then the value of the definite integral of the function f(x) from a to b is, 

$\int^{b}_{a}f(x)dx = \lim_{n \to \infty} \sum^{n}_{i = 1}f(x^{*}_{i})\Delta x$

a and b are collectively called interval of integration. This is calculated using the following expression, 

Let’s say F(x) = $\int f(x)dx$

$\int^{b}_{a}f(x)dx = F(b) - F(a)$

下图显示了函数f(x) 在区间 a 和 b 之间的定积分。

定积分遵循总和属性，使我们能够简化计算。

定积分的性质

Property 1: Limits of any definite integral can be interchanged, a minus sign is added while interchanging the limits. 

$\int^{b}_{a}f(x)dx = -\int^{a}_{b}f(x)dx$

Property 2: If the upper limits and lower limits are equal, then the value of the integral is zero. 

$\int^{a}_{a}f(x)dx = 0$

Property 3: When a function is multiplied with a constant, its integral is also multiplied by that constant. 

$\int^{b}_{a}cf(x)dx = c\int^{b}_{a}f(x)dx$

Property 4: Definite integrals can be broken down across sums and differences. 

$\int^{b}_{a}(f(x) \pm g(x))dx = \int^{b}_{a}f(x)dx \pm \int^{b}_{a}g(x)dx$

Property 5: The intervals of integrals can be broken down. 

$\int^{b}_{a}f(x)dx = \int^{c}_{a}f(x)dx + \int^{b}_{c}f(x)dx$

定积分为面积

计算曲线所包围的面积是积分最重要的应用之一。定积分允许我们计算固定点 x = a 和可变点 x 之间的任何曲线 f(x) 所包围的面积。

现在由于定积分计算的是非常小的矩形条的总和，如上图所示，它们可以用来计算曲线下的面积。在这种情况下，曲线下的面积将由下式给出，

$\int^{b}_{a}f(x)dx = [F(x)]^{b}_{a} = F(b) - F(a)$

其中，F(x) = $\int f(x)dx$

x 轴上方曲线所包围的区域

考虑下图中给出的函数。该函数完全位于 x 轴上方。我们感兴趣的是计算该曲线与点 x = a 和 x = b 之间的 x 轴之间的面积。这种情况很简单，只需要我们计算曲线下的面积

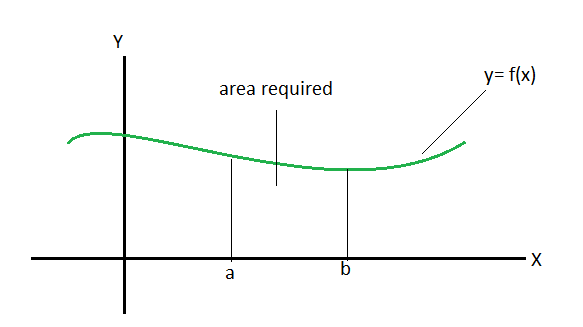
因此，当曲线完全位于 x 轴上方时，面积变为，

$\int^{b}_{a}f(x)dx = F(b) - F(a)$

曲线所包围的区域不完全在x 轴上方

在下图中，曲线的某些部分位于 x 轴下方。函数是 f(x) = -x ² + 1

感兴趣的区域在 x 轴下方，这部分的积分评估导致我们进入负区域。这是不可能的，因为面积不能为负。如果一个函数在某些点上同时位于 x 轴上方和 x 轴下方。那么我们在计算面积时需要特别注意，因为如果把它们一起计算，正负面积会相互抵消，我们将无法得到正确的面积值。因此，在这种情况下，必须打破限制，以便将两个积分都分离出来，并且应该将它们加上它们的绝对值。

假设一个函数由 f(x) 给出，函数位于 [0,3] 之间的 x 轴上方和 (3,∞) 之间的 x 轴下方。目标是计算[0,5]之间的函数所包围的面积。

所以，A = $\int^{5}_{0}f(x)dx$

⇒A = $\int^{3}_{0}f(x)dx + |\int^{5}_{3}f(x)dx |$

让我们看一些示例问题

示例问题

问题 1：计算函数f(x) 和 x 轴在 x = 0 到 x = 1 之间所包围的面积。

f(x) = 3

回答：

$\int^{1}_{0} f(x)dx$

⇒$\int^{3}_{0}(3 )dx$

⇒$3[3x]^{3}_{0}$

⇒$9[x]^{3}_{0}$

⇒ $9(3)$

⇒ 27

问题 2：计算函数f(x) 和 x 轴在 x = 0 到 x = 3 之间的面积。

f(x) = x ² + 2

回答：

$\int^{3}_{0} f(x)dx$

⇒$\int^{3}_{0}(x^2 + 2)dx$

⇒$\int^{3}_{0}x^2dx + \int^{3}_{0}2dx$

⇒$[\frac{x^3}{3}]^{3}_{0} +[2x]^{3}_{0}$

⇒$[\frac{3^3}{3} - 0] +[2(3) - 0]$

⇒9 + 6

⇒ 15 

问题 3：计算函数f(x) 和 x 轴在 x = 0 到 x = 3 之间的面积。

f(x) = 3x

回答：

$\int^{3}_{0} f(x)dx$

⇒$\int^{3}_{0}(3x )dx$

⇒$3[\frac{x^2}{2}]^{3}_{0}$

⇒$3[\frac{3^2}{2} - 0]$

⇒ $\frac{3^3}{2}$

⇒ $\frac{27}{2}$

问题4：计算函数f(x)和x轴在x=0到x=之间的面积$\frac{\pi}{2}$ .

f(x) = sin(x) + 2

回答：

$\int^{\frac{\pi}{2}}_{0} f(x)dx$

⇒$\int^{\frac{\pi}{2}}_{0}(sin(x) + 2)dx$

⇒$\int^{\frac{\pi}{2}}_{0}sin(x)dx + \int^{\frac{\pi}{2}}_{0}2dx$

⇒$[-cos(x)]^{\frac{\pi}{2}}_{0} + [2x]^{\frac{\pi}{2}}_{0}$

⇒$1 + 2(\frac{\pi}{2} - 0)$

⇒$1 +\pi$

问题 5：计算函数f(x) 和 x 轴在 x = 0 到 x = 1 之间所包围的面积。

f(x) = -e ^x

回答：

$\int^{1}_{0} -e^xdx$

Now this function lies completely below the x-axis, so only the magnitude of the area will be considered, the sign will be ignored. 

⇒$\int^{1}_{0} -e^xdx$

⇒$[-e^x]^{1}_{0}$

⇒$[e^x]^{0}_{1}$

⇒1 – e

Only the magnitude of this area will be considered. 

问题6：通过函数f(x)计算[0,3]之间的面积。

$f(x)= \begin{cases} -3x,& \text{if } x\geq 1\\ x, & \text{otherwise} \end{cases}$

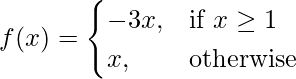
解决方案：

It is obvious from the definition that the function becomes negative after x >=1. 

So, the integral must be broken down at x = 1. 

$\int^{3}_{0}f(x)dx$

⇒$\int^{1}_{0}f(x)dx + \int^{3}_{1}f(x)dx$

⇒$\int^{1}_{0}xdx + \int^{3}_{1}(-3x)dx$

⇒$[\frac{x^2}{2}]^{1}_{0} + [\frac{-3x^2}{2}]^{3}_{1}$

⇒$[\frac{1}{2}] + |(-3)[\frac{3^2}{2} - \frac{1^2}{2}]|$

⇒$\frac{1}{2}+ |(-3)4|$

⇒$\frac{1}{2}+ 12$

⇒$\frac{25}{2}$