30-60-90 公式

其中有各种类型的三角形，据说有些特殊，其中有30-60-90。如果其中一个角是 90°，我们通常将三角形称为直角。因此，我们可以说30-60-90是一种特殊的直角三角形。这种类型的三角形将始终具有 30°、60° 和 90° 的角度。

30-60-90 公式

下图表示 30-60-90 三角形，∠A = 60°，∠B = 90°，∠C = 30°。 30-60-90 发音为“三十 - 六十 - 九十”。

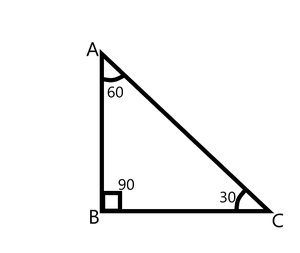
30-60-90 三角形

与 30° 角相对的一侧保持最小值，并设为“a”厘米。与60°角相对的另一边为中等值，为“a√3”厘米。最后，与90°角相对的一侧的值最大，为“2a”cm。

从下图中，

• AB = a cm（与 30° 角相反）⇢ 最短边
• BC = a√3 cm（与 60° 角相反）⇢ 中间侧
• AC = 2a cm（与 90° 角相反）⇢ 最大边

因此，AB:BC:CA = a:√3a:2a

否定“a”，因为它很常见。现在，看看 30-60-90 三角形的边比，即 1:√3:2。

为了证明这一点，让我们考虑一个等边三角形，即所有边的长度相同的三角形，并将其设为“a”厘米。

让我们首先考虑如图所示的等边三角形（所有边相等并且在顶点处成60°角）。如果我们从一个顶点（比如 A）到另一边（比如 BC）画一条线。然后另一边即BC被分成2等分（每部分有a/2）并形成90°角。设 BC 的分界点或中点为 D。由于绘制的直线，顶点 A 处的 60°角也将被等分，每个部分保持 30°。

现在看图的一半，即三角形 ABD，它类似于一个 30-60-90 的三角形，边 AB = a cm，BD = a/2 cm，AD = 未知（比如 x cm）

要找到 AD 的值，让我们使用毕达哥拉斯定理，该定理指出“在直角三角形中，斜边（最长边）的平方等于其他两条边的平方和”，从图中AB是斜边，BD和AD是另外2边。

所以，

AB ² = BD ² + AD ²

a ² = (a/2) ² + x ²

x ² = a ² – (a/2) ²

x = √3a/2 厘米 (AD)

与角 30-60-90 相对的边的比率将是 a/2: √3a/2: a ⇒ 1:√3:2（取为普通并忽略它并乘以 2）

This ratio 1:√3:2 is known as the 30-60-90 formula

30-60-90 三角形面积

To find the area of 30-60-90 let’s consider the common formula for the area of triangle i.e., = 1/2 × base × height

For the triangle, ABD, AD act as height (√3a/2 cm), and BD act as a base (a/2)

Therefore the area of triangle =1/2 × a/2 × √3a/2

Area = √3a²/8

示例问题

问题1：如果30-60-90三角形的两条边分别是20厘米和40厘米，求另一边。

解决方案：

Given 2 sides are 20 and 40, which are in the ratio of 1:2

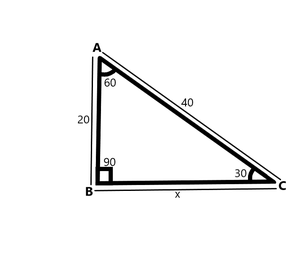
To find the third side i.e., x from the 30-60-90 formula 1:√3:2 x ⇒ √3a

 x ⇒ 20 × √3 cm. 

x = 20√3cm 

问题2：30-60-90的最短边是40cm，求三角形的面积？

解决方案：

Given the shortest side is 40cm i.e., a = 40 cm.

Area of 30-60-90 is given as (√3 × a²)/8

Therefore Area ⇒ (√3 × 40 × 40) / 8

⇒ 200√3 cm².

问题3：30-60-90的最长边是120cm，求三角形的面积？

解决方案：

Given the longest side is 120cm i.e., 2a = 120 cm.

Therefore, a = 60 cm.

Area of 30-60-90 is given as (√3 × a²)/8

Therefore Area ⇒ (√3 × 60 × 60) / 8

⇒ 450√3 cm².

问题4：30-60-90的中边是12√3cm，求三角形的面积？

解决方案：

Given the moderate side is 120cm i.e., a√3 = 12√3 cm.

Therefore, a = 12 cm.

Area of 30-60-90 is given as (√3 × a²)/8

Therefore Area ⇒ (√3 × 12 × 12) / 8

⇒ 18√3 cm².

问题5：三角形的最短边是90厘米，求最长边？

解决方案：

Given the shortest side of 30-60-90 is 90 cm.

From the 30-60-90 formula the shortest and longest sides are in the ratio 1:2 ⇒ x:2x

Given x = 90 2x = ?

Therefore, 2x = 2 × 90 = 180 cm.

问题6：三角形最长边是20cm，求中间边长？

解决方案：

Given the longest side of 30-60-90 is 20 cm.

From 30-60-90 formula the longest and intermediate sides are in the ratio 2:√3 ⇒ 2x :√3 x

Given 2x = 20   

x = 10  

Therefore, √3x = √3 × 10 = 10√3 cm.