📌  相关文章
📜  开普勒行星运动定律

📅  最后修改于: 2022-05-13 01:57:37.467000             🧑  作者: Mango

开普勒行星运动定律

行星和太阳的周期、轨道和区域通常是开普勒行星运动定律的重点。在以太阳为中心的太阳系中，行星在椭圆轨道上围绕太阳旋转，太阳在一点。开普勒根据他对行星运动的评估制定了一套规则，现在被称为开普勒三定律。

开普勒的三项定律详细说明了行星根据它们在太空中的轨迹如何表现。 1609 年，《新天文学》发表了开普勒运动定律的前两条。他们的发现被证明是推进现代科学的真诚尝试。

开普勒定律简介

运动的概念总是相对的。根据运动中粒子的能量，运动分为两类：

有界运动：粒子在有界运动中具有负的总能量（E < 0），并且有两个或多个极值点，总能量总是等于粒子的势能，即粒子的动能变为零。

当偏心率 0 ≤ e < 1 时，E < 0 表示物体的运动是有界的。圆形轨道的偏心率为零，而椭圆轨道的偏心率为 1。

无界运动：无界运动中的粒子具有正的总能量（E > 0）和一个总能量始终等于粒子势能的单一极值点，即粒子的动能变为零。

当偏心率e≥1时，E>0表示物体自由运动。抛物线轨道的离心率 e = 1，而双曲线轨道的离心率 e > 1。

轨道定律、等积定律和周期定律是开普勒的第一、第二和第三定律，下面将详细描述。

开普勒第一定律——轨道定律

开普勒第一定律被称为椭圆定律，它指出行星以椭圆形围绕太阳旋转。轨道定律是开普勒第一定律的通称。

行星在我们已经熟悉的圆形轨道上围绕太阳运行。然而，根据开普勒的说法，行星确实围绕太阳旋转，尽管不是在圆形轨道上。但是，它以椭圆为中心。

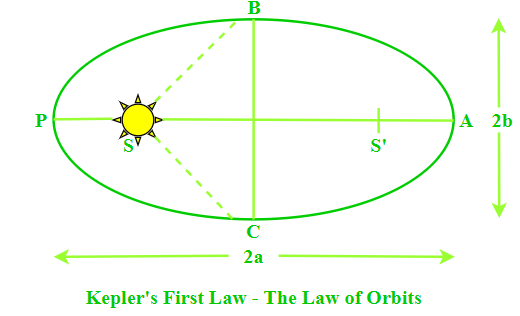
椭圆中有两个焦点 (S & S')。太阳 (S) 位于椭圆的焦点之一。近日点（PS）是指行星离太阳最近的点，而远日点（AS）是指行星离太阳最远的点。长轴是（2a），短轴是（2b）。任何行星到两个焦点的距离总和是恒定的，这是椭圆的属性之一。季节是由行星的椭圆轨道带来的。

开普勒第二定律——面积定律

开普勒第二定律，通常被称为面积定律，决定了每颗行星绕太阳旋转的速度。每颗行星在太空中旋转的速度不断变化。当一颗行星离太阳最近时，它自转最快，当它离太阳最远时，它自转最慢。

根据定律的数学公式，行星或旋转物体在给定时间时所覆盖的区域是相同的，无论与焦点处的项目距离如何。因为面积相等，所以距离越远的弧越短，导致速度越慢。这适用于轨道上的所有物体。

地球离太阳最近时形成的区域可以计算为一个宽而短的三角形，如下图所示。另一方面，当地球离太阳最远时形成的区域可以近似为一个窄而长的三角形。这些区域的大小大致相同。当地球离太阳最远时，这些三角形的底边最短。已经注意到，如果这个假设区域的大小与地球离太阳最近时的大小相同，那么当地球离太阳最远时，它必须移动得更慢。

动量守恒定律

该定律也可以通过角动量守恒定律来验证，该定律指出，在固定轨道上围绕恒星旋转的行星的动量沿轨道始终是恒定的。

行星在位置 B 的角动量 L B = mr B v B 。

行星在位置 D 的角动量，L D = mr D v D 。

现在，由于 L 在任何点都是常数，所以 L B = L D

⇒ r B v B = r D v D

上述表达式表明，对于在轨道上旋转的行星，距离和速度彼此成反比。

开普勒第三定律——周期定律

开普勒第三定律，通常被称为周期定律，建立了行星的轨道周期和轨道半径与其他行星的比较。第三定律比较了各种行星的运动特性，与开普勒第一和第二定律相反，开普勒第一和第二定律描述了单个行星的运动特性。

开普勒第三定律定义了行星恒星系统的质量、行星与恒星的距离以及它们的轨道周期之间的关系。它指出，行星时间周期的平方与行星轨道半长轴的立方成正比，与恒星和行星质量之和成反比。

示例问题

问题 1：当从太阳表面看时，下列哪些量在行星运动中保持不变？

回答：

问题2：行星的半径是地球半径的3倍。计算行星公转的时间。

解决方案：

问题 3：轨道角动量（关于太阳）与地球质量的比值为 4.4 × 10 15 m 2 ⁄ s。找到地球所包围的区域。

解决方案：

问题 4：定义开普勒第一定律。

回答：

问题 5：根据运动中粒子的能量定义行星的运动。

回答：