实际气体与理想气体行为的偏差
物质状态是不同形式之一。在日常生活中,可以看到四种物质状态:固体、液体、气体和等离子体。已知存在许多中间态,例如液晶,而某些状态,例如玻色-爱因斯坦凝聚态、中子简并物质和夸克-胶子等离子体,仅在极端条件下存在,例如极端寒冷、极端的密度和极端的能量。有关所有奇异物质状态的完整列表,请参阅物质状态列表。
真实气体的行为
All of the gases are real-life instances. Although there is no such thing as an ideal gas, genuine gases are known to behave in ideal ways under certain circumstances. Nitrogen, oxygen, hydrogen, carbon dioxide, helium, and other actual gases are examples.
理想气体和真实气体:理想气体在所有压力和温度下都遵循理想气体方程 PV=nRT。另一方面,没有气体是极好的。几乎所有气体都以某种方式与理想行为不同。非理想或实际气体,例如 H 2 、N 2和 CO 2 ,不符合理想气体方程。
压缩系数
一个称为压缩系数的新函数,用 Z 表示,可用于量化实际气体偏离理想行为的程度。它被描述为
Z = PV/RT
绘制可压缩因子 Z 与 P 的关系图,揭示了偏离最佳行为的程度。温度和压力对 Z 值为 1 的理想气体没有影响。Z 值大于或小于 1 决定了理想气体行为与实际气体行为之间的差异。气体不理想的程度由单位和 Z 之间的差异表示。压力和温度会导致偏离实际气体的最佳行为。使用压力和温度来检查下面讨论的一些气体的可压缩性曲线。
压力变化对偏差的影响
在下图中,绘制了恒定温度下 H 2 、N 2和 CO 2的压缩系数 Z 与压力的关系图。
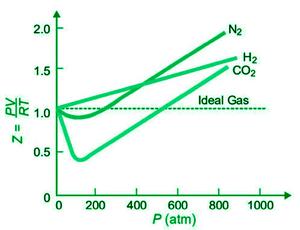
对于所有这些气体,Z 在非常低的压力下实际上等于 1。真实气体在低压(高达 10 个大气压)下表现几乎完美。随着压力升高,H 2表现出 Z 的稳定增加(从 Z=1)。结果,H 2曲线在所有压力下都高于理想气体曲线。对于N 2和CO 2 ,Z首先减小(Z1),然后达到最小值,最后随着压力的增加而增加(Z>1)。因为CO 2是最容易液化的气体,所以它的曲线下降幅度最大。
温度对偏差的影响
下图显示了不同温度下 N 2的 Z 或 PV/RT 与 P 的关系图。
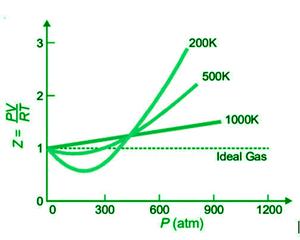
随着温度的升高,与理想气体行为的偏差越来越小,如图所示。在较低温度下,曲线明显下降,曲线斜率为负。在这种情况下,Z<1。随着温度的升高,曲线的倾角减小。曲线的最小值在一定温度下消失,并在很宽的压力范围内保持水平。在此温度下,PV/RT 几乎相等,因此满足波义耳定律。因此,波义耳温度指的是气体的温度。每种气体都有自己的波义耳温度,例如 N 2为 332K。
Note:
- Real gases perform approximately ideal at low pressures and relatively high temperatures, and the ideal-gas equation is obeyed.
- A real gas deviates greatly from ideality at low temperatures and sufficiently high pressures, and the ideal-gas equation is no longer valid.
- As the gas approaches the liquefaction point, the departure from ideal behaviour grows.
范德华方程(真实气体行为的原因)
根据范德华 (Van der Waals) (1873),实际气体与理想行为的偏差可归因于两个错误的动力学理论假设。以下是其中一些:
- 气体分子没有体积,有点质量。
- 气体中没有分子间的吸引力。
因此,从动力学理论发展而来的理想气体方程 PV=nRT 不能应用于实际气体。范德华指出,理想气体方程的压力 (P) 和体积 (V) 部分需要修改以使其适用于实际气体。
音量校正
气体的体积是容器中分子可以移动的自由空间的量。理想气体的体积 V 等于容器的体积。因为理想的气体分子没有体积,它们可以在容器内自由移动。相比之下,范德华认为真正气体中的分子是具有固定体积的刚性球形物体。
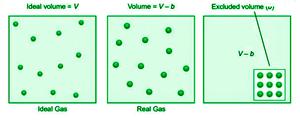
因此,实际气体的体积是理想体积减去气体分子体积。如果 b 是每摩尔气体的有效分子体积,则理想气体方程的体积修正如下:
对于 n 摩尔气体,它变为 (V-b)。
(V–nb)
在哪里,
排除体积,缩写为 b,是每种气体的恒定且唯一的量。
压力校正
气体内部的分子被四面八方的分子吸引。这些吸引人的特征相互抵消。另一方面,准备撞击容器壁的分子仅被一侧的分子吸引。结果感觉是被迫进去的。结果,它以较小的力撞击墙壁,实际气体压力 P 小于理想压力。如果实际压力 P 比理想压力 P Ideal小 p 倍,我们有
P = P理想- p
P理想= P + p
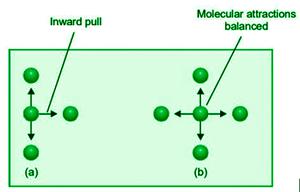
范德瓦尔斯确定数量 p 的值如下:
p = 一个2 /V 2
其中n是体积V中气体分子的总数,a表示气体的比例常数。因此,理想气体方程的压力 P 被修改为:
对于 n 摩尔气体,使用 (P+an 2/ V 2 )。
当调整后的压力和体积数代入理想气体方程时,PV=nRT,我们得到:
(p+an 2/ V 2 ) (V–nb) = nRT
对于 n 摩尔气体,这称为范德华方程或真实气体方程。范德华方程变为: 对于 1 摩尔气体 (n=1):
(p+a/V 2 )(V–b) = RT
示例问题
问题1:定义理想气体和真实气体。
回答:
An ideal gas obeys the ideal gas equation PV = nRT at all pressures and temperatures. No gas, on the other hand, is excellent. Almost all gases vary in some manner from the ideal behaviour. Non-ideal or actual gases, such as H2,N2, and CO2, do not obey the ideal-gas equation.
问题2:什么是压缩系数?
回答:
The degree to which real gas deviates from ideal behaviour can be expressed using a new function called the Compressibility factor, indicated by Z.
Z=PV/RT
问题 3:气体的理想行为是什么?
回答:
An ideal gas obeys the ideal gas equation PV=nRT at all pressures and temperatures. However, no gas is ideal. Almost all gases vary in some manner from the ideal behaviour.
问题 4:真实气体的行为是什么?
回答:
Gases that depart from ideal behaviour are known as real gases. The degree to which real gas deviates from ideal behaviour can be expressed using a new function called the Compressibility factor, indicated by Z.
Z=PV/RT
A plot of the compressibility factor, Z, vs P can reveal the degree of divergence from ideal behaviour. Z=1 for an ideal gas, and it is unaffected by temperature or pressure.
问题 5:实际气体行为的原因是什么?导出范德华方程。
回答:
According to Van der Waals (1873), the deviations of real gases from ideal behaviour are attributable to two faulty kinetic theory postulates. The following are some of them:
1. In a gas, the molecules have no volume and have point masses.
2. There are no intermolecular attractions in a gas.
As a result, the ideal gas equation PV=nRT developed from kinetic theory could not be applied to real gases. Van der Waals pointed out that both the pressure (P) and volume (V) elements of the ideal gas equation needed to be modified to make it applicable to real gases.
Van der Waals changed the ideal gas equation to make it applicable to all gases. The Van der Waals equation for n moles of gases,
(p+an2/V2)(V–nb)=nRT
问题 6:导出体积校正公式。
回答:
A real gas’s volume equals the ideal volume minus the volume occupied by gas molecules. If b is the effective volume of molecules per mole of gas, the volume in the ideal gas equation is rectified as follows:
(V–b)
For n moles of gas,
(V–nb)
Where the excluded volume, which is constant and unique to each gas, is denoted by the letter b.