📌  相关文章
📜  理想气体方程和绝对温度

📅  最后修改于: 2022-05-13 01:57:36.745000             🧑  作者: Mango

理想气体方程和绝对温度

一端带有充满液体的灯泡的温度计，最常用的液体是水银、甲苯、酒精、戊烷、杂酚油，由于其不同的膨胀特性，除了固定读数外，它们显示不同的温度读数。另一方面，使用气体的温度计显示相同的温度读数。使用哪种类型的气体并不重要。实验表明，所有气体在低密度下都以相同的方式膨胀。

压力 (P)、体积 (V) 和温度 (T) 其中 T = t + 273.15 和 t 是以°C 为单位的温度是解释给定数量（质量）气体行为的变量。理想气体定律，也称为通用气体方程，是一种假设理想气体的方程状态。尽管存在缺陷，但理想气体定律很好地近似了许多气体在各种情况下的行为。 Benoit Paul Émile Clapeyron 在 1834 年提出了理想气体定律，它是经验查尔斯定律、波义耳定律、阿伏伽德罗定律和盖伊定律的混合体。吕萨克的。

理想气体方程

理想气体定律是 17 世纪 Boyle 和 18 世纪 Charles 的观测工作的结合。

波义耳定律：对于保持在固定温度下的给定量气体，气体压力与气体体积成反比，即在恒定温度下，一定量气体的压力和体积之间的关系可以写为，

查尔斯定律：对于保持在恒定压力下的给定固定数量的气体，气体体积与气体温度成正比，即在恒定温度下，一定量气体的体积和温度之间的关系可以写为，

这两个定律适用于低密度气体，可以归为一个关系。值得注意的是，

PV = 常数

和

V/T = 常数

对于特定数量的气体，则

因此PV/T也应该是一个常数。

它可以以更一般的形式表述，适用于任何数量的任何低密度气体，而不仅仅是特定数量的该气体。这种关系描述了理想气体定律，称为理想气体方程。

它也可以以更一般的形式表述，适用于任何数量的任何低密度气体，而不仅仅是特定数量的该气体。

理想气体方程的推导

绝对温度

温度与压力和体积有直接关系，即

PV ∝ T

这种关系使气体能够用于确定具有恒定体积的气体温度计中的温度。

因此，在恒定体积下，该关系可以写为，  

P ∝ T ，并且温度是用定容气体温度计根据压力读取的。

一条直线从压力与温度的关系图中出现。

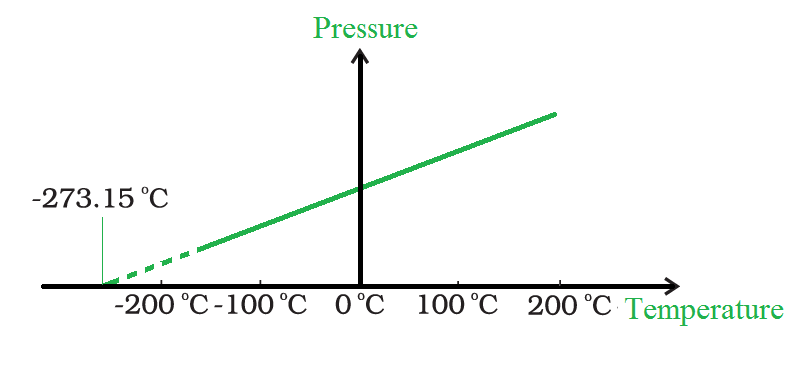
在恒定体积下，低密度气体的压力与温度的关系图

对真实气体的观察与低温下理想气体定律的预期值不同。然而，这种关系在很宽的温度范围内是线性的，如果气体仍然是气体，压力会随着温度的降低而下降到零。将直线外推到轴产生理想气体的绝对最低温度。绝对零定义为 - 273.15 摄氏度的温度。开尔文温标，通常称为绝对标度温度，建立在绝对零上。

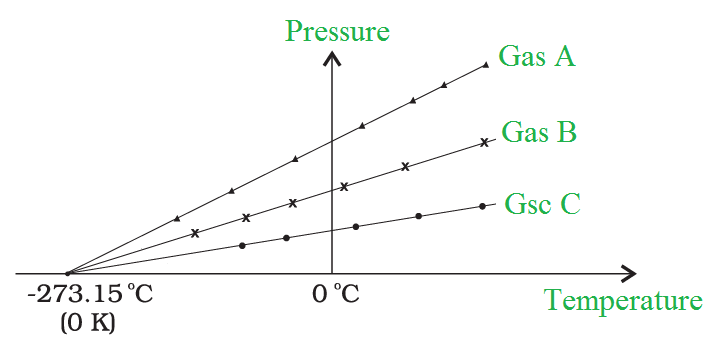
压力与温度的关系图中相同的绝对零温度以及低密度气体的线外推。

在开尔文温标上，以– 273.15 °C 作为零点，即0 K。在开尔文和摄氏温度系统中，单位大小是相同的。因此，它们之间的关系可以表示为

T = t + 273.15

其中 t 是以°C 为单位的温度

示例问题

问题1：STP 2.34 克二氧化碳气体所占的体积是多少？

解决方案：

问题 2：STP 的氩气样品占 56.2 升。确定样品中氩的摩尔数和氩的质量。

解决方案：

问题 3：在什么温度下，0.654 摩尔氖气在 1.95 个大气压下会占据 12.30 升？

解决方案：

问题 4：推导理想气体方程？

解决方案：

问题 5：将 5.600 g 固体 CO2 放入一个 4.00 L 的空密封容器中，温度为 300 K。当所有固体 CO2 变成气体时，容器中的压力是多少？

解决方案：