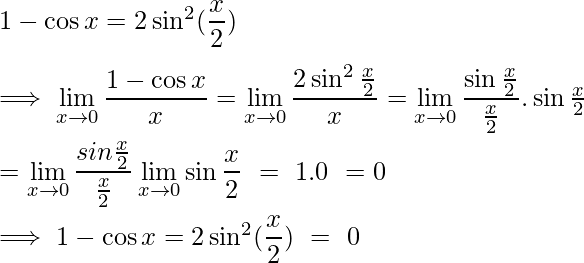挤压定理–极限

挤压定理也被称为三明治定理，三明治规则，警察定理，捏定理有时是挤压引理， carabinieri定理在数学分析中用于寻找一个函数的极限，当另外两个函数的极限成立时。已知存在。压缩定理适用于微积分和数学分析。它通常用于通过与其他两个已知或易于计算其极限的函数进行比较来确定一个函数的极限。它最初是由数学家阿基米德和Eudoxus进行几何运算以计算π的，并由卡尔·弗里德里希·高斯(Carl Friedrich Gauss)用现代术语表述。

定理

压缩定理的形式如下：

Let functions f(x), g(x), h(x) be real functions such that h(x) ≤ g(x) ≤ f(x). Then for all x in the domain of the function,

$if\ \lim\limits_{x\rightarrow a}h(x)=\lim\limits_{x\rightarrow a}f(x)=L, then \lim\limits_{x\rightarrow a}g(x)=L$
Here the functions h and f are said to be lower and upper bounds of g respectively. The above function is represented in a graph below.

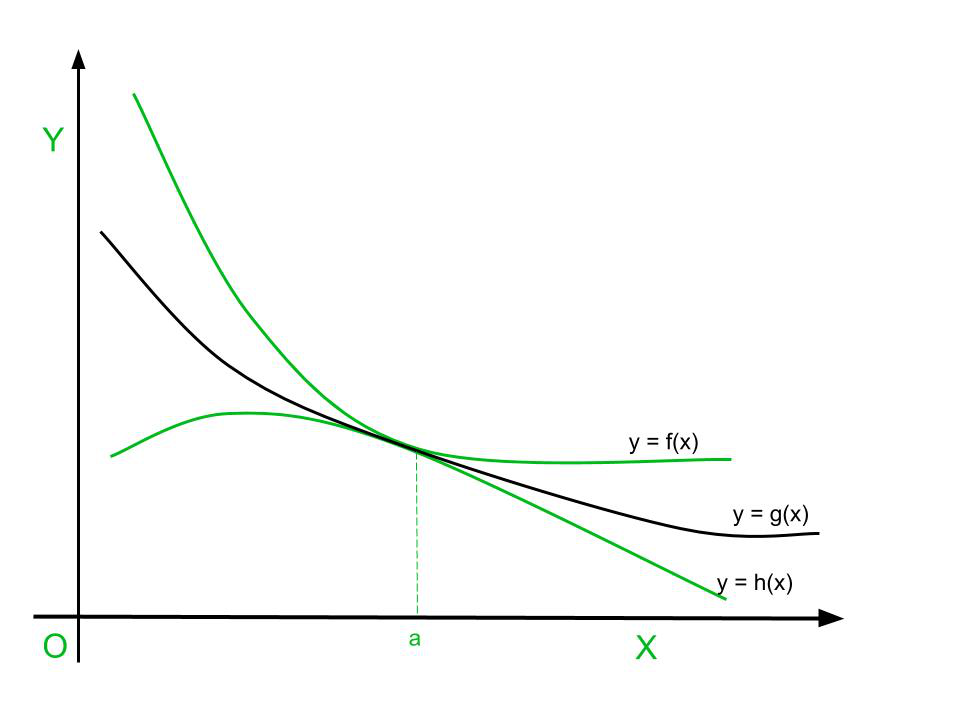
重要不平等

$\\cos\ x<\dfrac{\sin\ x}{x}<1,\ 0<|x|<\dfrac{\pi}{2}, \\ as\ \sin (-x)\ =\ -\sin\ x \ and\ \cos(-x)\ =\ \cos\ x, \\ so\ we\ just\ prove\ the\ inequality\ for\ the\ interval\ (0,\dfrac{\pi}{2})$
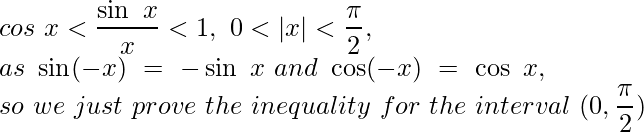
证明：

考虑下图：

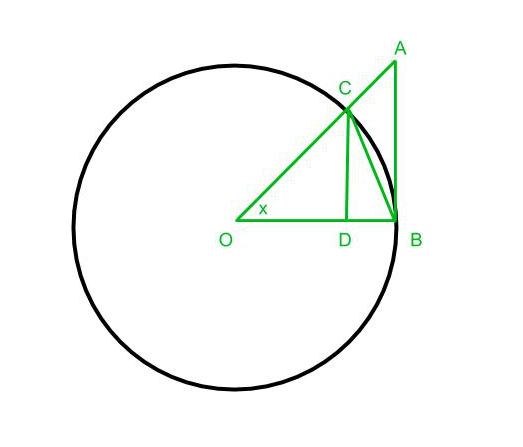
In the diagram above O is the centre of the circle AB is a tangent of the circle from point B and CD is perpendicular to OB and angle COD is x radians

$here,\ Area\ of \ \triangle OBC\ < \ Area\ of\ sector\ OBC\ < Area\ of\ \triangle OBA\\\qquad\\ \implies\ \dfrac{1}{2}OB.CD<\dfrac{x}{2\pi}\pi(OB)^2<\dfrac{1}{2}OB.BA\\\qquad\\ \implies\ CD<x.(OA)<AB.\\\qquad\\ in\ \triangle OCD\\\qquad\\ \sin x=\dfrac{CD}{CO}=\dfrac{CD}{OB}(OC=OB=radius)\ hence\ CD=OB\sin x\\\qquad\\ \tan x=\dfrac{AB}{OA}\ hence\ AB=OB\tan x\\\qquad\\ \implies OB\sin x\ <\ OB.x\ <\ OB\tan x\ and OB>0\\\qquad\\ \implies \sin x\ <\ x\ <\ \tan x\\\qquad\\ 0\ <\ x\ <\ \dfrac{\pi}{2},\ so \ by \ dividing \ by \sin x\ on\ the\ above\ equation,\ we\ get\\\qquad\\ 1\ <\ \dfrac{x}{\sin x}\ <\ \dfrac{1}{\cos x}\\\qquad\\$

by taking the reciprocals we get
$\cos x\ <\ \dfrac{\sin x}{x}\ <\ 1$

重要限制

$1.\ \lim\limits_{x\rightarrow\ 0}\dfrac{sin x}{x}=1$

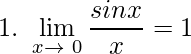
证明：

From the above inequality, we proved that
$\cos x\ <\ \dfrac{\sin x}{x}\ <\ 1$
From this equation, we understand that (sin x/x) always lies between cos x and 1. So (sin x/x) is sandwiched between 1 and cos x. We know that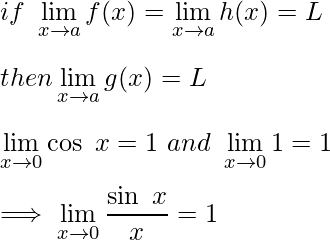
$\\\qquad\\ if\ \lim\limits_{x\rightarrow a}f(x)=\lim\limits_{x\rightarrow a}h(x)=L\\\qquad\\ then \lim\limits_{x\rightarrow a}g(x)=L\\\qquad\\ \lim\limits_{x\rightarrow0}\cos\ x=1\ and\ \lim\limits_{x\rightarrow0}1=1 \\\qquad\\ \implies \lim\limits_{x\rightarrow0}\dfrac{\sin\ x}{x}=1$

$2.\ \lim\limits_{x\rightarrow 0}\dfrac{1-cosx}{x}=0$

证明：

To prove this limit we use the trigonometric identity
$1-\cos x=2\sin^2(\dfrac{x}{2})\\\qquad\\ \implies\lim\limits_{x\rightarrow0}\dfrac{1-\cos x}{x}=\lim\limits_{x\rightarrow0}\dfrac{2\sin^2\frac{x}{2}}{x}=\lim\limits_{x\rightarrow0}\dfrac{\sin \frac{x}{2}}{\frac{x}{2}}.\sin\frac{x}{2}\\\qquad\\ =\lim\limits_{x\rightarrow0}\dfrac{sin\frac{x}{2}}{\frac{x}{2}}\lim\limits_{x\rightarrow0}\sin \dfrac{x}{2}\ =\ 1.0\ =0\\\qquad\\ \implies 1-\cos x=2\sin^2(\dfrac{x}{2})\ =\ 0$