11类RD Sharma解决方案–第六章三角函数图–练习6.1

问题1.绘制以下图形：

(i)y = 2罪2x

解决方案：

To obtain this graph y = 2 sin 2x,

First we draw the graph of y = sin x and then divide the x-coordinates of the points where it crosses x-axis by 2.

The maximum and minimum values of y are 2 and -2 respectively.

(ii)y = 3罪x

解决方案：

To obtain this graph y = 3 sin x,

First we draw the graph of y = sin x.

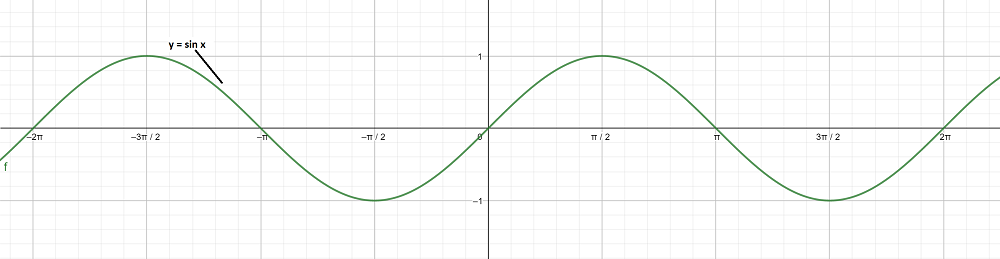
The maximum and minimum values of y are 3 and -3 respectively.

(iii) $y = 2 \hspace{0.1cm}sin (x-\frac{\pi}{4})$

解决方案：

To obtain this graph y-0 = $2 \hspace{0.1cm}sin (x-\frac{\pi}{4})$,

On shifting the origin at (π/4, 0), we get

X = $x-\frac{\pi}{4}$ and Y = y – 0

On substituting these values, we get

Y = 2 sin X

First we draw the graph of Y = 2 sin X and shift it by π/4 to the right.

(iv)y = 2罪(2x – 1)

解决方案：

To obtain this graph y – 0 = 2 sin $2(x-\frac{1}{2})$,

On shifting the origin at (1/2, 0), we get

X = $x-\frac{1}{2}$ and Y = y – 0

On substituting these values, we get

Y = 2 sin 2X

First we draw the graph of Y = 2 sin 2X and shift it by 1/2 to the right.

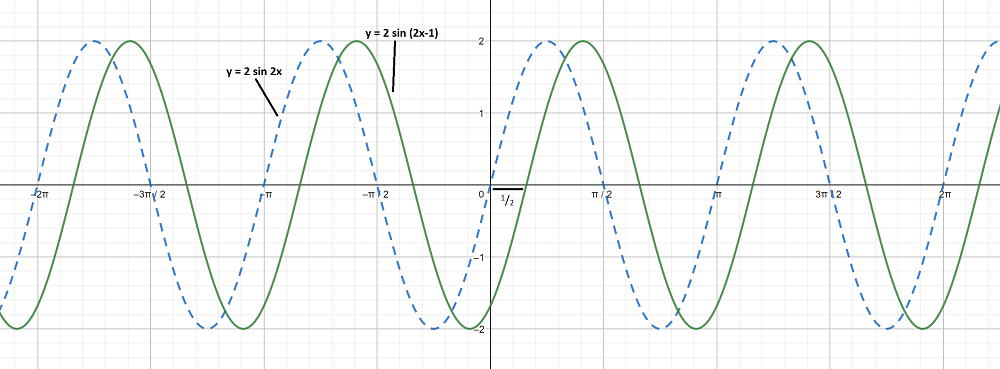
(v)y = 3罪(3x +1)

解决方案：

To obtain this graph y – 0 = 3 sin 3$(x+\frac{1}{3})$,

On shifting the origin at(-1/3, 0), we get

X = $x+\frac{1}{3}$ and Y = y – 0

On substituting these values, we get

Y = 3 sin 3X

First we draw the graph of Y = 3 sin 3X and shift it by 1/3 to the left.

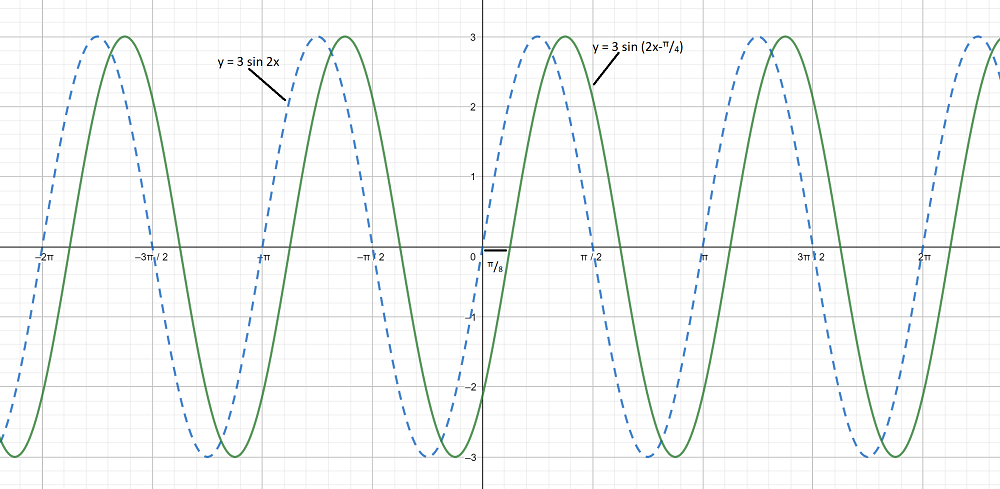
(六) 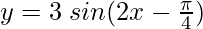$y = 3 \hspace{0.1cm}sin (2x-\frac{\pi}{4})$

To obtain this graph y-0 = $3 \hspace{0.1cm}sin \hspace{0.1cm}2(x-\frac{\pi}{8}),$

On shifting the origin at (π/8, 0), we get

X = $x-\frac{\pi}{8}$ and Y = y-0

On substituting these values, we get

Y = 3 sin 2X

First we draw the graph of Y = 3 sin 2X and shift it by π/8 to the right.

问题2：在同一轴上画出以下几对函数的图形：

(i)y =罪x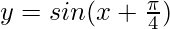， $y = sin (x+\frac{\pi}{4})$

Graph 1:

y = sin x

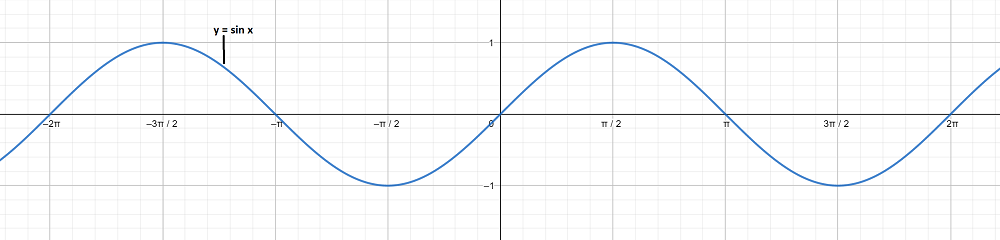
Graph 2:

To obtain this graph y-0 = $sin (x+\frac{\pi}{4}),$

On shifting the origin at (-π/4, 0), we get

X = $x+\frac{\pi}{4}$ and Y = y – 0

On substituting these values, we get

Y = sin X

First we draw the graph of Y = sin X and shift it by π/4 to the left.

The graph y = sin x and $y = sin (x+\frac{\pi}{4})$ are on different axes are as follows:

(ii)y =罪x，y =罪3x

Graph 1:

y = sin x

Graph 2:

To obtain this graph y = sin 3x,

First we draw the graph of y = sin x and then divide the x-coordinates of the points where it crosses x-axis by 3.

The graph y = sin x and y = sin 3x are on different axes are as follows: