11类RD Sharma解决方案–第5章三角函数–练习5.2

问题1.在以下每个中找到其他五个三角函数的值：

(i)cot x = 12/5，x在象限III中

(ii)cos x = -1 / 2，x在第二象限

(iii)tan x = 3/4，x在象限III中

(iv)sin x = 3/5，x在第I象限

解决方案：

(i) cot x = 12/5, x in quadrant III

As we knew that tan x and cot x are positive in third quadrant 

and sin x, cos x, sec x, cosec x are negative.

By using the formulas,

tan x = 1/cot x 

= $\frac{1}{\frac{12}{5}}$

= 5/12

cosec x = $-\sqrt{1 + cot^2 x}$

$= -\sqrt{1 + \left(\frac{12}{5}\right)^2}\\ = -\sqrt{\frac{(25+144)}{25}}\\ = -\sqrt{\frac{169}{25}}$

= -13/5 

sin x = 1/cosec x 

= $\frac{1}{\frac{-13}{5}}$

=- 5/13

cos x = $-\sqrt{1 - sin^2 x}$

$\\ = - \sqrt{1 - \left(\frac{-5}{13}\right)^2}\\ = - \sqrt{\frac{(169-25)}{169}}\\ = -\sqrt{\frac{144}{169}}$ 

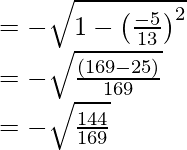
= -12/13 

sec x = 1/cos x 

= $\frac{1}{\frac{12}{13}}$

= – 13/12

Hence, the values of the other five trigonometric functions are: sin x = -5/13, cos x = -12/13, tan x = 5/12, cosec x = -13/5, sec x = -13/12 

(ii) cos x = -1/2, x in quadrant II

As we knew that sin x and cosec x are positive in second quadrant and

tan x, cot x, cos x, sec x are negative.

By using the formulas, we get

sin x = $\sqrt{1 - cos^2 x}$
$\\ = \sqrt{1 - \left(-\frac{1}{2}\right)^2}\\ = \sqrt{\frac{(4-1)}{4}}\\ = \sqrt{\frac{3}{4}}\\ = \frac{\sqrt3}{2}$
$\\ tan x = \frac{sin x}{cos x}\\ = \frac{\frac{\sqrt3}{2}}{{-\frac{1}{2}}}\\ = -\sqrt3$
$\\ cot x = \frac{1}{tan x}\\ = \frac{1}{-\sqrt3}\\ = \frac{-1}{\sqrt3}$
$\\ cosec x = \frac{1}{sin x} = \frac{1}{\frac{\sqrt3}{2}}\\ = \frac{2}{\sqrt3}$
$\\ sec x = \frac{1}{cos x}\\ = \frac{1}{\frac{-1}{2}}$
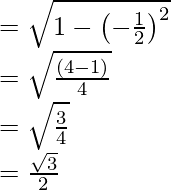
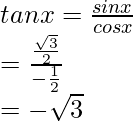
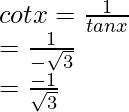
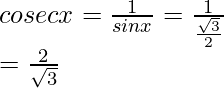
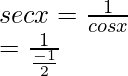
= -2

Hence, the values of the other five trigonometric functions are: sin x = √3/2, tan x = -√3, cosec x = 2/√3, cot x = -1/√3, sec x = -2

(iii) tan x = 3/4, x in quadrant III

As we knew that tan x and cot x are positive in third quadrant and sin x, cos x, sec x, cosec x are negative.

By using the formulas,

sin x = $\sqrt{1 - cos^2 x}$
$\\ = - \sqrt{(1-\left(\frac{-4}{5}\right)^2}\\ = - \sqrt{\frac{(25-16)}{25}}\\ = - \sqrt{\frac{9}{25}}\\ = - \frac{3}{5}$
$\\ cos x = \frac{1}{sec x}\\ = \frac{1}{\frac{-5}{4}}\\ = \frac{-4}{5}$
$\\ cot x = \frac{1}{tan x}\\ = \frac{1}{\frac{3}{4}}\\ = \frac{4}{3}$
$\\ cosec x = \frac{1}{sin x}\\ = \frac{1}{\frac{-3}{5}}\\ = \frac{-5}{3}$
$\\ sec x = -\sqrt{1 + tan^2 x}\\ = - \sqrt{1+\left(\frac{3}{4}\right)^2}\\ = - \sqrt{\frac{(16+9)}{16}}\\ = - \sqrt{\frac{25}{16}}\\ = \frac{-5}{4}$

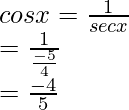
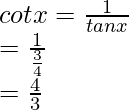
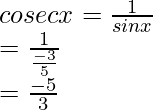
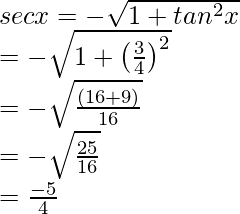
Hence, the values of the other five trigonometric functions are: sin x = -3/5, cos x = -4/5, cosec x = -5/3, sec x = -5/4, cot x = 4/3 

(iv) sin x = 3/5, x in quadrant I

As we knew that, all trigonometric ratios are positive in first quadrant.

So, by using the formulas,

tan x = $\frac{sin x}{cos x}$
$\\ = \frac{\frac{3}{5}}{\frac{4}{5}}\\ = \frac{3}{4}$
$\\ cosec x = \frac{1}{sin x}\\ = \frac{1}{\frac{3}{5}}\\ = \frac{5}{3} \\cos x = \sqrt{1-sin^2 x}\\ = \sqrt{1 - \left(\frac{-3}{5}\right)^2}\\ = \sqrt{\frac{(25-9)}{25}}\\ = \sqrt{\frac{16}{25}}\\ = \frac{4}{5}$
$\\ sec x = \frac{1}{cos x}\\ = \frac{1}{\frac{4}{5}}\\ = \frac{5}{4}$
$\\ cot x = \frac{1}{tan x}\\ = \frac{1}{\frac{3}{4}}\\ = \frac{4}{3}$

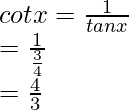
Hence, the values of the other five trigonometric functions are: cos x = 4/5, tan x = 3/4, cosec x = 5/3, sec x = 5/4, cot x = 4/3 

问题2.如果sin x = 12/13并位于第二象限中，则求出sec x + tan x的值。

解决方案：

Given:

Sin x = $\frac{12}{13}$and x lies in the second quadrant.

We know, in second quadrant, sin x and cosec x are positive and all other ratios are negative.

So, by using the formulas, we get

Cos x = $\sqrt{1-sin^2 x}$
$\\ = - \sqrt{1-\left(\frac{12}{13}\right)^2}\\ = - \sqrt{1- \left(\frac{144}{169}\right)}\\ = - \sqrt{\frac{(169-144)}{169}}\\ = -\sqrt{\frac{25}{169}}\\ = - \frac{5}{13}$

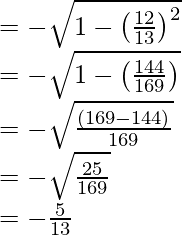
tan x = sin x/cos x 

sec x = 1/cos x

$tan x = \frac{\frac{12}{13}}{\frac{-5}{13}}\\ = -\frac{12}{5}\\ sec x = \frac{1}{\frac{-5}{13}}\\ = \frac{-13}{5}$

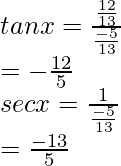
Sec x + tan x = ((-13/5) +(-12/5))

= (-13 – 12)/5 = -25/5 = -5

Hence, the value of Sec x + tan x = -5

问题3.如果sin x = 3/5，tan y = 1 /2，π / 2 3π / 2，则得出8 tan x-√5sec y的值。

解决方案：

Given, sin x = 3/5, tan y = 1/2, and π​/2 < x< π< y< 3π​/2

Here, x is in second quadrant and y is in third quadrant. So, cos x and 

tan x are negative in second quadrant and sec y is negative in third quadrant.

So, by using the formula, we get

cos x = $- \sqrt{1-sin^2 x}$

tan x = sin x/ cos x 

cos x = $- \sqrt{1-sin^2 x}$
$\\ = - \sqrt{1 - \left(\frac{3}{5}\right)^2}\\ = - \sqrt{1 - \frac{9}{25}}\\ = - \sqrt{\frac{(25-9)}{25}}\\ = - \sqrt{\frac{16}{25}}\\ = - \frac{4}{5}$
$\\ tan x = \frac{sin x}{cos x}\\ = \frac{\frac{3}{5}}{\frac{-4}{5}}\\ = \frac{3}{5} × \frac{-5}{4}\\ = -\frac{3}{4}$

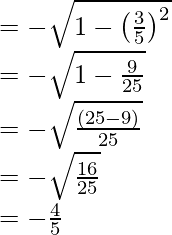
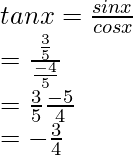
We know that sec y = $- \sqrt{1+tan^2 y}$
$\\ = - \sqrt{1 + \left(\frac{1}{2}\right)^2}\\ = - \sqrt{1 + \frac{1}{4}}\\ = - \sqrt{\frac{(4+1)}{4}}\\ = - \frac{\sqrt{5}}{2}$

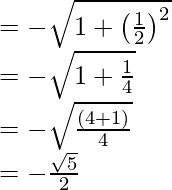
 8tan x – √5 sec y = 8 × (-3)/(4) – √5 × (-√5/2) = -6 + (5/2) = (-12 + 5)/2 = -7/2 

8tan x – √5 sec y = -7/2

Hence, the value of 8 tan x – √5 sec y = -7/2

问题4.如果sin x + cos x = 0且x位于第四象限，则求出sin x和cos x。

解决方案：

Given, sin x + cos x = 0 and x lies in fourth quadrant.

sin x = -cos x

sin x/cos x = -1

So, tan x = -1 (since, tan x = sin x/cos x)

cos x and sec x are positive in fourth quadrant and 

all other ratios are negative.

So, by using the formulas,

sec x = $\sqrt{1 + tan^2\ x}$

cos x = 1/sec x

sin x = $- \sqrt{1- cos^2 x}$

sec x = $\sqrt{1 + tan^2 x}$

$= \sqrt{1 + (-1)^2}\\ = \sqrt2\\ cos x = \frac{1}{sec\ x}\\ = \frac{1}{\sqrt2}\\ sin\ x = - \sqrt{1 - cos^2 x)}\\ = - \sqrt{1 - \left(\frac{1}{\sqrt2}\right)^2}\\ = - \sqrt{1 - \frac{1}{2}}\\ = - \sqrt{\frac{(2-1)}{2}}\\ = - \sqrt{\frac{1}{2}}\\ = -\frac{1}{\sqrt2}$

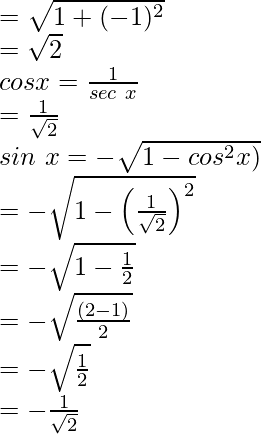
Hence, the value of sin x = -1/√2 and cos x = 1/√2 

问题5.如果cos x = -3/5且π

解决方案：

Given, cos x = -3/5 and π

tan x and cot x are positive in the third quadrant and all other rations are negative.

Now, by using the formulas, we get

sin x = – $\sqrt{1-cos^2 x}$

tan x = sin x/cos x     

cot x = 1/tan x 

sec x = 1/cos x 

cosec x = 1/sin x 

sin x = $- \sqrt{1-cos^2 x}$
$\\ = - \sqrt{1-\left(\frac{-3}{5}\right)^2}\\ = - \sqrt{1-\frac{9}{25}}\\ = - \sqrt{\frac{(25-9)}{25}}\\ = - \sqrt{\frac{16}{25}}\\ = - \frac{4}{5}$

tan x = $\frac{sin x}{cos\ x}$
$\\ = \frac{\frac{-4}{5}}{\frac{-3}{5}}\\ = \frac{-4}{5} × \frac{-5}{3}\\ = \frac{4}{3}$

cot x = $\frac{1}{tan\ x}$
$\\ = \frac{1}{\frac{4}{3}}\\ = \frac{3}{4}$

sec x = $\frac{1}{cos\ x}$
$\\ = \frac{1}{\frac{-3}{5}}\\ = \frac{-5}{3}$

cosec x = $\frac{1}{sin\ x}$
$\\ = \frac{1}{\frac{-4}{5}}\\ = \frac{-5}{4}$

Now we evaluate:

$\frac{cosec\ x+cot\ x}{sec\ x-tan\ x}$ $= \frac{\left[\frac{-5}{4} + \frac{3}{4}\right] }{ \left[\frac{-5}{3} - \frac{4}{3}\right]}$
$\\ = \frac{\left[\frac{(-5+3)}{4}\right] }{ \left[\frac{(-5-4)}{3}\right]}\\ = \frac{\frac{-2}{4} }{ \frac{-9}{3}}\\ = \frac{-1}{\frac{2} {-3}}\\ = \frac{1}{6}$