线是没有厚度的直的一维图形。在几何图形中,一条线在两个方向上无限延伸。它被描述为任意两点之间的最短距离。 A线也可以理解为沿一个特定方向彼此连接的多个点,它们之间没有间隙。
水平线
平行于X轴(或垂直于Y轴)的任何线都称为水平线。
Examples of horizontal line:
(a) y = 4.3
(b) y = k, where k is any constant
(c) y = 0, this is the equation of X-axis
水平线的斜率
水平线和X轴之间的角度为0°。因此,任何水平线的斜率= tan(0°)= 0
Slope of Horizontal line = 0
垂线
平行于Y轴(或垂直于X轴)的任何线都称为垂直线。
Examples of vertical line:
(a) x = 10
(b) x = k, where k is any constant
(c) x = 0, this is the equation of Y-axis
垂直线的斜率
垂直线和X轴之间的角度为90°。因此,任何垂直线的斜率= tan(90°)(未定义)。
Slope of vertical line is not defined.
x截距和y截距
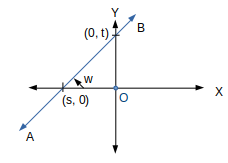
X截距
线与x轴的交点称为x截距。
例子:
In figure s is the x-intercept of line AB.
General form of x-intercept is (s, 0)
截距
线与y轴的交点称为y截距。
例子:
In figure, t is the y-intercept of line AB.
General form of y-intercept is (0, t)
从线方程截取
如果直线的方程式具有以下形式,则任何一条线,例如AB,其x截距为p,y截距为q。
(x/p) + (y/q) = 1
x和y截距的样本问题
问题1:找到具有以下等式的线的x和y截距:y = x + 10
解决方案:
Converting the equation of the given line in intercept form:
y – x = 10
(y/10) – (x/10) = 1, ———dividing both sides by 10
(y/10) + (-x/10) = 1
(x/(-10)) + (y/10) = 1,
Thus, x-intercept is -10 and y intercept is 10.
Another solution:
x-intercept is of the form (s, 0).
Let us put y = 0 in the equation of the given line:
0 = x + 10 or
x = -10
Thus, x-intercept of given line is -10.
y-intercept is of the form (0, t).
Let us put x = 0 in the equation of the given line:
y = 0 + 10 or
y = 10
Thus, y-intercept of given line is 10.
问题2:找到具有等式的直线的x和y截距:20y = 10 – 40x
解决方案:
Converting the equation of the given line in intercept form :
20y + 40x = 10
(20y/10) + (40x/10) = 1, ———dividing both sides by 10
(2y/1) + (4x/1) = 1
(x/(1/4)) + (y/(1/2)) = 1,
Thus, x-intercept is (1/4) and y intercept is (1/2).
Another solution:
x-intercept is of the form (s, 0).
Let us put y = 0 in the equation of the given line:
20*(0) = 10 – 40x, or
0 + 40x = 10, or
x = 1/4
Thus, x-intercept of given line is 1/4 or 0.25
y-intercept is of the form (0, t).
Let us put x = 0 in the equation of the given line:
20y = 10 – 40*(0)
20y = 10, or
y = 1/2
Thus, y-intercept of given line is 1/2 or 0.5
问题3:找到具有等式的线的x和y截距:4x + 5y = -3
解决方案:
Converting the equation of the given line in intercept form :
4x + 5y = -3 ——-given
4x/(-3) + 5y/(-3) = -3/(-3), —–dividing both sides by -3
x/(-3/4) + y/(-3/5) = 1,
Thus, x-intercept is (-3/4) and y intercept is (-3/5)
Another solution:
x-intercept is of the form (s, 0).
Let us put y = 0 in the equation of the given line:
4x + 5*(0) = -3, or
4x + 0 = -3, or
x = -3/4
Thus, x-intercept of given line is -3/4
y-intercept is of the form (0, t).
Let us put x = 0 in the equation of the given line:
4*(0) + 5y = -3, or
0 + 5y = -3, or
y = -3/5
Thus, y-intercept of given line is -3/5
问题4:线AB的x截距=0。找到它的y截距。
解决方案:
x-intercept of given line is 0.
This means that the point of intersection of the given line and X-axis is (0, 0).
In other words, the given line passes through the origin.
Thus, y-intercept of the given line is 0 (as the point of intersection of the given line and Y-axis is also (0, 0)).
问题5:一条线穿过点(3,4),(p,q)和(c,d),其中p和d分别是x和y截距。给定直线的斜率为-1/2 ,找到p,q,c和d的值。
解决方案:
p is the x-intercept of the given line, so (p, q) lies on X-axis.
This means that q = 0 ——–(i)
d is the y-intercept of the given line, so (c, d) lies on Y-axis.
This means that c = 0 ——–(ii)
Slope of any line = (y2 – y1)/(x2 – x1), where (x1, y1) and (x2, y2) are two points that lie on it.
Slope of given line = (4-q)/(3-p), or
-1/2 = (4-0)/(3-p), ——from (i)
(-1)*(3-p) = 4*2, or
p – 3 = 8, or
Thus, p = 11 ——–(iii)
Slope of given line = (4-d)/(3-c), or
-1/2 = (4-d)/(3-0), ——-from (ii)
(-1/2)*3 = 4 – d, or
d – 3/2 = 4, or
d = 4 + 3/2
Thus d = 11/2 or 5.5 ——–(iv)
Thus, the values are : p = 11, q = 0, c = 0, d = 11/2
从表中截取一行
General form of x-intercept is (s, 0), where s is any real number.
Thus, the point which has ordinate 0 is the x-intercept.
General form of x-intercept is (t, 0), where t is any real number.
Thus, the point which has x-coordinate 0 is the y-intercept.
样本问题
牢记以上几点,让我们解决以下问题:
问题1:找到通过以下点的直线的x轴截距和y轴截距:
| x | 8 | 4 | -8 | 0 | -12 |
| y | -5 | 0 | 15 | 5 | 20 |
解决方案:
The point (4, 0) lies on the x-axis. Thus, x-intercept is 4.
The point (0, 5) lies on the y-axis. Thus, y-intercept is 5.
问题2:找到通过以下点的直线的x轴截距和y轴截距:
| x | 1 | 2 | -1/2 | 4 |
| y | 1 | 1/3 | 2 | -1 |
解决方案:
No point in the table is such that it has x-coordinate = 0 or y-coordinate = 0.
So, let us find the equation of the line using two point form :
y – y1 = (y2 – y1)/(x2 – x1) * (x – x1), where (x1, y1) and (x2, y2) are two points.
We can take any two points from the table.
Let us take the points (1, 1) and (4, -1) for ease of calculation. Equation of given line is :
y – 1 = (-1-1)/(4-1) * (x – 1) or
3y – 3 = -2x + 2 or
2x + 3y = 2 + 3 or
2x + 3y = 5,
2x/5 + 3y/5 = 5/5, ——dividing both sides by 5
x/(5/2) + y/(5/3) = 1, which is the equation of given line in intercept form
Thus, x-intercept of given line is 5/2 or 2.5 and y-intercept of given line is 5/3.