二变量线性方程的形式–直线| 11年级数学

线是最简单的几何形状。它没有端点，并且在两个方向上一直延伸到无穷大。 “直线”一词仅表示没有“弯曲”。线上任意两点之间的梯度是相同的。因此， 我们可以说，如果线上任意两点之间的梯度相同，那么轨迹就是一条直线。因此，让我们看看该行中可以表示的不同方式是什么。

线的不同形式

• Point Slope Form
• Two – Point Form
• Slope – Intercept Form
• Intercept Form
• Normal Form

点–坡度形式

通过点(x ₁ ，y ₁ )和斜率’m’的直线方程可写为：

$y - y_{1} = m\left ( x - x_{1} \right )$

证明：

Let the point that lies on the curve be (x₁, y₁) and any general point can be denoted by (h, k).

So, the slope of the line can be written as 

Slope=(k-y₁) / (h-x₁)

Also, we know that the slope of line is m.

Hence,

(k-y₁) / (h-x₁)= m

Replace k by y and h by x

And, we get y – y₁  = m(x – x₁ )

例1.写出通过(5,6)且斜率等于3的直线的方程式

解决方案：

Putting the value of (x1,y1) as (5,6) and m = 3, we get

 y – 6 = 3* (x -5)

 y – 6 = 3x – 15

 y = 3x – 9 

例2：写出通过(0,0)且斜率等于1的直线的方程式

解决方案：

Putting the value of (x1,y1) as (0,0) and m=1, we get

 y – 0 = 1* (x -0)

 y = x   

两点式

通过点(x ₁ ，y ₁ )和(x ₂ ，y ₂ )的直线方程可写为：
$y - y_{1} = \left ( \frac{y_{2} - y_{1}}{x_{2} - x_{1}} \right )\left ( x - x_{1} \right )$

证明：

Since we know any two points on the line, we can write the slope of the line as 

m = (y₂-y₁) / (x₂-x₁)

And we also know from point slope form that 

y-y₁ = m(x-x₁) 

Substituting the value of m in the above equation, we get

y-y₁ = ((y₂-y₁) / (x₂-x₁) * (x-x₁)

示例1.编写通过(5,6)和(6,7)的直线的方程式

解决方案：

 Putting the value of (x1,y1) as (5,6) and (x2,y2) as (6,7) , we get

 y – 6 = (7-6)/(6-5) * (x -5)

 y – 6 = 1* (x – 5)

 y = x + 1

示例2.写出通过(0,5)和(5,5)的直线的方程式

解决方案：

Putting the value of (x1,y1) as (0,5) and (x2,y2) as (5,5), we get

 y – 5 = (5-5)/(5-0) * (x -0)

 y = 0

坡度-截距形式

斜率为’m’且在y轴上截取为’c’的直线方程可写为：
$y = mx + c$

证明：

Since y-intercept=c

Equation of line passing through (0,c) is given by

y – c = m(x-0)

y = mx + c

示例1.将斜率= 5且y截距为3的直线的方程写成

解决方案：

 Putting the value of m=5 and c=3 in Equation y = mx + c 

  y = 5x+3

例2编写斜率= 1且y截距为1的直线的方程

解决方案：

Putting the value of m=1 and c=1 in Equation y = mx + c

 y = x+1

拦截形式

x轴上的线切割截距’a’和y轴上的’b’的方程可写为：
$\frac{x}{a} + \frac{y}{b} = 1$

证明：

So we know that the line passes through the point (a,0) and (0,b), we can write the equation using two point form

y – y₁ = (y₂ – y₁) / (x₂ – x₁) * (x – x₁)

Putting x₁ = a, y₁ = 0, x₂ = 0 , y₂ = b

We get,

y = (b/(-a) * (x-a)

Dividing both sides by b and simplify RHS we get

y/b = -(x/a)+1

⇒ x/a + y/b =1

示例1.编写以x截距为5且y截距为3的直线的方程式

解决方案：

Putting the value of a=5 and b=3 in Equation x / a + y / b = 1 

 x/5 + y/3 =1

示例2.写出x截距为1且y截距为1的直线的方程式

解决方案：

Putting the value of a=1 and b=1 in Equation x / a + y / b = 1 

x/1 + y/1 =1

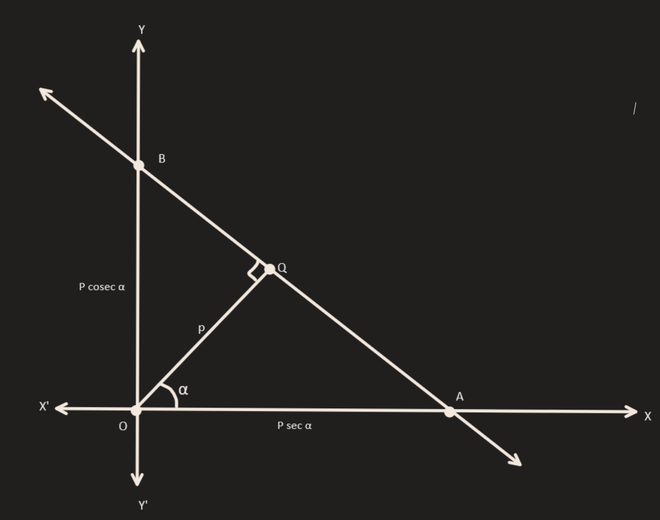
正常形式

垂直于原点的垂直线的长度为’p’且与x轴的正方向形成角度’∝’的直线方程为：
$xcos\alpha + ysin\alpha = p$

证明：

Let the line AB be such that the length of the perpendicular OQ from the origin O to the line be p and

From the diagram, using the intercept form we get

Equation of line AB is

x/p sec∝ + y/p cosec∝ =1

or

xcos∝ + ysin∝ =p 

示例1.编写一条直线方程，其直线从原点开始的长度为5个单位，并且该直线与x轴的正方向成^{45 0的角度。}

解决方案：

So, basically we are given the value of p=5 and ∝=45⁰

Putting the values in the above equation we get, 

x /√2 + y√2 = 5

x + y = 5√2

示例2.编写一条直线方程，其直线与原点的垂直线长度为1个单位，并且该垂直线与x轴的正方向形成^{60 0的角度。}

解决方案：

So, basically we are given the value of p=1 and ∝=60⁰

Putting the values in the above equation we get,

x * (1/2) + y * (√3/2) = 1

x + y√3 = 2