点坡形式–直线| 11年级数学

有几种形式可以表示二维坐标平面上的直线方程。它们的三个主要形式是点斜率形式，斜率截取形式以及常规形式或标准形式。点斜率形式包括直线的斜率和直线上的一个点，顾名思义。可以有无限条具有给定斜率的直线，但是当我们指定直线经过给定点时，我们将获得一条唯一的直线。因此，仅需要线上的一个点及其斜率即可表示点-斜率形式的直线。

点斜率方程

考虑二维坐标平面上的任何直线。令其斜率为m ， (x ₁ ，y ₁ )为线上的固定点。可以有无限个斜率m的直线，但是当我们指定直线穿过固定点(x ₁ ，y ₁ )时，我们得到一条唯一的直线。令(x，y)为线上的任意点。我们知道线的斜率是m ，即线上的任意两个点之间的斜率始终是m。利用这一事实，我们可以得出具有给定斜率并经过固定点的任何直线的方程式。

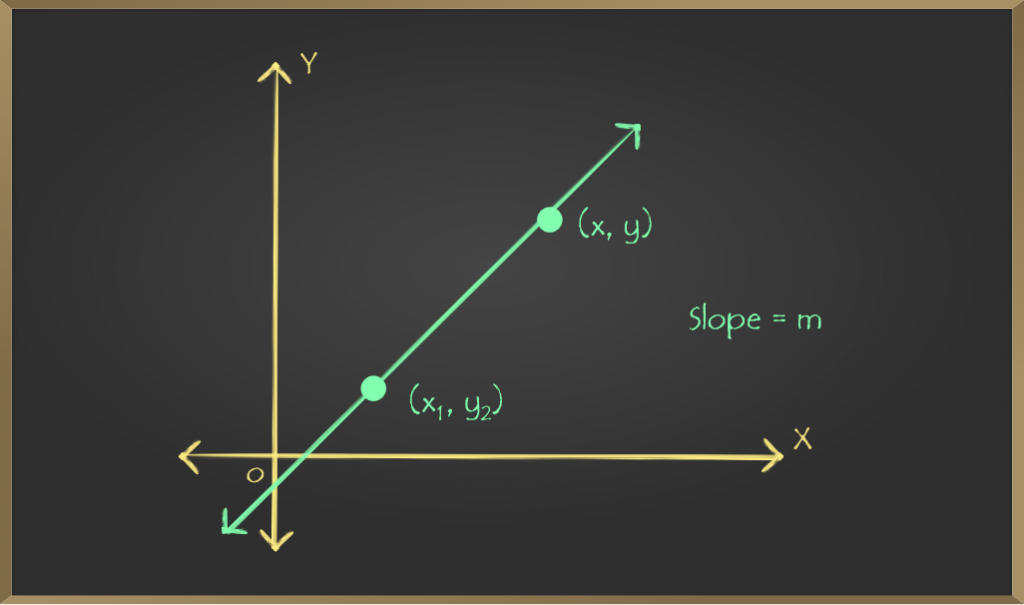
我们知道，

(y的变化)/(x的变化)=斜率，

由于(x，y)和(x ₁ ，y ₁ )是直线上的两个点，并且m是直线的斜率，因此(x，y)和(x ₁ ，y ₁ )之间的斜率将等于m 。

现在，在(x，y)和(x ₁ ，y ₁ )之间的斜率=(y – y ₁ )/(x – x ₁ )

∴(y − y ₁ )/(x − x ₁ )= m…(1)

将等式(1)的两边乘以(x − x ₁ )，我们得到，

⟹ (y-y ₁ )= m(x-x ₁ ) …(2)

这是所考虑的直线或点斜率形式的方程式。

该方程称为点斜率形式的原因非常明显。该方程只包含斜率和直线上的固定点作为常数，因此称为点斜率形式。

点斜率方程的样本问题

问题1：找到斜率为3且直线为0的直线方程
通过(-1，5)。

解决方案：

Here slope of the line is 3 or m = 3 and the line passes through (-1, 5),

hence a fixed point on the line is

(-1, 5) or (x₁, y₁) = (-1, 5)

Let (x, y) be any point on the line

∴ Slope between (x, y) and (x₁, y₁) = (y – y₁) / (x – x₁)

Since (x₁, y₁) and (x, y) are two points on the line,

the slope between them will be the slope of the line (m)

∴ (y – y₁) / (x – x₁) = m

Multiplying both sides by (x – x₁) we get,

⇒ y – y₁ = m(x – x₁)

Putting m = 3 and (x₁, y₁) = (-1, 5) we get,

⇒ y – 5 = 3(x – (-1))

⇒ y – 5 = 3(x + 1)

⇒ y – 5 = 3x + 3

⇒ y – 3x – 5 – 3 = 0

⇒ y – 3x – 8 = 0, which is the required equation 

问题2：找到斜率为-2并经过(7，-4)的直线方程。

解决方案：

Here slope of the line is -2 or m = -2 and the line passes through (7, -4), hence a fixed point on the

line is (7, -4) or (x₁, y₁) = (7, -4)

Let (x, y) be any point on the line

Since (x₁, y₁) and (x, y) are two points on the line, the

slope between them will be the slope of the line

∴ (y – y₁) / (x – x₁) = m

Putting m = 3 and (x₁, y₁) = (7, -4) we get,

⇒ (y – (-4)) / (x – 7) = -2

Multiplying both sides by (x – 7) we get,

⇒ y + 4 = (-2)(x – 7)

⇒ y + 4 = -2x + 14

⇒ y + 2x + 4 – 14 = 0

⇒ y + 2x – 10 = 0, which is the required equation 

问题3：找到斜率为1/4并经过(2，3)的直线方程。

解决方案：

Here slope of the line is 1/4 or m = 1/4 and the line passes through (2, 3), 

hence, a fixed point on the line is (2, 3) or (x₁, y₁) = (2, 3)

We know, point-slope form is:  (y – y₁) = m(x – x₁)

Putting the values of m, x₁ and y₁ in the equation we get,

⇒ (y – 3) = (1/4)(x – 2)

Multiplying both sides by 4 we get,

⇒ 4(y – 3) = 1(x – 2)

⇒ 4y – 12 = x – 2

⇒ 4y – x – 12 + 2 = 0

⇒ 4y – x – 10 = 0, which is the required equation

坡度截距方程

斜率截距形式可以视为点斜率形式的特例，其中直线上的固定点位于X轴或Y轴上。当一条直线穿过任何一条轴时，它将在距原点一定距离的固定点处切割该轴。该距离称为线的截距。因此，如果给出了截距，我们可以轻松确定切点并将其用作点-坡度形式的固定点。如果说这条线是在距原点c ₁的距离上切割Y轴的，那么很明显，切割点在Y轴上，并且坐标是(0，c ₁ ) ，它将是固定的。点斜率形式的点。类似地，如果线相交上的C ₂的从原点的距离X轴，切割点在X轴和其坐标将是(C _2,0)。请参见下图以进一步说明。

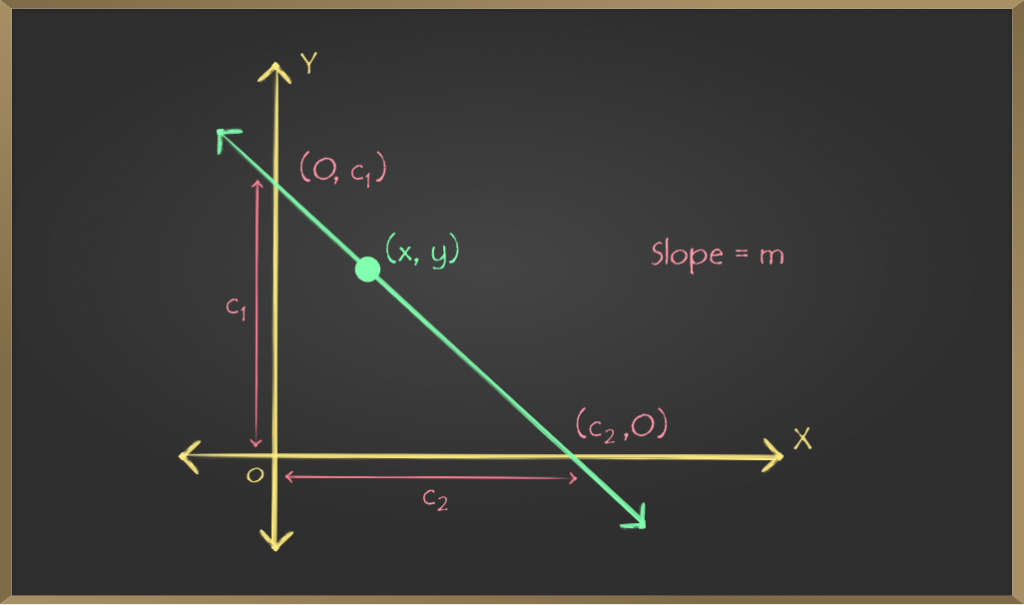
取决于线是从哪个轴切出截距，这两种情况如下：

情况1：如果给出了y截距

考虑一个斜率为m的直线，该直线被称为在距原点c ₁的距离处切开Y轴。如上所述，该线在固定的切割点(0，c ₁ )上切割Y轴。将斜率= m ， x ₁ = 0且y ₁ = c ₁设为点斜率形式，

(y – y₁) = m (x – x₁)

Putting the values we get,

⇒ y – c₁ = m (x – 0)

⇒ y – c₁ = mx

⇒ y = mx + c₁               …(3),

这是所考虑的线或斜率截距形式的方程式。

问题1：找到斜率为-1且y截距为3的直线方程。

解决方案：

Here slope of the line is -1 or m = -1 and

y-intercept is 3, hence the line cuts the Y-axis on the

fixed point (0, 3) or (x₁, y₁) = (0, 3)

Putting the values of m, x₁ and y₁ in the point-slope

form we get,

Point-slope form: (y – y₁) = m(x – x₁)

⇒ y – 3 = (-1)(x – 0)

⇒ y – 3 = (-1)x

⇒ y – 3 = -x

⇒ y + x – 3 = 0, which is the required equation

问题2：找到斜率为4且y截距为-5的直线方程

解决方案：

Here slope of the line is 4 or m = 4 and

y-intercept is -5 or c₁ = -5

Putting the values of m and c₁ in the slope-intercept

form we get,

Slope-intercept form: y = mx + c₁

⇒ y = 4x + (-5)

⇒ y = 4x – 5

⇒ y – 4x + 5 = 0, which is the required equation

情况2：如果给出了x截距

考虑一个斜率为m的直线，该直线被称为在距原点c ₂的距离处切开了X轴。如上所讨论的，线切割X轴在一个固定的切削点，它是(C _2，0)。推杆坡度= m， x ₁ = c ₂和y ₁ = 0的点斜率形式，

(y – y₁) = m (x – x₁)

Putting the values we get,

⇒ y – 0 = m (x – c₂)

⇒ y = m (x – c₂)               …(4),

这是所考虑的线或斜率截距形式的方程式。

问题1：找到斜率为2和x截距为1的直线的方程式

解决方案：

Here slope of the line is 2 or m = 2 and

x-intercept is 1, hence the line cuts the X-axis on the

fixed point (1, 0) or (x₁, y₁) = (1, 0)

Putting the values of m, x₁ and y₁ in the point-slope

form we get,

Point-slope form: (y – y₁) = m(x – x₁)

⇒ y – 0 = 2(x – 1)

⇒ y = 2(x – 1)

⇒ y = 2x – 2

⇒ y – 2x + 2 = 0, which is the required equation

问题2：找到斜率为-3和x截距为-7的直线方程

解决方案：

Here slope of the line is -3 or m = -3 and

x-intercept is -7 or c₂ = -7

Putting the values of m and c₂ in the slope-intercept

form we get,

Slope-intercept form: y = m(x – c₂)

⇒ y = (-3)(x – (-7))

⇒ y = (-3)(x + 7)

⇒ y = -3x – 21

⇒ y + 3x + 21 = 0, which is the required equation

概括

1. 具有斜率m且经过固定点(x ₁ ，y ₁ )的直线的方程，即点斜率形式为： (y-y ₁ )= m(x-x ₁ )
2. 具有斜率m和y截距c ₁的直线的方程，即斜率截距形式为： y = mx + c ₁
3. 具有斜率m和x截距c ₂的直线的方程，即斜率截距形式为： y = m(x – c ₂ )