精确方程和积分因子

微分方程用于描述许多物理现象。它们帮助我们观察现实生活中发生的事情，并将其以数学形式表示。在此级别上，我们主要关注线性和一阶微分方程。如果y的所有导数仅出现一次幂，则“ y”中的微分方程为线性。因此，它具有以下形式：y的所有导数仅出现在一次幂上。它的形式如下

$A_{n}y^{n}+A_{n-2}y^{n-1}+...+A_{1}y'+A_{0}y = f$

允许系数仅取决于自变量。注意，该顺序可以任意大。我们将看一下精确的微分方程。这些方程是一阶微分方程。

精确的微分方程

它是一阶微分方程，看起来像

M(x，y)dx + N(x，y)dy = 0

它有一个特殊的函数I(x，y)，它的偏导数可以代替M和N，例如，

$\frac{\partial{I}}{\partial{x}}dx + \frac{\partial{I}}{\partial{y}}dy = 0$

解决这些方程式意味着找到函数I(x，y)=C。

检查方程是否正确：

假设有一个求解函数I(x，y)。然后，

$\frac{\partial{M}}{\partial{y}} = \frac{\partial^{2}{I}}{\partial{x}\partial{y}}{}$

$\frac{\partial{N}}{\partial{x}} = \frac{\partial^{2}{I}}{\partial{x}\partial{y}}{}$

他们最终应该一样。因此，要检查方程是否正确，只需计算这些偏导数即可。因此，既然我们已经检查了方程的正确性，我们将继续寻找该方程的解。

计算精确微分方程的解：

通用解可以通过以下两种方式之一进行计算：

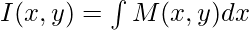1. $I(x,y) = \int M(x,y)dx$ (以x为自变量)
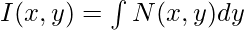2. $I(x,y) = \int N(x,y)dy$ (以y为自变量)

这样，一般的解决方案就变成了

I(x，y)= C

样本问题

问题1：求解：(3x ² y ³ – 5x ⁴ )dx +(y + 3x ³ y ² )dy = 0

解决方案：

Here, M(x, y) = 3x²y³ – 5x⁴ and N(x, y) = y + 3x³y²  

First check if this equation is exact or not, 

$\frac{\partial{M}}{\partial{y}} = 6x^{2}y^{2} \\ \frac{\partial{N}}{\partial{x}} = 6x^{2}y^{2}$

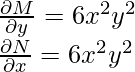
Since both of these are same, the equation is exact. 

Now let’s find the solution of this equation,

I$I(x,y) = \int M(x,y)dx \\ \hspace{1.1cm}= \int (3x^{2}y^{3} - 5x^{4})dx \\ \hspace{1.1cm}= x^{3}y^{3} - x^{5} + f(y)$

Notice that we are writing f(y) in place constant of integration “C”, because we had y as a fixed parameter while integrating it, but we know that is a variable, so we need to calculate f(y). 

We know, $\frac{\partial{I}}{\partial{y}} = N(x,y)\\ 3x^{3}y^{2} + \frac{df}{dy} = y + 3x^{3}y^{2} \\ \frac{df}{dy} = y \\ df = ydy \\ \int df = \int ydy \\ f = \frac{y^{2}}{2} + C$

Let’s plug in f(y) in the general solution, 

$I(x,y) =x^{3}y^{2} - x^{5} + \frac{y^{2}}{2} + C$

This is the general solution for this exact differential equation. 

问题2：针对以下问题求解微分方程：

(x ² + 3y ² )dy + 2xydx = 0

解决方案：

 M(x, y) = 2xy, N(x, y)=x²+3y²

$\frac{dM}{dy}= 2x\\\frac{dN}{dx}=2x$

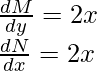
Therefore, the equation is exact.

Solution of this equation: I(x, y)=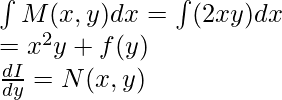$\int M(x,y)dx= \int(2xy)dx\\=x^2y+f(y)\\ \frac{dI}{dy}=N(x,y)$ 

Substituting I(x, y) obtained:

$\frac{d(x^2y+f(y))}{dy}=x^2+3y^2\\ x^2+ \frac{df}{dy}=x^2+3y^2\\\frac{df}{dy}=3y^2\\df=\int3y^2dy\\f=y^3+C$

Put the value of f(y) in I(x, y):

I(x, y)= x²y+y³+C

This is the general solution for the exact differential equation.

问题3：求解^y dx +(2y + xe ^y )dy = 0

解决方案：

M(x, y) = e^y, N(x, y) = (2y+xe^y)

Solving $\frac{dM}{dy},\frac{dN}{dx}$

$\frac{dM}{dy}=e^y,\frac{dN}{dx}=e^y$

Hence, the given equation is exact equation.

Solution of the equation: I(x, y) = $\int M(x,y)dx= e^ydx=xe^y+f(y)$

It is known that dI/dy = N(x, y)

Substituting I(x, y):

$\frac{d(xe^y+f(y))}{dy}=2y+xe^y\\xe^y+\frac{df}{dy}=2y+xe^y\\\frac{df}{dy}=2y\\f=\int2ydy\\f=y^2+c$

Therefore, the solution for the exact equation obtained is,

I(x, y) = xe^y + y² + C

整合因子

这也是求解线性微分方程的另一种方法。

$\frac{dy}{dx} + Py = Q$

上式表示线性一阶微分方程。 P和Q是x的常数或函数。以下步骤概述了如何使用积分因子求解这种形式的一阶线性方程。

步骤1：以形式写给定的微分方程$\frac{dy}{dx} + Py = Q$ ，其中P和Q只是常数或x的函数。

步骤2：找出整合因素$I.F = e^{\int Pdx}$

步骤3：将微分方程的解写为$y(I.F) = Q(I.F)dx + C$

Note: In case, the first-order differential equation is in the form $\frac{dx}{dy} + P_{1}x = Q_{1}$, where P₁ and Q₁ are constants or functions of y only. Then $I.F = e^{\int P_{1}dx}$ and the solution of the differential equation is given by $x.(I.F) = \int (Q_{1} \times I.F)dy + C$ 

样本问题

问题1：找到以下方程式的一般解，

$\frac{dy}{dx} - y = cosx$

解决方案：

The equation matches the form given above, so here P = -1 and Q = cosx.

Therefore, $I.F = e^{\int -dx} = e^{-x}$

Multiplying both sides of the equation by I.F.

$e^{-x}\frac{dy}{dx} - e^{-x}y = e^{-x}cosx \\ (\frac{dy}{dx})(ye^{-x}) = e^{-x}cosx \\$

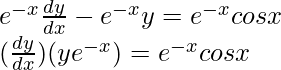
Integrating both sides of the equation by I.F, we get 

$ye^{-x} = \int e^{-x}cosxdx + C \\ \text{Let, } \\ I = \int e^{-x}cosxdx\\ = cosx(-e^{-x}) - \int (-sinx)(e^{-x})dx \\ = -cosx(e^{-x}) - \int sinxe^{-x}dx \\ = -cosxe^{-x} + sinxe^{-x} - \int cosxe^{-x} \\ I = -cosxe^{-x} + sinxe^{-x} - I \\ 2I = (-cosxe^{-x} + sinxe^{-x})\\ I = \frac{(-cosxe^{-x} + sinxe^{-x})}{2}$

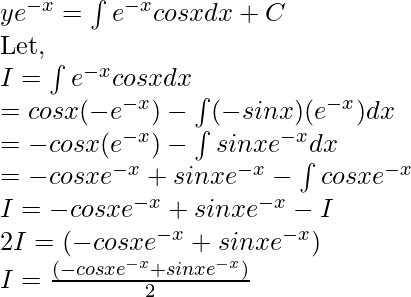
Putting this value in the original equation, 

$-ye^{-x} = \frac{(-cosxe^{-x} + sinxe^{-x})}{2}e^{-x} + C \\ y = \frac{sinx - cosx}{2} + Ce^{x}$

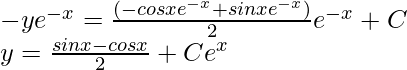
This is the general solution of the given differential equation. 

问题2：找到微分方程的一般解，

$\frac{dy}{dx}-\frac{3y}{x+1}=(x+1)^4$

解决方案：

 P= -3/(x+1), Q=(x+1)⁴

First, find the I.F.,

I.F.=$e^{\int\frac{-3}{x+1}dx}\\e^{-3ln(x+1)}\\e^{ln(x+1)^{-3}}=\frac{1}{(x+1)^3}$

Multiply by Integrating factor on both sides:

$\frac{1}{(x+1)^3}\frac{dy}{dx}-\frac{3y}{(x+1)^4}=(x+1)$

Integrate both sides:

$\frac{y}{(x+1)^3}=0.5x^2+x+c$

Hence, the general equation is:

y = (x+1)3(0.5x²+x+c)

问题3：微分方程的一般解是?

$\frac{dy}{dx}+\frac{3y}{x}=\frac{e^x}{x^3}$

解决方案：

First, find the I.F’

P = 3/x, Q = e^x/x³

I.F = $e^{\int\frac{3}{x}dx}=e^{3lnx}=e^{lnx^3}=x^3$

Therefore, I.F = x³

Multiply Integrating factor on both sides,

$x^3\frac{dy}{dx}+3x^2y=e^x$

Integrate both sides,

$x^3y=e^x+c\\y=\frac{e^x+c}{x^3}$

特殊整合因素

到目前为止，我们已经了解了关于精确微分方程的所有知识以及如何求解它们，但是有时我们会遇到某些方程“几乎精确”但不是精确的微分方程，因为它们不是精确的，而是通过做一些事而由精确微分方程形成的。他们的变化。如果我们可以追溯并再次使方程式精确，则该问题将很容易解决。

为了追溯到精确积分因子，可将方程式与一个称为“特殊积分因子”的乘积相乘。 $\mu(x)$或者$\mu(y)$ (取决于问题陈述)。

仅使用x的积分因子

假设给定的几乎精确的方程M(x，y)dx + N(x，y)dy = 0 [几乎精确]

让我们乘以特殊的积分因子$\mu(x)$

$\mu(x)$ ×M(x，y)dx + $\mu(x)$ ×N(x，y)dy = 0

现在，为了使上述方程式精确，必须具有以下值： $\mu(x),$特殊积分因子的公式，

$\mu(x)=e^{\int{\frac{dM/dy-dN/dx}{N}dx}}$

问题：求解微分方程，(1 + y ² )dx + xydy = 0

解决方案：

To begin with, we first need to check the exactness of the differential equation

dM/dy = 2y, dN/dx = y

Now, as the equation is not exact, we need to find out the integration factor that is needed to be adjusted in the equation to make it exact.

(dM/dy- dN/dx) = 2y-y = y

1/N{dM/dy- dN/dx}=y/(xy)= 1\x

Here, we figured that the equation depends on x only.

Therefore, the integrating factor is $\mu(x)$

$\frac{1}{\mu}\frac{d\mu}{dx}=\frac{1}{x}$

Separate the variables and integrate both sides,

$ln\mu= lnx$

$\mu= ^+_-x$

We obtained the value of integrating factor, multiply this with our original equation

(x+xy²)dx + x²ydy=0

Checking for exactness again,

dM/dy= 2xy, dN/dx= 2xy

Integrate M and N as usual,

M(x, y)= $\int(x+xy^2)dx=\frac{x^2}{2}+\frac{x^2y^2}{2}+f(y)$

Substitute to determine f(y)

$\frac{d(\frac{x^2}{2}+\frac{x^2y^2}{2}+f(y))}{dy}=x^2y$

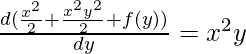
It follows f(y)=C where, C is a Constant.

Therefore, the general solution becomes,

$\frac{x^2}{2}+\frac{x^2y^2}{2}+C=0$

仅使用y的积分因子

同样，可以通过将几乎精确的方程乘以特殊的积分因子来获得精确方程$\mu(y)$ ，公式为

$\mu(y)=e^{\int{\frac{dN/dx-dM/dy}{M}dy}}$

问题：求解微分方程，(2y ² + 2y + 4x ² )dx +(2xy + x)dy = 0

解决方案：

To begin with, we need to check if the following equation is exact or not,

dM/dy = (4y +2), dN/dx = (2y +1)

dM/dy ≠ dN/dx {the equation is not exact}

In order to find the Integrating factor, solve the value of $\frac{dM/dy-dN/dx}{N}= \frac{(4y+2)-(2y+1)}{2xy+x}$

$\frac{(2y+1)}{x(2y+1)}$=1/x

Since the value obtained is purely a function of x, we can conclude that the special integrating factor is $\mu(x)$

$\mu(x)=e^{1/xdx}$= e^lnx= x

Multiply the special integrating factor with the original equation,

 x[(2y²+2y+4x²)dx + (2xy +x) dy]=0

 (2xy²+2xy+4x³)dx + (2x²y +x²) dy=0

Finding the exactness of the differential equation obtained,

dM/dy=(4xy+2x), dN/dx=(4xy+2x)

Now we can integrate M and N as usual,

$\int{M(x, y)dx}=\int{(2xy^2+2xy+4x^3)dx}=x^2y^2+x^2y+x^4+C(y)$

$\int{N(x, y)dy}=\int{(2x^2y+x^2)dy}= x^2y^2+x^2y+C(x)$

Therefore, Solution: x²y²+x²y+x⁴=C