复利|八级数学

复利是将收益再投资于您的本金以获取指数回报的过程，因为接下来的增长将在更大的本金上进行，此过程将为收益增加本金。在这段时间里，本金将成倍增长并产生不同寻常的回报。

有时，我们会遇到一些类似的表述，例如“在银行以每年11％的利率存入FD的一年利息”。或“年利率为8％的储蓄帐户”。当涉及投资时，通常有两种类型的权益：

• 单纯的兴趣
• 复利

我们已经了解简单利息(SI)，我们将在本文中详细介绍复利(CI)。首先，让我们了解一个故事在不断加剧。

A Prisoner was once awaiting his death sentence when the king asked for his last wish.

The Prisoner demanded grain of rice (foolish demand right?) but added that the number of grain should be doubled after moving to every square till the last square of the Chess Board ( that is 1 on first, 2 on second, 4 on third, 8 on fourth, 16 on fifth and so on, till the 64th square).

The king thought that it is a very small demand and ordered his ministers to have that much amount of rice calculated and provided to the prisoner. The amount calculated was so big that the king lost his entire kingdom and was indebted to prisoner all of his life.

囚犯使用的是“复合”的想法。现在，让我们定义复利。

复利

复利(或复利)是根据初始本金和前一期间的累计利息计算的贷款或存款利息。被认为起源于17世纪的意大利，复合利息可以被认为是“利息利息”，它将使总和的增长速度比仅根据本金计算的单利增长得更快。
在计算公式之前，让我们看一个示例，

问题：赫玛借了一笔卢比。每年向银行支付200万，期限为2年，年利率为8％。查找复利和她在2年末必须支付的金额。

答：

To do it, we need to find interest year by year.

Step 1. First let’s find Simple Interest for the first year,

          Here, principal P₁ = 2,00,000, R = 8% and T = 1.

          SI₁ = SI at 8% on P₁ for one year = $\frac{P_{1} \times R \times T}{100} = \frac{200000 \times 8 \times 1}{100} = 16000$

Step 2. So, now the amount received at the end of the first year = SI₁ + P₁ = 16000 + 2,00,000 = 2,16,000. Now, this will become principal.

            Thus, P₂ = 2,16,000, R = 8 and T = 1

Step 3. Now we will find simple interest for the second year by taking the total amount at the end of 1st year as principal P₂.

           SI₂ = SI at 8% on P₂ for one year =$\frac{P_{2} \times R \times T}{100} = \frac{216000 \times 8 \times 1}{100} = 17280$

           This amount now at the end of 2nd year = SI₂ + P₂ = 17280 + 2,16,000 = 2,33,280

           Total interest given = 17280 + 16000 = 33280.,

We need to notice that Principal remains the same in Simple Interest(SI), but in Compound Interest(CI) it recalculated and changes every year. 

复利计算公式

Let’s derive the formula for compound interest by taking the previous example only, but this time we will not use the values for the variables.

 $SI_{1} = \frac{P_{1} \times R}{100}$ 

Now, the amount at the end of first year will the principal for the second year, i.e

$P_{2} = A_{1} = \frac{P_{1}R}{100} + P_{1} \\ \hspace{1.35cm} = P_{1}(1 + \frac{R}{100})$

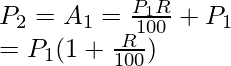
So, now SI for 2nd year

$SI_{2} = \frac{P_{2}R}{100}$

Calculating the amount for the 2nd year,

$A_{2} = P_{2} + \frac{P_{2}R}{100} \\ \hspace{0.5cm} = P_{2}(1 + \frac{R}{100})$

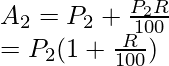
Now using the value of P2 in the above equation,

$A_{2} = P_{1}(1 + \frac{R}{100})(1+\frac{R}{100})\\ \hspace{0.5cm} = P_{1}(1 + \frac{R}{100})^{2}$

Similar if we keep calculating for “n” years,

We’ll end up with this formula of amount

$A = P(1 + \frac{R}{100})^{n}$

where P is the initial principal amount, R is the rate and n is the number of years after which the amount is calculated. 

每年或半年复利一次

您可能会注意到，一开始我们使用的是“每年复利率”。这是什么意思?

这意味着每年复利一次。我们还可以每半年或每季度增加一次利息。在这种情况下会发生什么?

让我们通过一个示例比较这两种情况，以查看每年和半年复利的比率之间的差异。

Suppose P = 1000, R = 5% and n = 2 years,

Case 1: Interest compound annually.

$I = \frac{PR}{100} = \frac{1000 \times 5}{100} = 50$

A = 50 + 1000 = 1050.

Case 2: Interest Compounded Half-Yearly.

$I = \frac{PRT}{100} = \frac{1000 \times 5 \times \frac{1}{2}}{100} = 25$

P₂ = I + P = 1025

$I_{2} = \frac{P_{2} RT }{100} = \frac{1025 \times 5 \times \frac{1}{2}}{100} = 25.625$

Final amount in this case A = P₂ + I₂ = 1025 + 25.625 = 1050.625  

如果半年复利，我们可以计算两次利息。因此时间段变为两倍，而速率变为一半。

所以公式变成

$A = P(1 + \frac{\frac{R}{2}}{100})^{2n}$

复利公式和实例的应用

增长与衰变

复利概念可以应用于任何增加或减少的数量，以使每个期间结束时的金额与该期间开始时的金额具有恒定的比率。

假设，如果某个城镇的人口在每年的年初稳定增长，并且每年增加2％，那么每年的增长因子就是$(1+\frac{2}{100})$即1.02，n年后的人口是该时期开始时人口的^{(1.02)n倍。}如果人口减少2％，则年衰减因子为$(1−\frac{2}{100}),$即0.98。

问题：城镇人口以每年2.5％的速度增长。如果其当前人口为3,26,40,000，请在3年后找到该人口。

答：

We can apply compound interest formula here,

Population “P” at the end of 3 years will be,

$P = 32640000(1+\frac{2.5}{100})^{3} \\ \hspace{0.38cm} = 32640000(1 + \frac{2.5}{100})^{3} \\ \hspace{0.38cm} = 32640000(1.025)^{3} \\ \hspace{0.38cm} = 35149710$

升值和折旧

当一件物品的价值随着时间的流逝而增加时，该物品被认为是升值的。当物品的价值随着时间的流逝而降低时，该物品就会贬值。

例如，如果一个人买了一辆汽车并使用了两年，那么很显然，买一辆新车是不值得的。因此，汽车将贬值。另一方面，如果一个人购买一块土地，他可能会发现，几年后，他将能够获得比其所支付的价格更好的价格。土地的价值将因此升值。当事情难以获得时，它们具有稀有价值和欣赏价值。

问题：以10万卢比建造的住宅单位的价值每年以10％的比率递增。施工后三年的价值是多少?

答：

Value of flat P = 1000000, rate of appreciation = 10, n = 3

After 3 years, let the value of flat be “A”.

$A = P(1 + \frac{R}{100})^{n} \\ \hspace{0.35cm} = 1000000( 1 + \frac{10}{100})^{3} \\ \hspace{0.35cm} = 1000000(\frac{11}{10})^{3} \hspace{0.35cm} = 100 \times 11 \times 11 \times 11 \\ \hspace{0.35cm} = 1331000$

So, after 3 years value of flat will be 13,31,000. 

如果细菌的生长速率已知，则其生长：

问题：在某个实验中，细菌的数量以每小时2.5％的速度增加。最初，这个数字是51,20,000。在2小时结束时找到细菌。

回答：

Initial count of bacteria = P = 51,20,000,

Increase rate “r” = 2.5 per hour,

We want to find the count after 2 hours, i.e; n = 2

Let the final count be “A”

$A = P(1 + \frac{R}{100})^{2}\\ \hspace{0.36cm} = 5120000( 1 + \frac{2.5}{100})^{2} \\ \hspace{0.36cm} = 5120000( \frac{41}{40})^{2}\\ \hspace{0.36cm} = 5379200$

So, the final count of bacteria is 53,79,200.