牛顿万有引力定律

不管苹果是否真的像某些故事所说的那样落在了艾萨克·牛顿的头上，万有引力定律给出的这个等式描述了为什么每个人都停留在地面上，是什么将地球锁定在围绕太阳的轨道上，并送人到月球。它总结了这样一个想法，即宇宙中所有物质粒子都通过引力相互吸引，牛顿定律告诉我们这种吸引有多强。让我们讨论一下该定律是如何发现和推导的。

什么是引力?

引力是一种力量，表明地球上或太空中的每个物体都相互吸引。物体上的重力取决于物体的质量，物体的质量越大，其他物体所施加的力就越大。我们可以看到的所有物体都被一定程度的彼此吸引，例如笔，橡皮，行星，手机，手表，冰箱。引力是与电磁力和核力的非接触力之一。

地球被太阳的引力吸引而绕着太阳旋转。

万有引力定律

宇宙中的每个物体都通过一个力吸引每个其他物体，该力与两个物体的质量乘积成正比，而与它们之间的距离的平方成反比。这就是所谓的万有引力定律。这具有非常有趣的历史，并且许多科学家和数学家进行了大量的手工和研究。

有一天，艾萨克·牛顿在他的大学里坐在一棵苹果树下。一个苹果从地上掉下来，他想这苹果为什么掉下来，为什么没掉下来。这种好奇心使他深受打击，于是他开始着手研究，并为苹果掉下来找到了数学上的解决方案。为此，他制定了一条法律，称为“万有引力定律” 。

Representation for Universal Law of Gravitation

According to Newton’s universal law of gravitation the gravitational force that exist between the two masses say m₁ and m₂ is directly proportional to the product of their masses and inversely proportional to the square of the distance between the two masses i.e. d.

Mathematically, both the conditions can be interpreted as:

• F ∝ m₁ × m₂
• F ∝ 1 / d²

Combining the above two conditions, it is obtained as:

• F ∝ m₁ × m₂ / d²

Now, deducing the above condition to an equation. Remove the proportionality sign by introducing the constant of proportionality.

$F=\dfrac{Gm_1m_2}{d^2}$

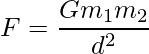
Here, G is the constant of proportionality and known as the Gravitational constant equal to 6.67 × 10 ⁻¹¹ N⋅m² / kg².

万有引力定律的意义

这一普遍的万有引力定律为进行更多的研究和寻找行星在地球上存在的活生物体而探索宇宙的途径开辟了许多道路。航天器使用此公式来计算不同的结果。重力对我们非常重要。它使我们进入绕太阳公转的轨道，并获得阳光和温暖。重力还可以保持空气和地球大气。黑洞是您可以看到引力力量的地方。黑洞吞没了所有东西，甚至将它们吞没，并将每件事物存储在它们称为“奇点”(Singularity)的尾巴中的一个很小的地方。

将我们束缚于地球的力量：

所有有生命和无生命的事物都在地球上，它们不会离开地球的大气层而掉入外层空间。即使人类已经开发出一些技术来穿透这种力量并进入太空进行探索。引力是当我们跳跃时将我们带回地球的力量。

地球向我们施加压力

月球绕地球运动：

巨大撞击理论说，月亮是地球的一部分，在地球创建的早期阶段，由于月球的引力，它脱离了地球并开始绕地球旋转。月球的公转时间和公转时间相等，这就是为什么我们只能看到它是一侧的原因。

行星绕太阳运动：

长期以来，人们一直认为太阳围绕太阳旋转是一回事。当伽利略(Galileo Galilei)说地球围绕太阳旋转时，他被勒令到圣公会进行审判，他将被监禁，并将保持孤独。甚至太阳也绕银河系绕行。完成一个轨道需要2.36亿年。我们的银河系是一个广阔的研究领域。

行星绕太阳运动

样本问题

问题1：地球的质量为6×10 ²⁴千克，月亮的质量为7.4×10 ²²千克。如果地球与月球之间的距离为3.84×10 ⁵ km，则计算地球在月球上施加的力。

解决方案：

Given that,

The mass of the earth, m₁ is 6 × 10²⁴ kg.

The mass of the moon, m₂ is 7.4 × 10²² kg. 

The distance between earth and moon, d is 3.84 × 10⁵ km.

The formula to calculate the force exerted by the earth on the moon is:

$\begin{aligned}F&=\dfrac{Gm_1m_2}{d^2}\\&=6.67\times10^{-11}\times\dfrac{6\times10^{24}\times7.4\times10^{22}}{({3.84\times10^8})^2}\\&=2.02\times10^{20} \text{ N}\end{aligned}$

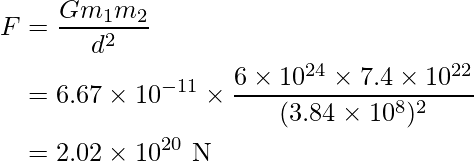
Hence, the force exerted by the earth on the moon is equal to 2.02 × 10²⁰ N.

问题2：您的老师在教您远离您5 m的万有引力定律，如果他的质量为60 kg，那么您对他施加的万有引力值是多少? (提示：自己承担体重)。

解决方案：

Given that,

The mass of the teacher, M is 60 kg.

The mass of the person, m is 70 kg.

The distance between them, d is 5 m.

The formula to calculate the gravitational force exerted by the person on the teacher is:

$\begin{aligned}F&=\dfrac{GMm}{d^2}\\&=6.67\times10^{-11}\times\dfrac{60\text{ kg}\times70\text{ kg}}{(5\text{ m})^2}\\&=1.1\times 10^{-9}\text{ N}\end{aligned}$

Hence, the gravitational force exerted by the person on the teacher is 1.1 × 10^-9 N.

问题3：卫星A的质量是卫星B的两倍。卫星A绕卫星B的半半径绕地球旋转。与地球上的B卫星相比，A卫星上的力是多少倍?

解决方案：

Consider the mass of B satellite as m and the mass of A satellite as 2m.

Now, to calculate gravitational force for both satellite divide both forces to obtain an equation as:

$F_{A}= G \times \dfrac{M_{earth}\times 2m}{R^2}\\~\\ F_{B}= G \times \dfrac{M_{earth}\times m}{(2R)^2}\\~\\$

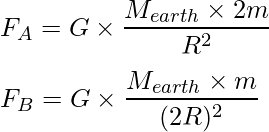
Now divide the force on A by Force on B as:

$F_{A} = 8\times F_{B}$

Satellites orbiting earth

Hence, the force on satellite A is 8 times the force on satellite B.

问题4：牛顿的万有引力定律适用于：

一种。仅小型

b。仅植物

C。所有身体，不论大小

d。用于太阳能系统

解决方案：

The Newton’s law of gravitation is applicable to all bodies irrespective of their size.

Hence, Option c. is correct.

问题5：考虑两个物体接触，使得它们之间的距离是通常距离的六倍。计算力的变化量。

解决方案：

According to the Newton’s universal law, the gravitational force (F) is inversely proportional to the square of the distance (d) between the two objects in contact. 

Mathematically:

 $F\propto\dfrac{1}{d^2}$

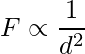
Consider the usual distance between the two bodies be x.

Then, the initial force is given as:

 $F_1\propto\dfrac{1}{x^2}$

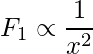
Now, when the distance between the two bodies changed to 6x then the new force is given as:

 $F_2 \propto \dfrac{1}{(6j)^2}\\\propto \dfrac{1}{36j^2}$

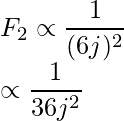
Now, divide the two forces as:

$\dfrac{F_1}{F_2}=\dfrac{1}{36}\\F_1=\dfrac{F_2}{36}$

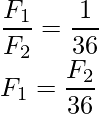
Hence, the usual force is changed by 1/36 times the new force.