欧几里得几何

几何词来自“ Geo”(意为地球)和“ meterin”(意为测量)。看起来几何形状源自测量土地的需求。几乎每个文明都对其进行了研究，例如埃及，中国，印度，希腊等。埃及人民能够计算出简单的面积和体积。他们甚至知道计算截顶金字塔体积的公式。我国还在我们的古代文明中详细研究了几何学。

印度的几何

在印度，印度河谷文明(约公元前3000年)广泛使用几何形状。这是一个高度组织的社会。这些城市的计划非常周到。例如，道路是平行的，并且存在地下排水系统。可以得出结论，城镇居民在测量方面确实很熟练。

在Sulbasutras中发现了相同的知识，其中有建筑手册。吠陀时期的几何形状起源于壁炉和祭坛的建造，用于表演吠陀仪式。对于家庭礼仪，正方形，圆形祭坛是优选的，并且具有三角形，长方形，梯形等形状的祭坛被用于公共礼拜。三角形的排列方式形成了43个子三角形。

欧几里得的几何定义

这些古老的文明主要将几何学用于实际目的，并且很少强调陈述背后的原因。欧几里得认为几何学是世界的某种抽象模型。点，线，形状的概念是从现实世界中看到的东西衍生出来的。他研究了现实世界中的物体，以将实体的概念形式化。

实体具有形状，大小，位置，并且可以从一个位置移动到另一个位置。它的边界称为曲面。它们将空间的一部分与另一部分隔开。假定它们没有厚度。曲面的边界由曲线或直线组成。这些线或曲线又由点组成。

从实线到直线，在每一步中，我们都会失去一个定义为尺寸的延伸。

欧几里得的定义：

1. 一点是没有任何意义的。
2. 一条线的宽度较小。
3. 线的末端是点。
4. 一条直线是一条与点本身均匀分布的线。
5. 表面是仅具有长度和宽度的表面。
6. 表面的边缘是线。
7. 平面是指在其表面上均匀分布有直线的表面。

在这些定义中，有些术语没有定义，或者可能需要进一步解释。例如一个点。

数学家决定让某些事情变得不确定。但是我们对这些术语的含义确实有直觉。因此，在几何图形中，我们将点，线和平面作为未定义的术语。

以这些定义为基础，欧几里得假定了一些尚待证明的性质。这些假设实际上是普遍真理。它们可以分为两种类型：

1. 假设：特定于几何的假设
2. 公理：常见概念

欧几里得公理

1. 等同于同一事物的事物彼此相等。
2. 如果将相等加到相等，则整体相等。
3. 如果从等于中减去等于，则余数等于。
4. 彼此重合的事物彼此相等。
5. 整体大于部分。
6. 相同事物的两倍的事物彼此相等。
7. 一半相同的事物彼此相等。

现在让我们讨论欧几里得的假设，

欧几里得的假设

假设1

A straight line may be drawn from any one point to any other point.

请注意，该假设表示至少有一条直线穿过两点，但是没有说明是否可以有多于一条这样的直线。但是在他的作品中，欧几里得经常认为它是独一无二的。

让我们将此结果写为公理

Axiom: Given two distinct points, there is a unique line that passes through them.

This statement is self-evident, let’s see it through a figure. 

假设2

A terminated line can be produced indefinitely.

Euclid terms a line segment as a terminated line. We know that a line segment can be extended in any of the directions. 

假设3

A circle can be drawn with any centre and any radius.

假设4

All right angles are equal to one another.

假设5

If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely, meet on that side on which the sum of angles is less than two right angles.

For example: 

We can see here, that the sum of interior angles is less than two right angles. Therefore, these two lines will intersect at a particular point if extended indefinitely. 

假设5比其他假设更复杂。但是不可能证明它们中的任何一个。

如今，“假定”和“轴心”在相同的意义上可以互换使用。当我们说“让我们假设”时，它的意思是“让我们根据宇宙中观察到的现象做出一些陈述”。之后，检查其真实性或有效性。如果它通过了该测试，则将其作为假设。

如果无法从这些公理中推导出与任何公理或先前证明的陈述相抵触的陈述，则该公理体系被称为“一致性”。因此，每当给出一个公理系统时，都必须检查一致性。

在假定和公理之后，欧几里得使用演绎推理来证明这些结果。被证明的陈述称为命题或定理。

定理：两条不同的线不能有一个以上的共同点。

Proof: Let us assume that there are two distinct lines “m” and “l”. We need to prove that they cannot intersect at more than two points. 

For now, consider that these two lines intersect at two points P and Q. So now we have two lines which passing through two distinct points, but it cannot be true as it violates the axiom we studied earlier. So it is not true and our assumption is false. 

Thus, two distinct lines cannot have more than one point in common. Hence, Proved. 

欧几里得几何上的样本问题

问题1：存在多少欧几里得假设?陈述他们每个人。

回答：

There are 5 postulates in Euclid’s Geometry,

1. A straight line may be drawn from any one point to any other point.
2. A terminated line can be produced indefinitely.
3. A circle can be drawn with any centre and any radius.
4. All right angles are equal to one another.
5. If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely, meet on that side on which the sum of angles is less than two right angles.

问题2：定义以下术语，

1. 平行线
2. 垂直线
3. 线段
4. 圆半径

回答：

1. Parallel Lines: The lines that maintain always equal distance from each other and hence they never meet.

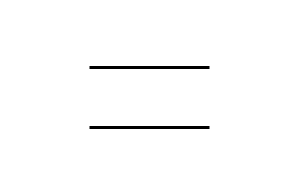
Lines: A line is a breadth less length.

Point: A point has no part

2. Perpendicular Lines: When two lines are perpendicular to each other (90° to each other), they are known as perpendicular lines.

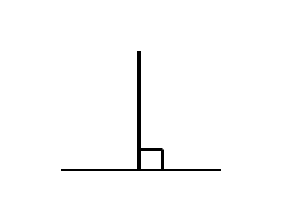
Angle: Two lines emerging from a same point form an angle.

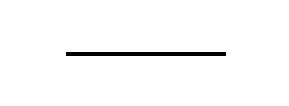
3. Line Segment: A Line having two ends and is finite in nature is a Line Segment.

4. Radius of a Circle: The Radius of a circle is defined as a line starting from the centre to the circumference.

问题3：在直线AB上，存在一个点C，使得AC = BC。证明线AC是线段AB的一半。

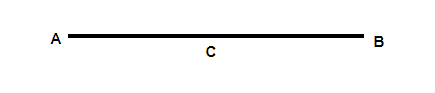
回答：

Given: AC= BC

To Prove: AC= 1/2 AB

Proof: 

We already know from the figure, AB=AC+ BC

But it is given that AC= BC

Therefore, we can write, AB= AC+ AC

AB= 2AC

AC= 1/2 AB

Hence, Proved.

问题4：在下图中，AD是线段，B和C是线上的两个点，使得AC = BD，证明AB = CD。

回答：

Given: AC = BD

To Prove: AB = CD

Proof: 

From the given figure, we can write, AC = AB + BC ⇢(1)

BD = BC + CD ⇢(2)

Equating equation (1) and (2) since, AC = BD (given)

AB + BC= BC + CD

AB = CD

Hence, Proved