9类RD Sharma解决方案–第8章直线和角度简介-练习8.2 |套装1

问题1：在下面的图中。OA和OB是相反的光线：

(i)如果x = 25°，y的值是多少?

(ii)如果y = 35°，x的值是多少?

解决方案：

(i) Given: 

x = 25

In the figure;

∠AOC and ∠BOC are forming a linear pair

So, ∠AOC + ∠BOC = 180°

In the figure;

 ∠AOC = 2y + 5 and ∠BOC = 3x

∠AOC + ∠BOC = 180°

(2y + 5) + 3x = 180

(2y + 5) + 3 (25) = 180

2y + 5 + 75 = 180

2y + 80 = 180

2y = 100

y = $\frac{100}{2}$ = 50

Hence, 

y = 50°

(ii) Given: 

y = 35°

In the figure; 

∠AOC + ∠BOC = 180° {Linear pair angles}

(2y + 5) + 3x = 180

(2(35) + 5) + 3x = 180

75 + 3x = 180

3x = 105

x = 35

Therefore, x = 35°

问题2.在下图中，写下所有相邻角对和所有线性对。

解决方案：

In the figure;

Pairs of adjacent angles are:

(∠AOC, ∠COB); 

(∠AOD, ∠BOD); 

(∠AOD, ∠COD); 

(∠BOC, ∠COD)

∠AOD + ∠BOD = 180° {Linear pair}

and 

∠AOC+ ∠BOC = 180° {Linear pair}

问题3.在给定的图中，找到x。进一步找到∠BOC，∠COD和，AOD。

解决方案：

In the figure; 

∠AOD and ∠BOD are forming linear pair,

Thus, 

∠AOD+ ∠BOD = 180°

And, 

∠AOD + ∠BOC + ∠COD = 180°

Given: 

∠AOD = (x+10)°, 

∠COD = x° 

and 

∠BOC = (x + 20)°

(x + 10) + x + (x + 20) = 180°

3x + 30 = 180°

3x = 180 – 30

x = $\frac{150}{3}$

x = 50°

Here,

∠AOD = (x+10) = 50 + 10 = 60

∠COD = x = 50°

∠BOC = (x+20) = 50 + 20 = 70°

Therefore, 

∠AOD = 60°, 

∠COD = 50° 

and 

∠BOC=70°

问题4。在图中，光线OA，OB，OC，OD和OE的公共端点为0。请证明∠AOB+∠BOC+∠COD+∠DOE+∠EOA= 360°。

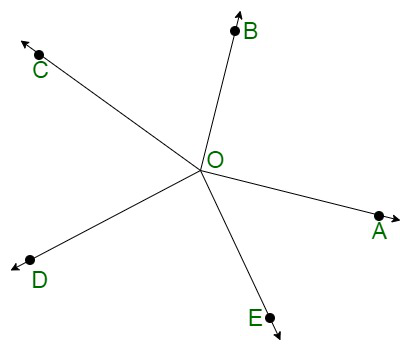
解决方案：

Given: 

Rays OA, OB, OC, OD and OE have the common endpoint O.

Construct: Draw an opposite ray OX to ray OA, which make a straight line AX.

In the figure:

∠AOB + ∠BOX = 180° {Linear pair}

Or, 

∠AOB + ∠BOC + ∠COX = 180° ……….(i)

Also,

∠AOE + ∠EOX  = 180°  {Linear pair}

Or, 

∠AOE + ∠DOE + ∠DOX = 180° ………..(ii)

After adding equations, (i) and (ii), we will get;

∠AOB + ∠BOC + ∠COX + ∠AOE + ∠DOE + ∠DOX = 180° + 180°

∠AOB + ∠BOC + ∠COD + ∠DOE + ∠EOA = 360°

Hence, Proved.

问题5.在图中，∠AOC和∠BOC形成线性对。如果a – 2b = 30°，找到a和b?

解决方案：

Given: 

∠AOC and ∠BOC are forming a linear pair.

 a + b = 180° …….(i)

a – 2b = 30° …….(ii) {given}

After subtracting equation (ii) from (i), we will get

a + b – a + 2b = 180 – 30

3b = 150

b = $\frac{150}{3}$

b = 50°

Thus, 

a – 2b = 30°

a – 2(50) = 30°

a = 30 + 100

a = 130°

Hence,

a = 130°

b = 50°

问题6.当两条线在一个点处相交时，会形成多少对相邻的角度?

解决方案：

Here, the four pairs of adjacent angles are formed when two lines intersect each other at a single point.

So here Let two lines AB and CD intersect at point O as shown below in the figure

Thus, the 4 pair of adjacent angles are :

(∠AOD, ∠DOB),

(∠DOB, ∠BOC),

(∠COA, ∠AOD) 

and 

(∠BOC, ∠COA)

问题7.在给定的图中，您总共可以命名几对相邻的角?

解决方案：

The number of Pairs of adjacent angles, from the following figure are;

∠EOC and ∠DOC

∠EOD and ∠DOB

∠DOC and ∠COB

∠EOD and ∠DOA

∠DOC and ∠COA

∠BOC and ∠BOA

∠BOA and ∠BOD

∠BOA and ∠BOE

∠EOC and ∠COA

∠EOC and ∠COB

Thus, we have 10 pairs of adjacent angles.

问题8.在图中，确定x的值。

解决方案：

As we know that, the sum of all the angles around a point O is equal to 360°.

Thus,

3x + 3x + 150 + x = 360°

7x = 360° – 150°

7x = 210°

x = $\frac{210}{7}$

x = 30°

Hence, the value of x is 30°.

问题9.在图中，AOC是一条线，找到x。

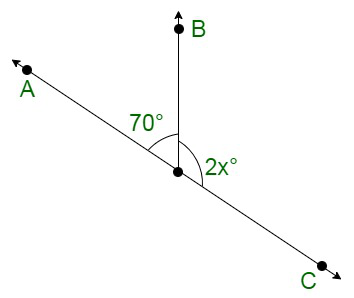
解决方案：

In the following figure, 

∠AOB + ∠BOC = 180° {Linear pairs}

70 + 2x = 180

2x = 180 – 70

2x = 110

x = $\frac{110}{2}$

x = 55°

Hence, the value of x is 55°.

问题10.在图中，POS是一条线，找到x。

解决方案：

In the following figure;

∠POQ + ∠QOS = 180° {Linear pair}

∠POQ + ∠QOR +∠SOR = 180°

60° + 4x + 40° = 180°

4x = 180° -100°

4x = 80°

x = 20°

Thus, the value of x is 20°.