您可能已经看过一组火车线,楼梯或一组钢琴键。这些有什么共同点吗?两条轨道永不交叉,梯子的两侧永不相交。钢琴琴键始终平行。让我们仔细看一下平行线和横向线,以及它们对应的角度。
Parallel lines: The combination of two or more lines that are stretched to infinity and never intersect each other are called the parallel line or coplanar lines. The parallel lines are denoted by a special symbol, given by ||.
Transversal: A transversal of any given line is a line that intersects two or more given lines at distinct points.
Corresponding angles: The angles made when a transversal intersect with any pair of parallel lines are called the corresponding angles.
The figure below shows both parallel and transversal lines along with the corresponding angles formed by them as:

平行线和横向
考虑一条线l,该线l在点P和Q处分别与线m和n相交。因此,线l是线m和n的横向线,其中获得了八个不同的角度。八个角度一起形成四对相应的角度。如下图所示,∠2和∠6构成一对相应的角度。相对于直线和横向线具有相同位置的所有角度都是一对相应的角度。
此外,将线之间的区域中的角度(例如,∠4和∠5)称为内角,而将位于两条线外侧的角度(例如,∠1和and8)称为外角。位于横向相对侧的角度称为交替角度,例如∠4和∠6。共享相同顶点并具有共同光线的角度(例如,图中的∠1和∠2或∠6和∠5)称为相邻角。在这种情况下,获得了由两条直线相交的相邻角,两条直线相交是互补的。彼此相对的两个角度分别为∠1和∠3,称为垂直角。

平行线和横向线所成的角度
Hence, the pair of corresponding angles, alternate interior angles, alternate exterior angles, Interior angles on the same side of the transversal are as follows:
- Corresponding angles: ∠ 1 and ∠ 5, ∠ 2 and ∠ 6, ∠ 4 and ∠ 8 and ∠ 3 and ∠ 7.
- Alternate interior angles: ∠ 4 and ∠ 6, and ∠ 3 and ∠ 5.
- Alternate exterior angles: ∠ 1 and ∠ 7, and ∠ 2 and ∠ 8.
- Interior angles on the same side of the transversal: ∠ 4 and ∠ 5, and ∠ 3 and ∠ 6.
对应角度公理
让我们找出当线m平行于线n时,这些对中的角度之间的关系。

对对应的角度。
因此,相应的角度公理表示为:
If a transversal intersects two parallel lines, such that a pair of corresponding angles is equal, then the two lines are parallel to each other.
证明:相应的角度相等。
证明:线m和n彼此平行,线l遍历。
由于线m和n是平行的。
所以,
∠3 +∠6 = 180°(平行四边形的相邻角)……(1)
∠7 +∠6 = 180°(附加角)……(2)
∠3 +∠2 = 180°(附加角)……(3)
因此,从等式(1)和(2)可以得出结论:
∠3 =∠7
同样,从(1)和(3)可以得出结论:
∠6 =∠2
这样,也可以证明:
∠1 =∠5
∠4 =∠8
这意味着,所有四对对应的角度都彼此相等。
对应角公理的逆
对应的角度公理的相反表示为:
If a transversal intersects two lines such that a pair of corresponding angles is equal, then the two lines are parallel to each other.

对对应的角度。
证明:如果相应的角度相等,则线是平行的。
证明:线l遍历线m和n。
所以,
∠3 =∠7(给定,相应的角度相等)……(1)
∠7 +∠6 = 180°(附加角)……(2)
因此,从等式(1)和(2)可以得出结论:
∠3 +∠6 = 180°
因此,相邻角度之和是补充。
因此,线是平行的。
交替角度公理
备用角度公理表示为:
If a transversal intersects two parallel lines, then each pair of alternate interior angles is equal.

一对交替的角度。
证明:交替的内角相等。
证明:线m和n彼此平行,线l是遍历的。
∠3 =∠7(对应的公理角)……(1)
∠7 =∠5(垂直相反的角度)……(2)
因此,从等式(1)和(2)可以得出结论:
∠3 =∠5
同样,它可以写为:
∠4 =∠6
因此,交替的内角是相等的。
交替角度公理的逆
备用角度公理的反函数表示为:
If a transversal intersects two lines such that a pair of alternate interior angles is equal, then the two lines are parallel.

一对交替的角度。
证明:如果交替的内角相等,则两条线平行。
证明:线m和n彼此平行,线l遍历。
所以,
∠3 =∠5(交替的内角)…….(1)
∠7 =∠5(垂直相反的角度)……。(2)
因此,从等式(1)和(2)可以得出结论:
∠3 =∠7
根据对应角度公理的反面:如果一个横向线与两条线相交,使得一对对应的角度相等,则两条线彼此平行。
因此,两条线是平行的。
横向同一侧的内角特性
内角的属性表示为:
If a transversal intersects two parallel lines, then each pair of interior angles on the same side of the transversal is supplementary.

对内角
证明:如果一个横向线与两条平行线相交,则该横向线同一侧的每对内角都是互补的。
证明:线m和n彼此平行,线l遍历。
所以,
∠3 =∠7(对应的公理角)……(1)
∠6 +∠7 = 180°(附加角)……(2)
因此,从等式(1)和(2)可以得出结论:
∠6 +∠3 = 180°
因此,横向同一侧的每对内角都是互补的。
横截面同一侧的内角特性的逆
内角特性的反面表示为:
If a transversal intersects two lines such that a pair of interior angles on the same side of the transversal is supplementary, then the two lines are parallel.

对内角。
证明:如果一个横向线与两条线相交,使得该横向线同一侧上的一对内角是互补的,则两条线是平行的。
证明:横向同一侧的一对内角是补充的。
∠6 +∠3 = 180°(给定,一对内角的和为补充)……(1)
∠6 +∠7 = 180°(附加角)……(2)
因此,从等式(1)和(2)可以得出结论:
∠7 =∠3
因此,两条线是平行的。
平行于同一条线的线
平行于同一条线的线定理表示为:
Lines which are parallel to the same line are parallel to each other.

与同一条线平行的线。
证明: 平行于同一条线的线彼此平行(p || q || r)。
证明:线p和r平行,线p和q彼此平行,线l遍历。
现在,p || q和p || [R
由于m ||因此,
∠1 =∠2(对应的公理角)……(1)
∠1 =∠3(对应的公理角)……(2)
因此,从等式(1)和(2)可以得出结论:
∠2 =∠3
然而,根据对应角度公理的相反,如果一个横向线与两条线相交,使得一对对应的角度相等,则两条线彼此平行。
因此,两条线q和r平行,因此平行于r。
样本问题
问题1:在图中,如果PQ || RS,∠MXQ = 135°,∠MYR = 40°,找到∠XMY。
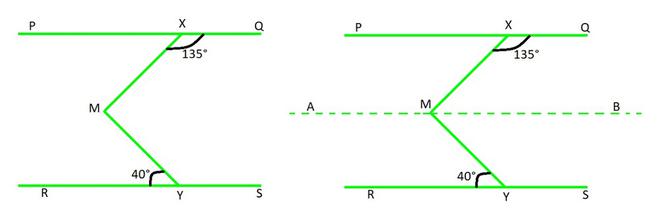
解决方案:
Lets construct a line AB parallel to line PQ, through point M.
Now, AB || PQ and PQ || RS
⇒ AB || RS || PQ (Theorem 5)
∠ QXM + ∠ XMB = 180° (AB || PQ, Interior angles on the same side of the transversal XM)
As, ∠ QXM = 135°
135° + ∠ XMB = 180°
∠ XMB = 45°
Now, ∠ BMY = ∠ MYR (AB || RS, Alternate angles)
∠ BMY = 40°
As, ∠ XMB + ∠ BMY = 45° + 40°
Therefore, ∠ XMY = 85°.
问题2:如果一个横向线与两条线相交,使得一对相应角度的等分线平行,则证明两条线是平行的。
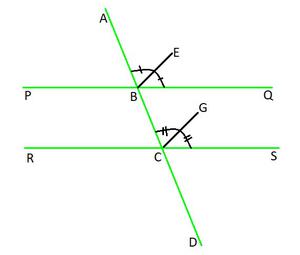
解决方案:
Let a transversal AD intersects two lines PQ and RS at points B and C respectively. Ray BE is the bisector of ∠ ABQ and ray CG is the bisector of ∠ BCS and BE || CG.
To prove: PQ || RS.
Given that, the ray BE is the bisector of ∠ ABQ.
Therefore, ∠ ABE = ½ ∠ ABQ ……(1)
Similarly, ray CG is the bisector of ∠ BCS.
Therefore, ∠ BCG = ½ ∠ BCS ……(2)
Using Corresponding angles axiom,
∠ ABE = ∠ BCG (BE || CG and AD is the transversal) ……(3)
Using (1) and (2) in (3), you get
½ ∠ ABQ = ½ ∠ BCS
That is, ∠ ABQ = ∠ BCS
As they are the corresponding angles formed by transversal AD with PQ and RS.
Therefore, PQ || RS. (Converse of corresponding angles axiom)
问题3:在图中,AB || CD和CD ||英孚也是EA⊥AB。如果∠BEF = 55°,则找到x,y和z的值。

解决方案:
Since, AB || CD and CD || EF
⇒ AB || CD || EF (Lines which are parallel to the same line are parallel to each other)
And, EB and AE are transversal.
y + 55° = 180° (CD || EF, Interior angles on the same side of the transversal EB)
y = 180º – 55º = 125º
As, x = y (AB || CD, Corresponding angles axiom)
x = y = 125º
Now, ∠ EAB + ∠ FEA = 180° (Interior angles on the same side of the transversal EA)
90° + z + 55° = 180°
Hence, z = 35°.
问题4:在图中,找到x和y的值,然后证明AB ||光盘。

解决方案:
Here,
x+50° = 180° (linear pair is equal to 180°)
x = 130°
and, y = 130° (vertically opposite angles are equal)
Here, what we can observe is,
x = y = 130°
In two parallel lines, the alternate interior angles are equal.
Hence, this proves that alternate interior angles are equal and so, AB || CD.
问题5:在图中,如果AB || CD,∠APQ = 50°,∠PRD = 127°,求x和y。

解决方案:
Here, APQ = PQR (Alternate interior angles)
x = 50°
And,
APR = PRD (Alternate interior angles)
APQ+QPR = 127°
127° = 50°+ y
y = 77°
Hence, the values are, x = 50° and y = 77°.