问题1.在两个直角三角形中,一侧的锐角等于另一侧的锐角。证明三角形是全等的。
解决方案:
We have two right triangles ABC and DEF in which one side and acute angle of one are equal to the corresponding side and angles of the other, i.e.,
∠A = ∠D
BC = EF

Prove: Δ ABC ≅ Δ DEF
Proof:
From Δ ABC and Δ DEF
∠ B = ∠ E = 90° …….(i)[Right triangle]
BC = EF …….(ii)[Given]
∠A = ∠D ……(iii)[Given]
By AAS congruence criterion,
Δ ABC ≅ Δ DEF
Hence, proved.
问题2.三角形的外部垂直角的平分线是否平行于底角。证明三角形是等腰的。
解决方案:
In ABC be a triangle
Given that AD is bisector of∠EAC and AD || BC.

From the above figure,
∠1 = ∠2 [AD is a bisector of ∠ EAC]
∠1 = ∠3 [Corresponding angles]
and
∠2 = ∠4 [alternative angle]
Also, we have ∠3 = ∠4
AB = AC
So the sides AB and AC are equal so
Δ ABC is an isosceles triangle.
问题3.在等腰三角形中,如果顶点角度是底角之和的两倍,则计算三角形的角度。
解决方案:
Let us considered Δ ABC is isosceles triangle
In which AB = AC and ∠B = ∠C
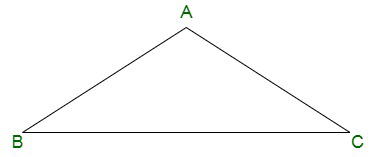
Given: ∠A = 2(∠B + ∠C)
Find: The value of ∠A, ∠B, and ∠C
So, we have
∠A = 2(∠B + ∠C)
∠A = 2(∠B + ∠B) [∠B = ∠C because Δ ABC is isosceles triangle]
∠A = 2(2 ∠B)
∠A = 4(∠B)
As we know that sum of angles in an isosceles triangle = 180°
So, ∠A + ∠B + ∠C = 180°
Now put the value of ∠A and ∠C, we get
4 ∠B + ∠B + ∠B = 180°
6 ∠B =180°
∠B = 30°
Here, ∠B = ∠C
∠B = ∠C = 30°
And ∠A = 4 ∠B
∠A = 4 x 30° = 120°
Hence, the value of ∠A = 120°, ∠B = 30°, and ∠C = 30°.
问题4. PQR是一个三角形,其中PQ = PR,并且是PQ边上的任意点。通过S,画一条平行于QR的直线,并在T处与PR相交。证明PS = PT。
解决方案:
In ΔPQR is a triangle
It is given that PQ = PR, and S, a line is drawn parallel to QR and intersecting PR at T
So, ST || QR.
Prove: PS = PT

As we know that PQ = PR, so the given △PQR is an isosceles triangle.
Hence, ∠ PQR = ∠ PRQ
∠ PST = ∠ PQR and ∠ PTS = ∠ PRQ [Corresponding angles as ST parallel to QR]
∠ PQR = ∠ PRQ
∠ PST = ∠ PTS
So, In Δ PST,
∠ PST = ∠ PTS
Therefore, Δ PST is an isosceles triangle.
So, PS = PT.
Hence, proved.
问题5:在一个△ABC中,假定AB = AC,而B和C的等分线在O处相交。如果M是产生的BO上的一个点,则证明∠MOC=∠ABC。
解决方案:
In △ABC,
It is given that AB = AC and the bisector of ∠B and ∠C intersect at O.

Prove: ∠MOC = ∠ABC
It is given that AB = AC
So the △ABC is an isosceles triagnle
Hence
∠B = ∠C
∠ABC = ∠ACB
From the figure BO and CO are bisectors of ∠ABC and ∠ACB
So,
∠ABO = ∠OBC = ∠ACO = ∠OCB = 1/2 ∠ABC = 1/2 ∠ACB ………..(i)
In △OBC
∠BOC + ∠MOC = 180° ………(ii) [Straight line]
∠OBC + ∠OCB + ∠BOC = 180°[Sum of angles in an isosceles triangle = 180°]
From equation (ii)
∠OBC + ∠OCB + ∠BOC = ∠BOC + ∠MOC
∠OBC + ∠OCB = ∠MOC
2∠OBC = ∠MOC
From Equation(i)
2(1/2 ∠ABC) = ∠MOC
∠ABC = ∠MOC
Hence proved
问题6. P是ABC角平分线上的一个点。如果平行于AB的P线与Q的BC相遇,则证明三角形BPQ为等腰。
解决方案:
Given that P is a point on the bisector of ∠ABC, and PQ || AB.

Prove: △BPQ is an isosceles triangle
It is given that BP is bisector of ∠ABC
So, ∠ABP = ∠PBC ……….(i)
Also given that PQ || AB
So, ∠BPQ = ∠ABP ……….(ii) [alternative angles]
From equation (i) and (ii), we have
∠BPQ = ∠PBC
or ∠BPQ = ∠PBQ
In △BPQ
∠BPQ = ∠PBQ
Hence, proved △BPQ is an isosceles triangle.
问题7.证明等边三角形的每个角度均为60°。
解决方案:
Prove: Each angle of an equilateral triangle is 60°.
Let us considered we have an equilateral triangle △ABC
So, AB = BC = CA
Take AB = BC
So, ∠A = ∠C …….(i) [Because opposite angles to equal sides are equal]
Take BC = AC
∠B = ∠A ………(ii) [Because opposite angles to equal sides are equal]
From (i) and (ii), we get
∠A = ∠B = ∠C ………….(iii)
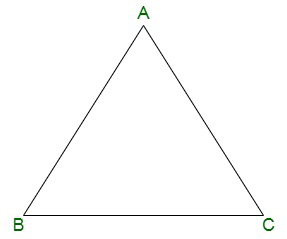
As we already know that the sum of angles in a triangle = 180°
So, ∠A + ∠B + ∠C = 180°
From equation(iii), we get
∠A + ∠A + ∠A = 180°
3∠A = 180°
∠A = 60°
So, ∠A = ∠B = ∠C = 60°
Hence Proved
问题8.三角形ABC的角度∠A,∠B,∠C彼此相等。证明ABC是平等的。
解决方案:
Given that in △ABC
∠A =∠B = ∠C
Prove: △ABC is equilateral

In △ABC
As we already know that the sum of angles in a triangle = 180°
So, ∠A + ∠B + ∠C = 180°
Given that ∠A =∠B = ∠C
So,
∠A + ∠A + ∠A = 180°
3∠A = 180°
∠A = 60°
So, ∠A =∠B = ∠C = 60°
As we know that the angles of equilateral triangles are of 60°
Hence, proved that △ABC is equilateral.
问题9. ABC是一个三角形,其中∠B= 2∠C。 D是BC上的一点,因此AD将∠BAC一分为二,而AB = CD。证明∠BAC= 72°。
解决方案:
Given that in △ABC,
∠B = 2 ∠C, AD bisectors of∠BAC, and AB = CD.

Prove:∠BAC = 72°
Now, draw a line BP which is bisector of ∠ABC, and join PD.
Let us considered∠C = ∠ACB = y
∠B = ∠ABC = 2y
Let us considered ∠BAD = ∠DAC = x
∠BAC = 2x [AD is the bisector of ∠BAC]
In △BPC,
∠CBP = ∠PCB = y [BP is the bisector of ∠ABC]
PC = BP
In △ABP and △DCP,
∠ABP = ∠DCP = y
AB = DC [Given]
And PC = BP
So, by SAS congruence criterion,
△ABP ≅ △DCP
∠BAP = ∠CDP and AP = DP [C.P.C.T]
∠CDP = 2x
∠ADP = ∠DAP = x
In △ABD
∠ABD + ∠BAD + ∠ADB = 180°
∠ADB + ∠ADC = 180°
So,
∠ABD + ∠BAD + ∠ADB = ∠ADB + ∠ADC
2y + x = ∠ADP + ∠PDC
2y + x = x + 2x
2y = 2x
y = x
In △ABC,
As we already know that the sum of angles in a triangle = 180°
∠A + ∠B + ∠C = 180°
2x + 2y + y = 180° [∠A = 2x, ∠B = 2y, ∠C = y]
2(y) + 3y = 180° [x = y]
5y = 180°
y = 36°
∠A = ∠BAC = 2 × 36 = 72°
∠A = 72°
Hence proved
问题10. ABC是一个直角三角形,其中∠A= 90°,AB = AC。找到∠B和∠C。
解决方案:
Given that in ABC is a right-angled triangle
∠A = 90° and AB = AC
Find: ∠B and ∠C
In △ABC
AB = AC [Given]
∠B = ∠C
As we already know that the sum of angles in a triangle = 180°
So, ∠A + ∠B + ∠C = 180°
90° + ∠B + ∠B = 180°
2 ∠B = 180° – 90°
∠B = 45°
Hence, the value of ∠B = ∠C = 45°.