在本文中,我们将讨论以下概念,
- 什么是 Z 分数?
- Z-Score 公式
- 如何计算 Z 分数?
- Z-score的解释
什么是 Z 分数?
Z-score 也称为标准分数,让我们了解数据点与平均值之间的距离。它表示一个元素与平均值相差多少标准差。因此,Z-Score 是根据与平均值的标准偏差来衡量的。例如,标准差为 2 表示该值与平均值相差 2 个标准差。为了使用 z 分数,我们需要知道总体均值 (μ) 以及总体标准差 (σ)。
Z-Score 的公式
A z-score can be calculated using the following formula.
z = (X – μ) / σ
where,
z = Z-Score,
X = The value of the element,
μ = The population mean, and
σ = The population standard deviation
如何计算 Z 分数?
通常,问题陈述中提供了总体均值 ((μ)、总体标准差 (σ) 和观测值 (x),将其代入上述 Z-score 方程中即可得出 Z-Score 值. 根据给定的 Z-Score 是正值还是负值,可以使用在线或附录中统计教科书背面的相应正 Z 表或负 Z 表。
例子:
Question:
You take the GATE examination and score 500. The mean score for the GATE is 390 and the standard deviation is 45. How well did you score on the test compared to the average test taker?
Solution:
The following data is readily available in the above question statement
Raw score/observed value = X = 500
Mean score = μ = 390
Standard deviation = σ = 45
By applying the formula of z-score,
z = (X – μ) / σ
z = (500 – 390) / 45
z = 110 / 45 = 2.44
This means that your z-score is 2.44.
Since the Z-Score is positive 2.44, we will make use of the positive Z-Table.
Now let’s take a look at Z Table (CC-BY) to know how well you scored compared to the other test-takers.
Follow the instruction below to find the probability from the table.
Here, z-score = 2.44
- Firstly, map the first two digits 2.4 on the Y-axis.
- Then along the X-axis, map 0.04
- Join both axes. The intersection of the two will provide you the Z-Score value you’re looking for
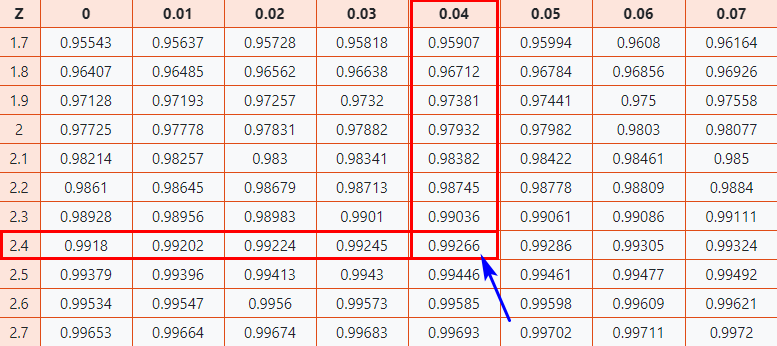
As a result, you will get the final value which is 0.99266.
Now, we need to compare how our original score of 500 on the GATE examination compares to the average score of the batch. To do that we need to convert the Z-Score into a percentage value.
0.99266 * 100 = 99.266%
Finally, you can say that you have performed well than almost 99% of other test-takers.
Z-score的解释
- z 分数小于 0 的元素表示该元素小于平均值。
- z 分数大于 0 的元素表示该元素大于平均值。
- z-score 等于 0 的元素表示该元素等于平均值。
- z-score 等于 1 的元素表示该元素比均值大 1 个标准差; z 分数等于 2,比平均值大 2 个标准差,依此类推。
- z-score 等于 -1 的元素表示该元素比均值小 1 个标准差; z 分数等于 -2,比平均值小 2 个标准差,依此类推。
- 如果给定集合中的元素数量很大,那么大约 68% 的元素的 z-score 介于 -1 和 1 之间;大约 95% 的人的 z 分数在 -2 到 2 之间;大约 99% 的人的 z-score 介于 -3 和 3 之间。这被称为经验法则或 68-95-99.7 法则,可以在下图中展示
