第 11 类 RD Sharma 解决方案 - 第 26 章椭圆 - 练习 26.1 |设置 1

问题 1. 求焦点为 (1,–2)、准线为 3x – 2y + 5 = 0、偏心率为 1/2 的椭圆的方程。

解决方案：

Given that, 

Focus is (1, -2)

directrix is 3x – 2y + 5 = 0, 

eccentricity(e) is 1/2.

As we know, SP = ePM

⇒ SP = PM/2

⇒ (SP)² = 1/4(PM)² 

⇒ 4(SP)² = (PM)²

⇒ 4[(x – 1)² + (y + 2)²] = $[\frac{3x-2y+5}{\sqrt{3^2+2^2}}]^2$

⇒ 4[x² + 1 – 2x + y² + 4 + 4y] = $\frac{(3x-2y+5)^2}{13}$

⇒ 52[x² + 1 – 2x + y² + 4 + 4y] = (3x – 2y + 5)²

⇒ 52[x² + 1 – 2x + y² + 4 + 4y] = 9x² + 4y² + 25 – 12xy – 20y + 30x 

Thus 43x² + 43y² + 12xy – 134x + 228y + 235 = 0 is the required equation.

问题 2. 如果满足以下条件，则求椭圆方程：

(i) 焦点是 (0, 1)，准线是 x + y = 0 和 e = 1/2。

解决方案：

Given that,

focus is (0, 1), 

directrix is x + y = 0 

eccentricity(e) is 1/2

As we know, SP = ePM

⇒ SP = PM/2

⇒ (SP)² = 1/4(PM)²

⇒ 4(SP)² = (PM)²

⇒ 4[(x – 0)² + (y – 1)²] = $[\frac{x+y}{\sqrt{1^2+1^2}}]^2$

⇒ 8[x² + y² – 2y + 1] = x² + y² + 2xy

Thus 7x² + 7y² – 2xy – 16y + 8 = 0 is the required equation.

(ii) 焦点是 (–1,1)，准线是 x – y + 3 = 0 和 e = 1/2。

解决方案：

Given that,

focus is (-1, 1), 

directrix is x – y + 3 = 0 

eccentricity(e) is 1/2

As we know, SP = ePM

⇒ SP = PM/2

⇒ (SP)² = 1/4(PM)²

⇒ 4(SP)² = (PM)²

⇒ 4[(x + 1)² + (y – 1)²] = $[\frac{x-y+3}{\sqrt{1^2+1^2}}]^2$

⇒ 4[(x + 1)² + (y – 1)²] = (x – y + 3)²/2 

⇒ 8[(x + 1)² + (y – 1)²] = (x – y + 3)²

⇒ 8[(x + 1)² + (y – 1)²] = x² + y² + 9 – 6y – 2xy + 6x

⇒ 8[(x² + 1 + 2x) + (y² + 1 – 2y)] = x² + y² + 9 – 6y – 2xy + 6x

⇒ 8[x² + y² +  2 + 2x – 2y] = x² + y² + 9 – 6y – 2xy + 6x

Thus 7x² + 7y² + 2xy + 10x – 10y + 7 = 0 is the required equation.

(iii) 焦点是 (–2,3)，准线是 2x + 3y + 4 = 0 和 e = 4/5。

解决方案：

Given that,

focus is (-2, 3), 

directrix is 2x + 3y + 4 = 0 

eccentricity(e) is 4/5

As we know, SP = ePM

⇒ SP = 4/5(PM) 

⇒ (SP)² = 16/25(PM)² 

⇒ 25(SP)² = 16(PM)²

⇒ 25[(x + 2)² + (y – 3)²] = $16[\frac{2x+3y+4}{\sqrt{2^2+3^2}}]^2$

⇒ 25[(x + 2)² + (y – 3)²] = 16(2x + 3y + 4)²/13 

Thus 325[x² + y² + 4x – 6y + 13] = 16(2x + 3y + 4)² is the required equation.

(iv) 焦点是 (1, 2)，准线是 3x + 4y – 5 = 0 和 e = 1/2。

解决方案：

Given that,

focus is (1, 2), 

directrix is 3x + 4y – 5 = 0

eccentricity(e) is 1/2

We know, SP = ePM

⇒ SP = PM/2

⇒ (SP)² = 1/4(PM)²

⇒ 4(SP)² = (PM)²

⇒ 4[(x – 1)² + (y – 2)²] = $[\frac{3x+4y-5}{\sqrt{3^2+4^2}}]^2$

⇒ 4[(x – 1)² + (y – 2)²] = (3x + 4y – 5)²/25 

⇒ 100[(x – 1)² + (y – 2)²] = 9x² + 16y² + 25 + 24xy – 40y – 30x

⇒ 100[(x² + 1 – 2x) + (y² + 4 – 4y)] = 9x² + 16y² + 25 + 24xy – 40y – 30x

Thus 91x² + 84y² – 24xy – 170x – 360y + 475 = 0 is the required equation.

问题 3. 求椭圆的偏心率、焦点坐标、纬直角长度：

(i) 4x ² + 9y ² = 1

解决方案：

Given that 4x² + 9y² = 1

$\frac{x^2}{\frac{1}{4}}+\frac{y^2}{\frac{1}{9}}=1$

So, 

⇒ Eccentricity = $\sqrt{\frac{\frac{1}{4}-\frac{1}{9}}{\frac{1}{4}}}$

= √5/3         

Length of latus rectum = $\frac{\frac{2}{9}}{\frac{1}{2}}$

= 4/9

Foci are(√5/6; 0) and (-√5/6; 0)  

(ii) 5x ² + 4y ² = 1

解决方案：

Given that 5x² + 4y² = 1

$\frac{x^2}{\frac{1}{5}}+\frac{y^2}{\frac{1}{4}}=1$

So, 

⇒ Eccentricity = 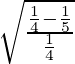$\sqrt{\frac{\frac{1}{4}-\frac{1}{5}}{\frac{1}{4}}}$

= 1/√5 

Length of latus rectum = $\frac{\frac{2}{5}}{\frac{1}{2}}$

= 4/5

Foci are (0; 1/2√5) and (0; -1/2√5) 

(iii) 4x ² + 3y ² = 1

解决方案：

Given that 4x² + 3y² = 1

$\frac{x^2}{\frac{1}{4}}+\frac{y^2}{\frac{1}{3}}=1$

So, 

⇒ Eccentricity = $\sqrt{1-\frac{\frac{1}{4}}{\frac{1}{3}}}$

= 1/2

Length of latus rectum = 2a²/b

= $\frac{\frac{2}{4}}{\frac{1}{\sqrt3}}$

= √3/2 

Foci are (0; 1/2√3)  and (0; -1/2√3).        

(iv) 25x ² + 16y ² = 1600

解决方案：

Given that 25x² + 16y² = 1600

$\frac{25x^2}{1600}+\frac{16y^2}{1600}=1$

⇒ $\frac{25x^2}{1600}+\frac{16y^2}{1600}=1$

So, 

⇒ Eccentricity = $\sqrt{1-\frac{a^2}{b^2}}$

= 3/5

⇒ Coordinates of foci are (0, 6) and (0, –6).

⇒ Length of latus rectum = 2a²/b

= 2 x (64/10)

= 64/5

问题 4. 求过点 (–3, 1) 且有偏心率的椭圆方程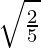$\sqrt{\frac{2}{5}}$ .    

解决方案：

Let the equation of the plane be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  …(i)

It is given that the ellipse pass through the point (–3, 1), so,

$\frac{9}{a^2}+\frac{1}{b^2}=1$ …(ii)

⇒ $e=\sqrt{1-\frac{a^2}{b^2}}$

⇒ $\sqrt{\frac{2}{5}}=\sqrt{1-\frac{a^2}{b^2}}$

⇒ b²/a² = 3/5 

⇒ b² = 3a²/5

⇒ b² = 3a²/5 ……(iii)

Now put the value of b² in equation (ii), we get

$\frac{9}{a^2}+\frac{1}{\frac{3a^2}{5}}=1$

$\frac{9}{a^2}+\frac{5}{3a^2}=1$

$\frac{1}{a^2}[9 + \frac{5}{3}]=1$

9 + 5/3 = a²

a² = 32/3

Now put thew value of a² in eq(iii), we get,

b² = 3/5 x 32/3 = 32/5

Now put the a² and b² in eq(i), we get,

$\frac{x^2}{\frac{32}{3}}+\frac{y^2}{\frac{32}{5}}=1$

Thus 3x² + 5y² = 32 is the required equation of the plane.

问题 5. 求椭圆方程，如果：

(i) e = 1/2 和焦点 (±2, 0)。

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  …(i)

Now, ae = 2

or, a² = 16

Now, b² = a²(1 – e²)

⇒ b² = 16(1 – (1/2)²)

⇒ b² = 12

Now put the a² and b² in eq(i), we get,

$\frac{x^2}{16}+\frac{y^2}{12}=1$

Thus 3x² + 4y² = 48 is the equation of the ellipse.

(ii) e = 2/3，阔直肠长度 = 5

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  …(i)

Now, 

⇒ 2b²/a = 5

⇒ b² = 5a/2  ….(ii)

Since, b² = a²(1 – e²)

⇒ 5a/2  = a²(1 – (2/3)²)

⇒ a = 9/2

⇒ a² = 81/4

Now put the value of a in eq(ii), we get

b² = 5/2 x 9/2

b² = 45/4

Now put the a² and b² in eq(i), we get,

$\frac{x^2}{\frac{81}{4}}+\frac{y^2}{\frac{45}{4}}=1$

Thus 20x² + 36y² = 405 is the required equation.

(iii) e = 1/2 和半长轴 = 4

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Semi – major axis = a = 4

⇒ a² = 16

We know, a² = b²(1 – e²)

⇒ 16 = b²(1 – (1²/2²)) 

⇒ b² = 12

Now put the a² and b² in eq(i), we get,

⇒ $\frac{x^2}{16}+\frac{y^2}{12}=1$

Thus 3x² + 4y² = 48 is the required equation.

(iv) e = 1/2 和长轴 = 12

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Given, 2a = 12

⇒ a = 6

We know, $b=\sqrt{1-\frac{b^2}{a^2}}$

⇒ $\frac{1}{2}=\sqrt{1-\frac{b^2}{36}}$

⇒ b² = 27

On substituting the values of a² and b² in eq(i), we get,

⇒ $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$

⇒ $\frac{x^2}{36}+\frac{y^2}{27}=1$

Thus 3x² + 4y² = 108 is the equation of the ellipse.

(v) 它通过 (1, 4) 和 (–6, 1)。

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

It is given that ellipse passes through (1, 4) and (–6, 1), we get

$\frac{1}{a^2}+\frac{16}{b^2}=1$

 Let p = 1/a² and r = 1/b² 

⇒ p + 16r = 1       ……(ii)

 Since the ellipse also passes through the point (–6, 1), we have

$\frac{36}{a^2}+\frac{1}{b^2}=1$

⇒ 36p + r = 1      ……(iii)

On solving eq(ii) and (iii), we have:

p = 3/115; r = 7/115

On substituting the values in eq(i), we get;

⇒ $\frac{3x^2}{115}+\frac{7y^2}{115}=1$

Thus 3x² + 7y² = 115 is the required equation.

(vi) 其顶点为 (±5, 0) 和 foci(±4, 0)

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Given that a = 5 and ae = 4

Thus, e = 4/5 $e=\frac{4}{5}$

Now, b² = a²(1 – e²)

⇒ b² = 25(1 – 16/25) 

⇒ b² = 9

On substituting the values of a² and b² in eq(i), we get,

Thus $\frac{x^2}{25}+\frac{y^2}{9}=1$ is the required equation.

(vii) 顶点为 (0, ±13) 和焦点 (0, ±5)

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Given: b = 13 and be = 5

Hence, e = 5/13 

Now, a² = b²(1 – e²)

⇒ a² = 169(1 – 25/169)

⇒ a² = 144

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{144}+\frac{y^2}{169}=1$

Thus $\frac{x^2}{144}+\frac{y^2}{169}=1$ is the required equation.

(viii) 它的顶点是 (±6, 0) 和 foci(±4, 0)

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Given: a = 6 and ae = 4

Thus, e = 2/3 

Now, b² = a²(1 – e²)

⇒ b² = 36(1 – 16/36)

⇒ b² = 20

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{36}+\frac{y^2}{20}=1$

Thus $\frac{x^2}{36}+\frac{y^2}{20}=1$ is the required equation.

(ix) 长轴末端 (±3, 0) 和短轴末端 (0, ±2)。

解决方案：

Let the equation of the ellipse be $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$…..(i)

Ends of major axis = (±3, 0)

Ends of minor axis = (0, ±2)

Since the ends of the major and minor axes are (±a, 0) and (0, ±b) respectively.

Hence, a = 3 and b = 2

So, a² = 9, b² = 4

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{9}+\frac{y^2}{4}=1$

Thus, $\frac{x^2}{9}+\frac{y^2}{4}=1$is the required equation.

(x) 长轴末端 (0, ±√5) 和短轴末端 (±1, 0)。

解决方案：

Let the equation of the ellipse be $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ ….(i)

Ends of major axis = (0, ±√5)

Ends of minor axis = (±1, 0)

Since the ends of the major and minor axes are (±a, 0) and (0, ±b) respectively.

Hence, a = 1 and b = √5  

So, a² = 1, b² = 5

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{1}+\frac{y^2}{5}=1$

Thus, $\frac{x^2}{1}+\frac{y^2}{5}=1$is the required equation.

(xi) 长轴的长度为 26 和焦点 (±5, 0)。

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Given that the length of major axis = 26 and foci (±5, 0).

We have, 2a = 26

⇒ a = 13

⇒ a² = 169

 Also, ae = 5

⇒ e = 5/13 

We know, $e=\sqrt{1-\frac{b^2}{a^2}}$

⇒ $\frac{5}{13}=\sqrt{1-\frac{b^2}{169}}$

⇒ b² = 144

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{169}+\frac{y^2}{144}=1$

Thus $\frac{x^2}{169}+\frac{y^2}{144}=1$ is the required equation.

(xii) 短轴长度为 16 且 foci(0, ±6)

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Given that, length of minor axis is 16

2a = 16

a = 8

a² = 64

Now the coordinates of foci are (0, ±be)

So, be = 6 

(be)² = 36 

We know, a² = b²(1 – e²)

a² = b² – b²e²

64 = b² – 36 

b² = 100 

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{64}+\frac{y^2}{100}=1$

Thus, $\frac{x^2}{64}+\frac{y^2}{100}=1$ is the required equation.

(xiii) 焦点为 (±3, 0) 且 a = 4

解决方案：

Let the equation of the ellipse be:

$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Given that, ae = 3 and a = 4

Thus, e = 3/4 and a² = 16

We know, $e=\sqrt{1-\frac{b^2}{a^2}}$

⇒ $\frac{3}{4}=\sqrt{1-\frac{b^2}{16}}$

⇒ b² = 7

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{16}+\frac{y^2}{7}=1$

Thus $\frac{x^2}{16}+\frac{y^2}{7}=1$ is the required equation of the ellipse.

问题 6. 求出焦点为 (±4, 0), e = 1/3 的椭圆的方程。

解决方案：

Let the equation of the ellipse be $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

Then the coordinates of the foci are (±a, 0).

We have, ae = 4 and  e = 1/3

Thus, a = 12

and a² = 144

We know that, b² = a²(1 – e²)

⇒ b² = 144(1 – (1/3)²)

⇒ b² = 144(8/9)

⇒ b² = 128

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{144}+\frac{y^2}{128}=1$

Thus, $\frac{x^2}{144}+\frac{y^2}{128}=1$ is the required equation.

问题 7. 求标准形式的椭圆的短轴等于焦点到直角的距离为 10 的方程。

解决方案：

Given that, the coordinates of foci are (±ae, 0).

2b = 2ae 

⇒ b = ae

⇒ b² = a²e² ….(i)

Also given that the latus rectum is 10

So, 2b²/a = 10 

b²= 5a  ….(ii)

As we know that b² = a²(1 – e²) 

⇒ b² = a²(1 – e²) 

⇒ b² = a² – a²e²

⇒ b² = a² – b²

⇒ 2b² = a²

⇒ b² = a²/2  ….(iii)

Now put the value of b² in eq(ii), we get

a²/2 = 5a 

a = 10 

So, a² = 100

Now put the value a² of in eq(iii), we get

b² = 100/2  

b² = 50 

On substituting the values of a² and b² in eq(i), we get,

$\frac{x^2}{100}+\frac{y^2}{50}=1$

Hence, x² + 2y² = 100 is the required equation.

问题 8. 当长轴 (i) 平行于 x 轴 (ii) 平行于 y 轴时，求中心为 (-2, 3) 且半轴为 3 和 2 的椭圆的方程.

解决方案：

(i) When the major axis is parallel to the x-axis 

Let us assume $\frac{(x-x_1)^2}{a^2}+\frac{(y-y_1)^2}{b^2}=1$be the equation.

So, on substituting the values x₁ = -2, y₁ = 3, a = 3, and b = 2 in the equation, we have:

$\frac{(x+2)^2}{9}+\frac{(y-3)^2}{4}=1$

Thus, 4x² + 9y² + 16x – 54y + 61 = 0 is the required equation.

(ii) When the major axis is parallel to the y-axis 

Let us assume $\frac{(x-x_1)^2}{a^2}+\frac{(y-y_1)^2}{b^2}=1$be the equation.

So, on substituting the values x₁ = -2, y₁ = 3, a = 2, and b = 3 in the equation, we have:

$\frac{(x+2)^2}{4}+\frac{(y-3)^2}{9}=1$

Thus, 9x² + 4y² + 36x – 24y + 36 = 0 is the required equation.

问题 9. 求椭圆的离心率，其：

(i) 直肠阔是其短轴的一半

解决方案：

Given that, 2b²/a = 2b/2

⇒ 2b² = ab

⇒ 2b = a

Since, $e=\sqrt{1-\frac{b^2}{4a^2}}$

⇒ $e=\sqrt{1-\frac{1}{4}}$

⇒ e = √3/2 

Hence, the eccentricity of an ellipse is √3/2 

(ii) 直肠阔是其长轴的一半

解决方案：

Given that, 2b²/a = 2a/2

⇒ 2b² = a²

Since, $e=\sqrt{1-\frac{b^2}{2b^2}}$

⇒ $e=\sqrt{1-\frac{1}{2}}$

⇒ e = 1/√2

问题 10. 求以下椭圆的中心、轴长、偏心率、焦点：

(i) x ² + 2y ² – 2x + 12y + 10 = 0

解决方案：

Given that x² + 2y² – 2x + 12y + 10 = 0 

(x² – 2x) + 2(y² + 6y) = -10

⇒ (x² – 2x + 1) + 2(y² + 6y + 9) = -10 + 18 + 1

⇒ $\frac{(x-1)^2}{9}+\frac{(y+3)^2}{\frac{9}{2}}=9$

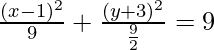
So, x₁ = 1, y₁ = -3

and a = 3  and b = 3/√2

Centre = (1, -3)

Major axis = 2a = 2(3) = 6

Minor axis = 2b = 3\sqrt2

e=$\sqrt{1-\frac{b^2}{a^2}}$

= 1/√2 

Foci = (1 ± 3/√2; -3) 

(ii) x ² + 4y ² – 4x + 24y + 31 = 0

解决方案：

Given that x² + 4y² – 4x + 24y + 31 = 0

(x² – 4x) + 4(y² + 6y) = -31

⇒ (x² – 4x + 4) + 4(y² + 6y + 9) = 9

⇒ $\frac{(x-1)^2}{9}+\frac{(y+3)^2}{\frac{9}{2}}=9$

So, x₁ = 1, y₁ = -3

and a = 3  and b = 3/2

Centre = (2, -3)

Major axis = 2a = 2(3) = 6

Minor axis = 2b = 3

e= $\sqrt{1-\frac{b^2}{a^2}}$

= √3/2  

Foci = (2 ± 3/√2; -3) 

(iii) 4x ² + y ² – 8x + 2y +1 = 0

解决方案：

Given that 4x² + y² – 8x + 2y +1 = 0

4(x² – 2x) + (y² + 2y) = -1

4(x² – 2x + 1) + (y² + 2y + 1) = -1 + 4 + 1

4(x² – 1) + (y² + 1) = 4

⇒ $\frac{(x-1)^2}{1}+\frac{(y+1)^2}{4}=1$

So, x₁ = 1, y₁ = -1

and a = 1  and b = 2

Centre = (1,-1)

Minor axis = 2a = 2(1) = 2

Minor axis = 2b = 4

e = $\sqrt{1-\frac{b^2}{a^2}}$

e = √3/2 

Foci = (1,  -1 ± √3) 

(iv) 3x ² + 4y ² – 12x – 8y + 4 = 0

解决方案：

Given that 3x² + 4y² – 12x – 8y + 4 = 0

3(x² – 4x) + 4(y² – 2y) = -4

3(x² – 4x + 4) + 4(y² – 2y + 1) = -4 + 12 + 4

⇒ $\frac{(x-2)^2}{4}+\frac{(y-1)^2}{3}=1$

So, x₁ = 2, y₁ = -1

and a = 2  and b = √3

Centre = (2, 1)

Major axis = 2a = 2(2) = 4

Minor axis = 2b = 2(√3) = 2√3

e = $\sqrt{1-\frac{b^2}{a^2}}$

e = 1/2  

Foci = (2 ±  1, 1) 

(v) 4x ² + 16y ² – 24x – 32y – 12 = 0

解决方案：

Given that 4x² + 16y² – 24x – 32y – 12 = 0

4(x² – 6x) + 16(y² – 2y) = 12

4(x² – 6x + 9) + 16(y² – 2y + 1) = 12 + 36 + 16

4(x – 3) + 16(y – 1) = 64

⇒ $\frac{(x-3)^2}{16}+\frac{(y-1)^2}{4}=1$

So, x₁ = 3, y₁ = 1

and a = 4  and b = 2

Centre = (3, 1)

Major axis = 2a = 2(4) = 8

Minor axis = 2b = 2(2) = 4

e = $\sqrt{1-\frac{b^2}{a^2}}$

e = √3/2  

Foci = (3 ±2√3; 1) 

(vi) x ² + 4y ² – 2x = 0

解决方案：

Given that x² + 4y² – 2x = 0

(x² – 2x) + 4y² = 0

(x² – 2x + 1) + 4y² = 0

(x – 1)² + 4y² = 0

⇒ $\frac{(x-1)^2}{1}+\frac{(y)^2}{\frac{1}{4}}=9$

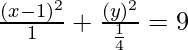
So, x₁ = 1, y₁ = 0

and a = 1  and b = 1/4

Centre = (1, 0)

Major axis = 2a = 2(1) = 2

Minor axis = 2b = 2(1/2) = 1

e = $\sqrt{1-\frac{b^2}{a^2}}$

e = √3/2 

Foci = (1 ±√3/2, 0)