第 11 类 RD Sharma 解决方案 - 第 26 章椭圆 - 练习 26.1 |设置 2

问题 11. 求焦点在 (±3, 0) 且通过 (4, 1) 的椭圆的方程。

解决方案：

Let the equation of the ellipse be $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ ….(i)

Given that the ellipse whose foci are at (±3, 0) and which passes through (4, 1)

So, 

ae = 3 

(ae)² = 9

y = 1 and  x = 4 

Substituting the values of x and y in the above equation, we have:

$\frac{16}{a^2}+\frac{1}{b^2}=1$ …(ii)

As we know that, b² = a²(1 – e²)

⇒ b² = a² – a²e²

⇒ b² = a² – 9 

or 

a² = b² + 9  ….(iii)

On solving eq(ii), we get

16b² + a²  = a²b²

Now put the value of a² from eq(iii), we get

16b² + b² + 9  = (b² + 9)b²

b⁴ – 8b² – 9  = 0

⇒ b = ±3

So, a = 3√2

Now put the value of a² and b² in eq(i), we get

$\frac{x^2}{18}+\frac{y^2}{9}=1$

Thus, $\frac{x^2}{18}+\frac{y^2}{9}=1$is the required equation. 

问题 12. 求一个椭圆的方程，其离心率为 2/3，直角为 5，圆心为原点。

解决方案：

Let the equation of the ellipse be $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ …..(i)

Given that 

eccentricity(e) = 2/3

latus rectum = 5 

So, 2b²/a = 5  …..(ii)

⇒ 2b² = 5a

⇒ 10a² = 45a

⇒ a = 9/2

On substituting the value of a in eq(ii), we have:

⇒ b² = 45/4

Now put the value of a² and b² in eq(i), we get

$\frac{4x^2}{81}+\frac{4y^2}{45}=1$

Thus, $\frac{4x^2}{81}+\frac{4y^2}{45}=1$is the equation of the ellipse.

问题 13. 求椭圆的方程，其焦点在 y 轴，偏心率为 3/4，中心在原点，通过 (6, 4)。

解决方案：

Let the equation of the plane be $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$

Given that 

eccentricity(e) = 3/4, 

As we know that  a² = b²(1 – e²)

⇒ $a^2=b^2(1-\frac{9}{16})$

⇒ $a^2=\frac{7}{16}b^2$

⇒ $\frac{x^2}{a^2}+\frac{7y^2}{16a^2}=1$

Since it passes through (6,4), we have:

⇒ $\frac{36}{a^2}+\frac{112}{16a^2}=1$

⇒ a² = 43 and b² = 688/7

Now put the value of a² and b² in eq(i), we get

$\frac{x^2}{43}+\frac{7y^2}{688}=1$

Thus $\frac{x^2}{43}+\frac{7y^2}{688}=1$is the required equation.

问题 14. 找出其轴位于坐标轴上并通过 (4, 3) 和 (-1, 4) 的椭圆的方程。

解决方案：

Let the equation of the ellipse be: $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ….(i)

It is given that the ellipse passes through (4, 3) and (-1, 4).

⇒ $\frac{16}{a^2}+\frac{9}{b^2}=1$and $\frac{1}{a^2}+\frac{16}{b^2}=1$

Let $p=\frac{1}{a^2}$and $r=\frac{1}{b^2}$

Then, 16p + 9r = 1 and p + 16b = 1

On solving these equations, we have:

$p=\frac{1}{a^2}=\frac{7}{247}$and $r=\frac{1}{b^2}=\frac{15}{247}$

Now by substituting all the values in eq(i), we have:

$\frac{7x^2}{247}+\frac{15y^2}{247}=1$

Thus, $\frac{7x^2}{247}+\frac{15y^2}{247}=1$is the required equation.

问题 15. 求一个椭圆的方程，其轴位于坐标轴上，通过点(-3, 1)，偏心率为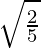$\sqrt{\frac{2}{5}}$ .

解决方案：

Let the equation of the ellipse be: $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ……(i)

It is given that the ellipse passes through the point (-3, 1).

So,

⇒ $\frac{9}{a^2}+\frac{1}{b^2}=1$

As we know that b² = a²(1 – e²)

Also the eccentricity(e) =  $\sqrt{\frac{2}{5}}$.

⇒ b² = a²(1 – 2/5)

⇒ b² = 3a²/5 

On substituting the values, we have:

$\frac{9}{a^2}+\frac{5}{3a^2}=1$

⇒ a² = 32/2

⇒ b² = 32/5

Now put the value of a² and b² in eq(i), we get

$\frac{x^2}{\frac{32}{2}}+\frac{y^2}{\frac{32}{5}}=1$

Thus, 3x² + 5y² = 32 is the required equation. 

问题 16. 求椭圆方程，焦点之间的距离是 8 个单位，准线之间的距离是 18 个单位。

解决方案：

Let the equation of the ellipse be: $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  ……(i)

Given that the distance between foci = 8 units

So, 2ae = 8  …(i)

Distance between directrices = 18 units

So, 2a/e = 18  …(i)

From eq(i) and (ii), we get

⇒ e = 8/2a

⇒ 4a² = 18(8)

⇒ a² = 36

⇒ a = 6

⇒ e = 2/3

Now, b² = a²(1 – e²)

⇒ b² = 36(1 – 4/9)

⇒ b² = 36(5/9)

⇒ b² = 20

Now put the values of a² and b² in eq(i), we get

$\frac{x^2}{36}+\frac{y^2}{20}=1$

Thus, $\frac{x^2}{36}+\frac{y^2}{20}=1$is the required equation.

问题 17. 求顶点为 (0, ±10) 且偏心率为 4/5 的椭圆的方程。

解决方案：

Let the equation of the ellipse be: $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$  …..(i)

As we know that the vertices of the ellipse are on the y- axis, so the coordinates of the vertices are (0, ±10).

Thus, b = 10

Since, a² = b²(1 – e²)

eccentricity(e) = 4/5 (given) 

⇒ a² = 100(1 – (4/5)²) 

⇒ a² = 100(1 – 16/25) 

⇒ a² = 36

Now put the values of a² and b² in eq(i), we get

$\frac{x^2}{36}+\frac{y^2}{100}=1$

Thus, 100x² + 36y²=3600 is the required equation.

问题 18. 一根长度为 12m 的杆在运动时其末端接触坐标轴。确定杆上点 P 的轨迹方程，该点距与 x 轴接触的末端 3 厘米。

解决方案：

Let us consider AB be the rod that make an angle θ with line OX and let P(x, y) be the point on it such that AP = 3 cm.

Then, PB = AB – AP = 12 – 3 = 9 cm

Thus, $cosθ=\frac{PQ}{PB}=\frac{x}{9}$

and, $sinθ=\frac{PR}{PA}=\frac{y}{3}$

As we know that sin²θ  + cos²θ = 1, we have:

$(\frac{y}{3})^2+(\frac{x}{9})^2=1$

Thus $\frac{x^2}{81}+\frac{y^2}{9}=1$is the locus of the point P. 

问题 19. 找出距 (0, 4) 的距离为距直线 y = 9 距离的三分之二的所有点的集合的方程。

解决方案：

Given that PQ = 2/3PL 

So,

⇒ $\sqrt{(x-0)^2+(y-4)^2}=\frac{2}{3}(y-9)$

⇒ 3²[x² + (y – 4)²] = 2²(y – 9)²

⇒ 9x² + 9y² – 72y + 144 = 4y² – 72y + 324

⇒ 9x² + 5y² = 180

Thus $\frac{x^2}{20}+\frac{y^2}{36}=1$is the required equation.