📌  相关文章
📜  圆公式的扇形面积

📅  最后修改于: 2022-05-13 01:57:53.653000             🧑  作者: Mango

圆公式的扇形面积

扇形是圆的一部分，由它的两个半径和连接它们的圆弧界定。半圆代表半个圆，是圆中最常见的扇区。圆的扇形是由圆弧及其两个半径形成的圆的饼形截面。当圆的一段圆周（也称为圆弧）和两个半径在圆弧的两端相交时，就会产生一个扇形。

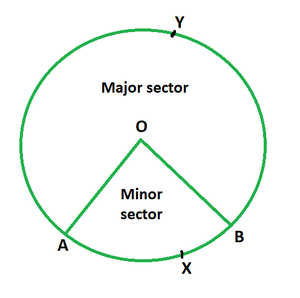
在上图中，小扇区是圆 OAXB 的部分，而大扇区是圆 OAYB 的部分。

扇区面积

圆的扇形面积是圆的边界扇形内占用的空间量。一个扇区总是从圆的中心开始。半圆同样是圆的一个扇形；在这种情况下，一个圆有两个大小相等的扇区。

公式

推导

示例问题

问题 1. 如果扇形的角度为 30°，则求半径为 5 cm 的给定圆的扇形面积。

解决方案：

问题 2。如果扇形的角度为 45°，则求半径为 9 cm 的给定圆的扇形面积。

解决方案：

问题 3. 求给定半径为 15 cm 的圆的扇形面积，如果扇形的角度是 π/2 弧度。

解决方案：

问题 4. 如果扇形面积为 770 平方厘米，半径为 7 厘米，求圆心对角。

解决方案：

问题 5. 如果圆的扇形面积为 132 平方厘米，圆的中心角为 60°，求圆的面积。

解决方案：