第 12 类 RD Sharma 解——第 8 章联立线性方程组的解——练习 8.1 |设置 2
问题 8. (i) 如果 A = 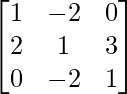 ,求 A -1 。使用 A -1求解线性方程组 x - 2y = 10, 2x + y + 3z = 8, -2y + z = 7。
,求 A -1 。使用 A -1求解线性方程组 x - 2y = 10, 2x + y + 3z = 8, -2y + z = 7。
解决方案:
Here,
A = 
|A| = 1 (1 + 6) + 2 (2 – 0) + 0 (- 4 – 0)
= 7 + 4 + 0
= 11
Let Cij be the cofactors of the elements aij in A.
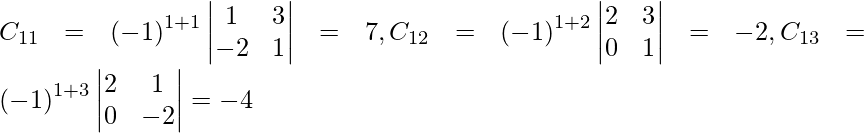
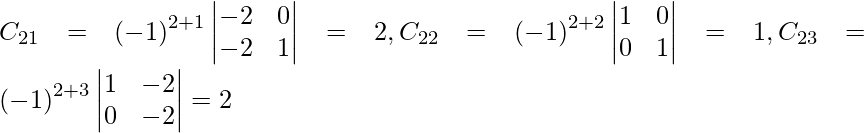

adj A = 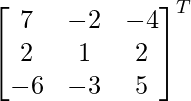
= 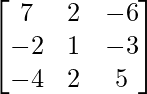
![]()
= 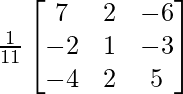
Now, X = A-1 B
X = 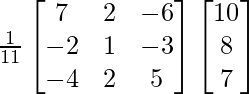
X = 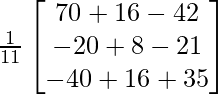
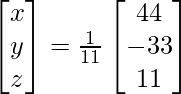
Therefore x = 4, y = -3 and z = 1.
(ii) 如果 A =  ,找到 A -1并因此求解以下方程组:
,找到 A -1并因此求解以下方程组:
解决方案:
Here,
A = 
|A| = 9 – 12 – 6
= -9
Let Cij be the cofactors of the elements aij in A.


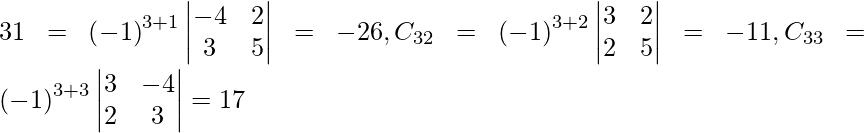
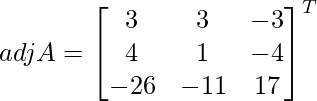
= 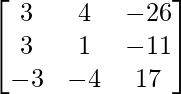
![]()
A = 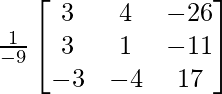
Here,
A = 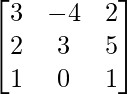 , X =
, X = ![]() and B =
and B = 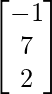
X = A-1 B
X = 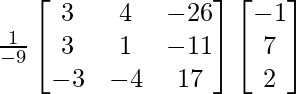
X = 
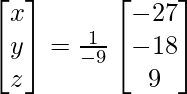
Therefore x = 3, y = 2 and z = – 1.
(iii) 如果 A = 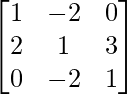 和 B =
和 B = 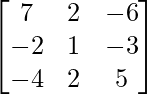 , 找到 AB。因此,求解方程组:x - 2y = 10、2x + y + 3z = 8 和 -2y + z = 7。
, 找到 AB。因此,求解方程组:x - 2y = 10、2x + y + 3z = 8 和 -2y + z = 7。
解决方案:
Here,
A =  , B =
, B = 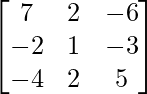
AB = 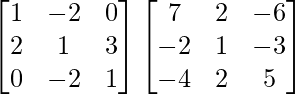
= 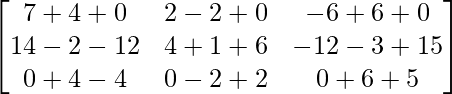
= 
= 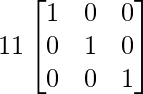
= 11 I
=> ![]()
![]()

X = A-1 B
X = 
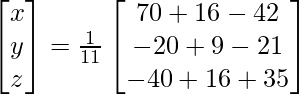
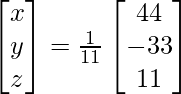
Therefore x = 4, y = -3 and z = 1.
(iv) 如果 A = 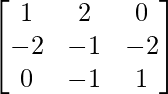 ,求 A -1 。使用 A -1求解线性方程组:x - 2y = 10, 2x - y - z = 8, -2y + z = 7。
,求 A -1 。使用 A -1求解线性方程组:x - 2y = 10, 2x - y - z = 8, -2y + z = 7。
解决方案:
Here,
A = 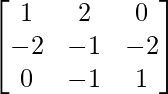
|A| = -3 + 4 + 0
= 1
Let Cij be the cofactors of the elements aij in A.
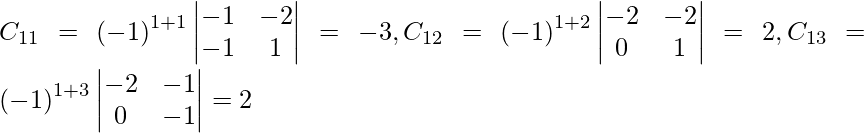
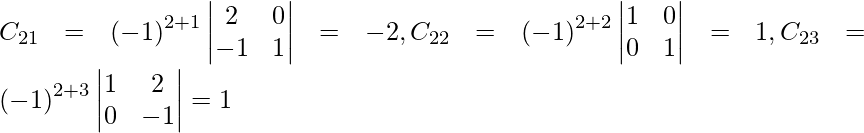

adj A = 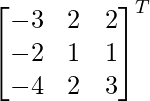
= 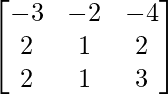
![]()
= 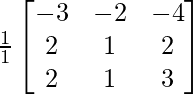
= 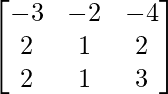
Now, X = A-1 B
X = 
X = 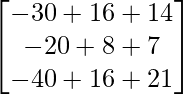

Therefore x = 0, y = – 5 and z = -3.
(v) 如果 A = 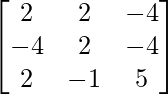 , B =
, B = 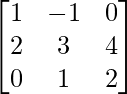 , 找到 BA 并用它来求解方程组 y + 2z = 7, x - y = 3, 2x + 3y + 4z = 17。
, 找到 BA 并用它来求解方程组 y + 2z = 7, x - y = 3, 2x + 3y + 4z = 17。
解决方案:
We have,
A = 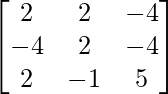 , B =
, B = 
BA = 
BA = 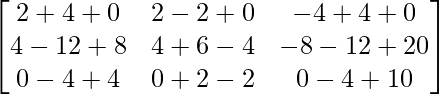
BA = 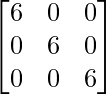
BA = 
BA = 6I
![]()
![]()
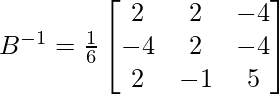
Now, BX = C. So, we have
X = B-1 C
X = 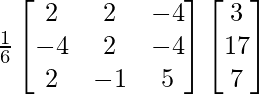
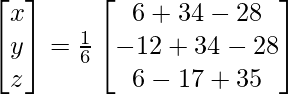
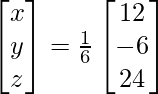
Therefore x = 2, y = – 1 and z = 4.
问题 9. 三个数的和是 2。如果第二个数的两倍加上第一个和第三个数的和,则和是 1。将第二个和第三个数加到第一个数的五倍,我们得到 6。使用矩阵求三个数。
解决方案:
Let the three numbers be x, y and z.
According to the question,
x + y + z = 2
x + 2y + z = 1
5x + y + z = 6
The given system of equations can be written in matrix form as,

A = 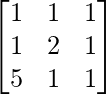 , X =
, X = ![]() and B =
and B = ![]()
|A| = 1 + 4 – 9
= -4
Let Cij be the cofactors of the elements aij in A.

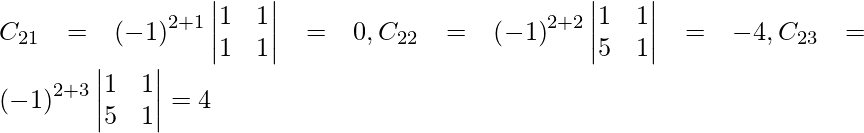

adj A = 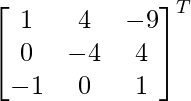
= 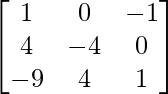
![]()
= 
X = A-1 B
X = 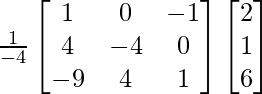
X = 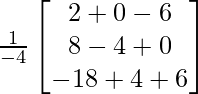
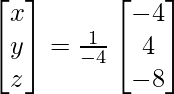
Therefore x = 1, y = – 1 and z = 2.
问题 10. 以每年 10%、12% 和 15% 的利率将 10,000 卢比投入三项投资。总收入为 1310 卢比,第一次和第二次投资的总收入为 190 卢比,低于第三次投资的收入。使用矩阵方法找到每个的投资。
解决方案:
Let the numbers are x, y, and z.
x + y + z = 10,000
0.1x + 0.12y + 0.15z = 1310
0.1x + 0.12y – 0.15z = – 190
The given system of equation can be written in matrix form as,
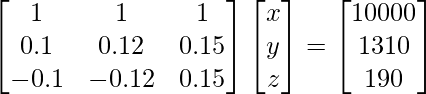
Here,
A = 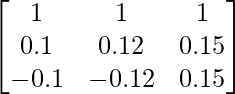 , X =
, X = ![]() and B =
and B = 
|A| = 0.036 – 0.03 + 0
= 0.006
Let Cij be the cofactors of the elements aij in A.
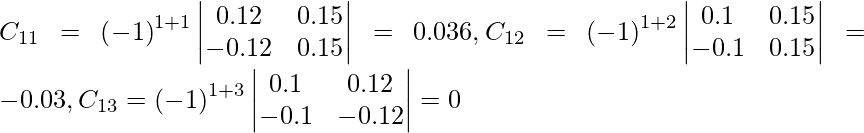

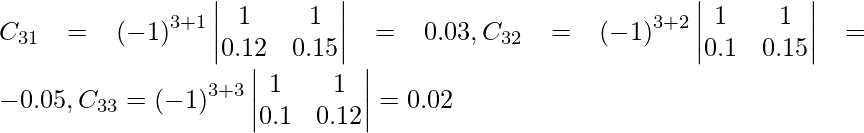
adj A = 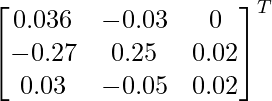
= 
![]()
= 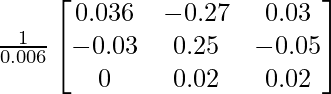
X = A-1 B
X = 
X = 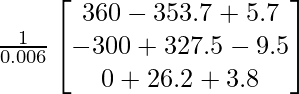
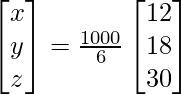
Therefore x = 2000, y = 3000 and z = 5000.
Thus, the three investments are of Rs 2000, Rs 3000 and Rs 5000, respectively.
问题 11. 一家公司每天生产三种产品。他们某一天的产量是45吨。发现第三种产品的产量比第一种产品的产量高8吨,而第一种和第三种产品的总产量是第二种产品产量的两倍。使用矩阵法确定每种产品的生产水平。
解决方案:
Let x, y and z be the production level of the first, second and third product, respectively .
x + y + z = 45
– x + z = 8
x – 2y + z = 0
The given system of equations can be written in matrix form as,
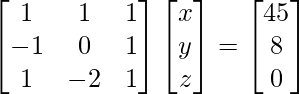
AX = B
A =  , X =
, X = ![]() , B =
, B = ![]()
Now,
|A| = 2 + 2 + 2
= 6
Let Cij be the cofactors of the elements aij in A.

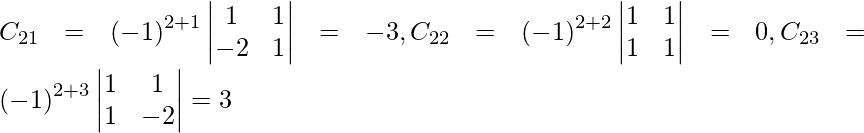

adj A = 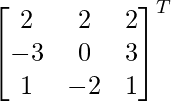
= 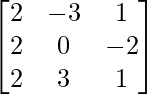
![]()
= 
X = A-1 B
X = 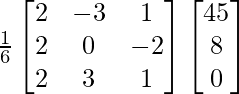
X = 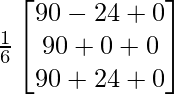
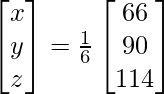
Therefore x = 11, y = 15 and z = 19.
Thus, the production level of first, second and third product is 11, 15 and 19, respectively.
问题 12. 三种商品 P、Q 和 R 的价格分别为每单位 Rs x、y 和 z。 A 购买 4 单位 R 并出售 3 单位 P 和 5 单位 Q。B 购买 3 单位 Q 并出售 2 单位 P 和 1 单位 R。C 购买 1 单位 P 并出售 4 单位 Q 和 6单位 R。在过程 A、B 和 C 中,分别赚取 6000 卢比、5000 卢比和 13000 卢比。如果卖出单位是正收益,买单位是负收益,用矩阵法求三种商品的单位价格。
解决方案:
The prices of three commodities P, Q and R are Rs x, Rs y and Rs z per unit, respectively.
3x + 5y – 4z = 6000
2x – 3y + z = 5000
-x + 4y + 6z = 13000
The given system of equations can be written in matrix form as follows:

AX = B
Here,
A = 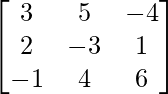 , X =
, X = ![]() , B =
, B = 
Now,
|A| = -66 – 65 – 20
= -151
Let Cij be the cofactors of the elements aij in A.
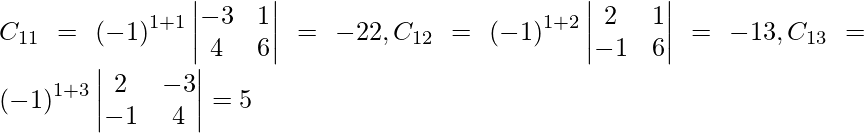
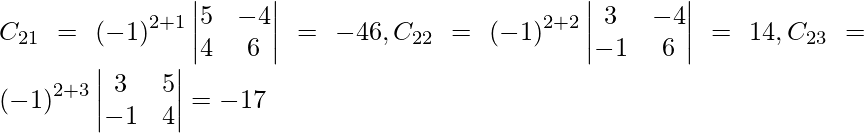
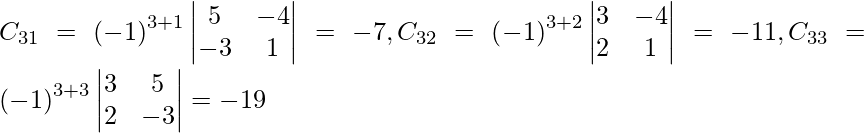
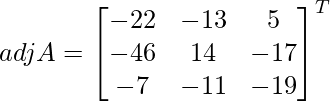
= 
![]()
= 
X = A-1 B
X = 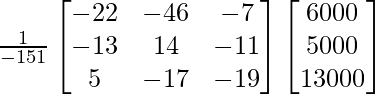
X = 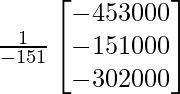
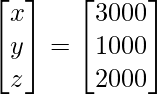
Therefore x = 3000, y = 1000 and z = 2000.
Thus, the prices of the three commodities P, Q and R are Rs 3000, Rs 1000 and Rs 2000 per unit, respectively.
问题 13. 一个居住区的管理委员会决定奖励一些成员(比如 x)诚实,一些(比如 y)帮助别人,还有一些(比如 z)监督工人保持殖民地整洁和干净的。全体获奖者之和为12。合作监督获奖者的三倍加上诚信获奖者的两倍之和为33。如果诚信监督获奖者人数之和为获奖者数量的两倍为了帮助他人,使用矩阵法,找出每个类别的获奖人数。除了这些价值观,即诚实、合作和监督之外,还表明了殖民地管理必须包括奖励的另一种价值观。
解决方案:
According to the question, we have
x + y + z = 12
2x + 3(y +z) = 33
x + z -2y = 0
The given system of equations can be written in matrix form as,
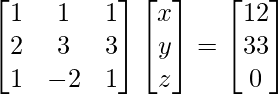
AX = B
Here,
A = 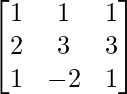 , X =
, X = ![]() and B =
and B = ![]()
Now, |A| = 3.
And adj A is given by 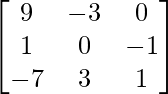 .
.

X = A-1 B
X = 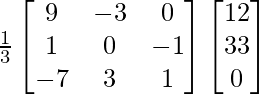
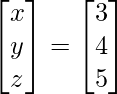
Therefore x= 3, y = 4 and z = 5.
Therefore, the number of awardees for Honesty, Cooperation and Supervision are 3, 4, and 5 respectively.
One more value which the management of the colony must include for awards may be Sincerity.
问题 14。一所学校希望以 6,000 卢比的现金奖励奖励其学生的诚实、规律和勤奋的价值观。辛勤工作的奖金加上诚实的奖金是 11,000 卢比的三倍。诚实和努力工作的奖金是规律性奖金的两倍。以代数方式表示上述情况,并使用矩阵方法找到每个值的奖金。除了这些价值观,即诚实、规律和努力工作之外,还提出了学校必须在奖励中包含的另一种价值观。
解决方案:
Let the award money given for Honesty, Regularity and Hard work be x, y and z respectively.
x + y + z = 6,000
x + 3 z = 11,000
x − 2y + z = 0
The given system of equations can be written in matrix form as,
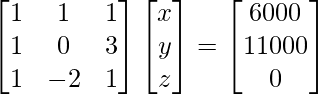
AX = B
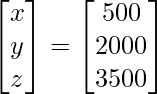
Here, A = 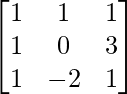 , X =
, X = ![]() and B =
and B = 
Now, |A| = 6.
And adj A is given by 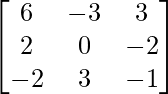 .
.
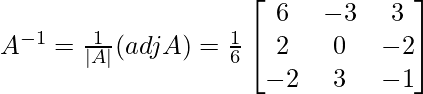
X = A-1 B
X = 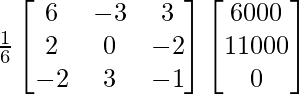
Therefore x = 500, y = 2000 and z = 3500.
Thus, award money given for Honesty, Regularity and Hard work are Rs 500, Rs 2000 and Rs 3500 respectively.
School can include sincerity for awards.
问题 15。两家机构决定以卢比的价格奖励其员工的机智、能力和决心这三个价值观。 x、y 和 z 分别为每人。第一个机构决定分别奖励4、3和2名员工,总价为卢比。 37000和第二机构决定分别奖励5名、3名和4名员工,总价款为卢比。 47000。如果每人的所有三个价格加起来为卢比。 12000 然后使用矩阵方法找到 x、y 和 z 的值。
解决方案:
According to the question, we have
4x + 3y + 2z = 37000
5x + 3y + 4z = 47000
x + y + z = 12000
We can express these equations as AX = B where, A = 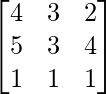 , X =
, X = ![]() and B =
and B = 
|A| = 4 (3 – 4) – 3 (5 – 4) + 2 (5 – 3)
= -4 – 3 + 4
= -3
adj A = 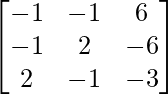

X = A-1 B
X = 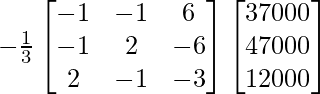
X = 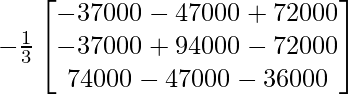


So, x = 4000 , y = 5000 and z = 3000.
问题 16. 两家工厂决定授予其员工三个价值观:(a) 适应新技术,(b) 在困难的情况下小心谨慎,以及 (c) 在紧张的情况下保持冷静,以 ₹ x, ₹ y 的比率, 和 ₹ 每人分别。一厂决定分别奖励2、4、3名员工,总奖金为29000卢比。二厂决定分别奖励5、2、3名员工,奖金为30500卢比。如果每人三项奖金加起来花费9500卢比,然后
(i) 用矩阵方程表示上述情况,用矩阵乘法形成线性方程。
(ii) 用矩阵法求解这些方程。
解决方案:
According to question,
2x + 3y + 4z = 29000
5x + 2y + 3z = 30500
x + y + z = 9500
The given system of equations can be written in matrix form as,
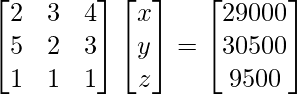


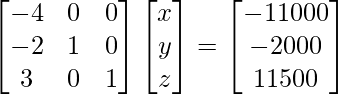

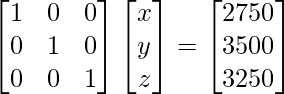
Therefore x = 2750, y = 3500 and z = 3250.
问题 17. A 和 B 两所学校希望以真诚、真实和乐于助人的价值观来奖励他们选择的学生。学校 A 希望分别向 3 名、2 名和 1 名学生分别奖励 3、2 和 1 名学生,每人 ₹x、每人 y 和 ₹z,总奖金为 ₹1,600。学校 B 希望花费 2,300 卢比来奖励其 4、1 和 3 名学生各自的价值观(通过与以前一样给予三个价值观相同的奖励)。如果每个值的一个奖项的总奖励金额为 900 卢比,则使用矩阵找到每个值的奖励金额。除了这三个值之外,建议另外一个值,应该考虑奖励。
解决方案:
Let the award money given for sincerity, truthfulness and helpfulness be ₹x, ₹y and ₹z respectively.
x + y + z = 900
3x + 2y + z = 1600
4x + y + 3z = 2300
The above system of equations can be written in matrix form as,
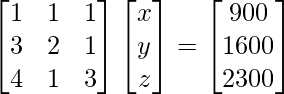
, where C = 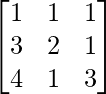 , X =
, X = ![]() and D =
and D = 
Now, |C| = 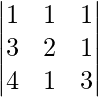
= 5 – 5 – 5
= -5
Let Cij be the cofactors of the elements aij in A.
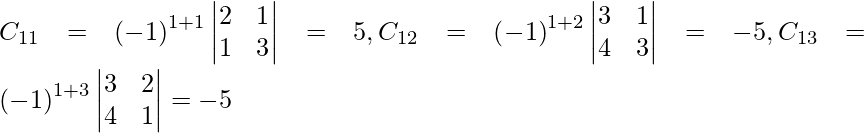
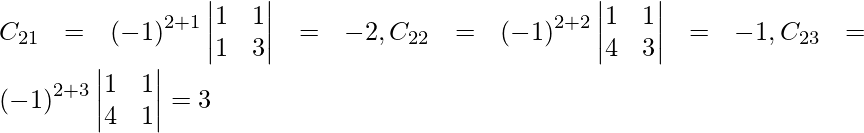
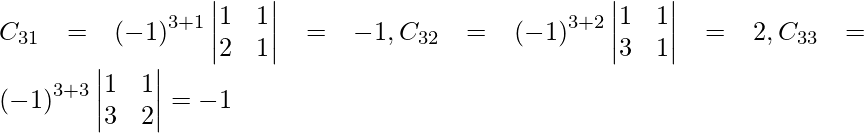
adj C = 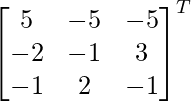
= 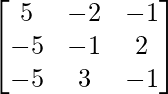
![]()
= 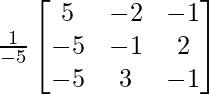
X = C-1 D
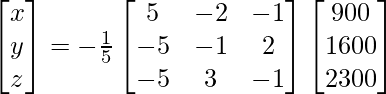

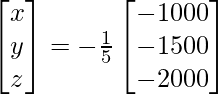
Therefore, x = -1000/-5, y = -1500/- 5 and z = -2000/-5
So, x = 200, y = 300 and z = 400.
Hence, the award money for each value of sincerity, truthfulness and helpfulness is ₹200, ₹300 and ₹400.
One more value which should be considered for award hardwork.
问题 18. P 和 Q 两所学校希望根据纪律、礼貌和守时的价值观来奖励他们选定的学生。学校 P 想要向其 3、2 和 1 名学生分别奖励 ₹x、每个 ₹y 和 ₹z,这三个值分别为 ₹1,000。 Q 学校希望花费 1,500 卢比来奖励其 4、1 和 3 名学生各自的价值观(通过与以前一样为三个价值观提供相同的奖励)。如果每个值的一个奖项的总奖励金额为 600 卢比,则使用矩阵找到每个值的奖励金额。除了以上三个值,建议多一个奖励值。
解决方案:
Let the award money given for Discipline, Politeness and Punctuality be ₹x, ₹y and ₹z respectively.
x + y + z = 600
3x + 2y + z = 1000
4x + y + 3z = 1500
The above system of equations can be written in matrix form AX = B as
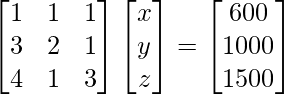
Here, A = 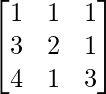 , X =
, X = ![]() and B =
and B = 
Now,
|A| = 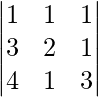
= 5 – 5 – 5
= -5
adj A = 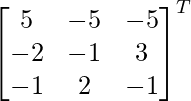
= 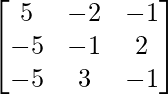
![]()
= 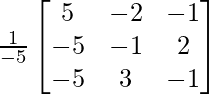
X = A-1 B
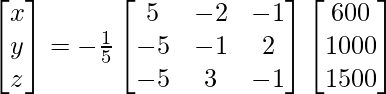

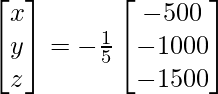
=> x = -500/- 5, y = -1000/- 5 and z = -1500/- 5
Therefore x = 100, y = 200 and z = 300.
Hence, the award money for each value of Discipline, Politeness and Punctuality is ₹100, ₹200 and ₹300.
One more value which should be considered for award is Honesty.
问题 19。两所学校 P 和 Q 想要奖励他们选择的学生宽容、善良和领导力的价值观。学校 P 希望分别向 3、2 和 1 名学生分别奖励 3、2 和 1 名学生,奖金总额为 2,200 卢比。 Q 学校希望花费 3,100 卢比来奖励其 4、1 和 3 名学生各自的价值观(通过向学校 P 的三个价值观提供相同的奖励)。如果每个值的一个奖项的总奖励金额为 1,200 卢比,则使用矩阵找到每个值的奖励金额。
除了这三个值之外,建议另外一个值,应该考虑奖励。
解决方案:
Let the award money given for Tolerance, Kindness and Leadership be ₹x, ₹y and ₹z respectively.
x + y + z = 1200
3x + 2y + z = 2200
4x + y + 3z = 3100
The above system of equations can be written in matrix form AX = B as
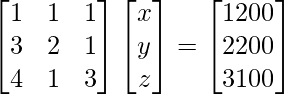
where, A =  , X =
, X = ![]() and B =
and B = 
Now,
|A| = 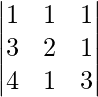
= 5 – 5 – 5
= -5
adj A = 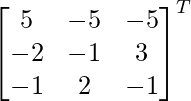
= 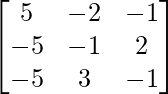
![]()
= 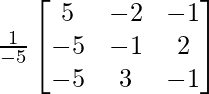
X = A-1 B

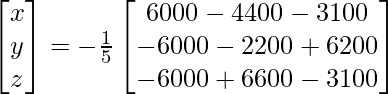
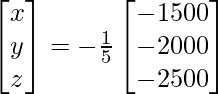
=> x = – 1500/-5, y = – 2000/-5 and z = -2500/-5
Therefore x = 300, y = 400 and z = 500.
Hence, the award money for each value of Tolerance, Kindness and Leadership is ₹300, ₹400 and ₹500.
One more value which should be considered for award is Honesty.
问题 20. 总计 7000 卢比存入三个不同的储蓄银行账户,年利率分别为 5%、8% 和 8.5%。这三个账户的年利息总额为 550 卢比。相等的金额已存入 5% 和 8% 的储蓄账户。在矩阵的帮助下,找出三个账户中每个账户的存款金额。
解决方案:
Let the amount deposited in each of the three accounts be ₹ x, ₹ x and ₹ y respectively.
2x + y = 7000
26x + 17y = 110000
The above system of equations can be written in matrix form AX = B as,
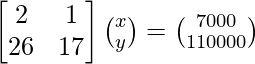
where, A = 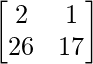 , X =
, X = ![]() and B =
and B = ![]()
Now,
|A| = 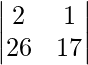
= 34 – 26
= 8
adj A = 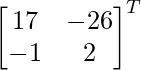
= 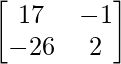
![]()
= 
X = A-1 B
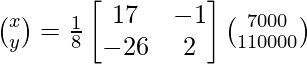
![]()
![]()
=> x = ![]() and y =
and y = ![]()
Therefore x = 1125 and y = 4750.
Hence, the amount deposited in each of the three accounts is ₹1125, ₹1125 and ₹4750.
问题 21. 一位店主有 3 种钢笔“A”、“B”和“C”。 Meenu 以 21 卢比的价格购买了每种品种的 1 支钢笔。Jeevan 以 60 卢比的价格购买了 4 支“A”品种、3 支“B”品种和 2 支“C”品种。而 Shikha 购买了 6 支“A”品种' 品种,2 支'B' 品种,3 支'C' 品种,售价 70 卢比。使用矩阵法,找出每种钢笔的成本。
解决方案:
Suppose there are 3 varieties of pen A, B and C
A + B + C = 21
4A + 3B + 2C = 60
6A + 2B + 3C = 70

where, P = 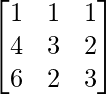 , Q =
, Q = ![]()
|P| = -5
Therefore, we get X = P-1 Q
adj P = 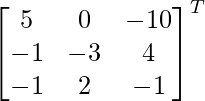
= 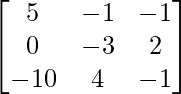
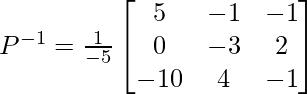
So, X = 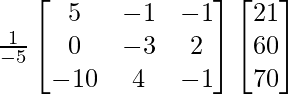
= 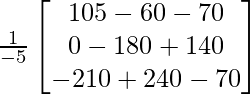
= 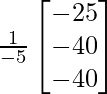
Therefore, X = ![]()
Therefore, cost of variety of pens for A, B and C is Rs 5, Rs 8 and Rs 8 respectively.