用平方根化简复分数

分数有两部分：分子和分母；分子是直线上方的数字，分母是直线下方的数字。在分数中，划分分子和分母的线或斜线表示除法。它用于显示我们与总数相比有多少组件。

复分数

分母和分子或两者都有分数的分数称为复分数。复有理表达式是包含变量的复分数。

例子： $\frac{3}{\frac{1}{2}}$ , $\frac{69}{\sqrt{420}}$ ， 等等。

用平方根化简复分数

脚步：

• 将分子和分母都乘以可以去除分母中根号的根号。例如，在表达式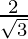$\frac{2}{\sqrt3}$ ，为了使分母有理化而要相乘的公根是√3。
• 如果可能，通过将项相乘并通过取出任何公因数进一步简化分子和分母来评估表达式。

示例：合理化分母： $\frac{4}{\sqrt6}$ .

解决方案：

Given: $\frac{4}{\sqrt6}$ 

Clearly, in order to rationalize the denominator, √6 needs to be multiplied with both the numerator and numerator.

= $\frac{4}{\sqrt6}×\frac{\sqrt{6}}{\sqrt6}$

= $\frac{4\sqrt{6}}{(\sqrt6)^2}$

= $\frac{4\sqrt{6}}{6}$

= 2√6/3

类似问题

问题 1. 合理化分母： $\frac{4\sqrt3}{2+\sqrt5}$ .

解决方案：

Multiply both the numerator and denominator with 2 − √5 to rationalize the denominator.

$\frac{4\sqrt3}{2+\sqrt5}×\frac{2-\sqrt5}{2-\sqrt5}$

Using the identity (a + b)(a − b) = a² − b², we have:

= $\frac{4\sqrt3(2-\sqrt5)}{2^2-(\sqrt5)^2}\\=\frac{8\sqrt3-4\sqrt{15}}{4-5}\\=\frac{8\sqrt3-4\sqrt{15}}{-1}\\=4\sqrt{15}-8\sqrt{3}$

问题 2. 合理化分母： $\frac{6+\sqrt3}{4-\sqrt3}$ .

解决方案：

Multiply both the numerator and denominator with 4 + √3 to rationalize the denominator.

$\frac{6+\sqrt3}{4-\sqrt3} ×\frac{4+\sqrt3}{4+\sqrt3}$

Using the identity (a + b)(a − b) = a² − b², we have:

= $\frac{6+\sqrt3(4+\sqrt3)}{4^2-(\sqrt3)^2}\\=\frac{24+10\sqrt3+3}{16-3}\\=\frac{27+10\sqrt3}{13}$

问题 3. 合理化分母： $\frac{1}{\sqrt5-2}$ .

解决方案：

Multiply both the numerator and denominator with √5 + 2 to rationalize the denominator.

$\frac{1}{2+\sqrt5}×\frac{√5 + 2}{√5 + 2}$

Using the identity (a + b)(a − b) = a² − b², we have:

= $\frac{\sqrt5+2}{(\sqrt5)^2-2^2}\\=\frac{\sqrt5+2}{5-4}\\=\sqrt{5}+2$

问题 4. 合理化分母： $\frac{6}{3+\sqrt7}$ .

解决方案：

Multiply both the numerator and denominator with 3 – √7 to rationalize the denominator.

$\frac{6}{3+\sqrt7}×\frac{3-\sqrt7}{3-\sqrt7}$

Using the identity (a + b)(a − b) = a² − b², we have:

= $\frac{6(3-\sqrt7)}{3^2-(\sqrt7)^2}\\=\frac{18-6\sqrt7}{9-7}\\=\frac{18-6\sqrt7}{2}\\=9-3\sqrt7$

问题 5. 合理化分母： $\frac{2}{\sqrt5-2}$ .

解决方案：

Multiply both the numerator and denominator with √5 + 2 to rationalize the denominator.

$\frac{2}{2+\sqrt5}×\frac{√5 + 2}{√5 + 2}$

Using the identity (a + b)(a − b) = a² − b², we have:

= $\frac{2\sqrt5+4}{(\sqrt5)^2-2^2}\\=\frac{2\sqrt5+4}{5-4}\\=2\sqrt{5}+4$

问题 6. 合理化分母： $\frac{7}{\sqrt5-2}$ .

解决方案：

Multiply both the numerator and denominator with √5 + 2 to rationalize the denominator.

$\frac{7}{2+\sqrt5}×\frac{√5 + 2}{√5 + 2}$

Using the identity (a + b)(a − b) = a² − b², we have:

= $\frac{7\sqrt5+14}{(\sqrt5)^2-2^2}\\=\frac{7\sqrt5+14}{5-4}\\=7\sqrt{5}+14$

问题 7. 合理化分母： $\frac{3}{\sqrt5}$ .

解决方案：

Given: $\frac{3}{\sqrt5}$

Clearly, in order to rationalize the denominator, √6 needs to be multiplied with both the numerator and numerator.

= $\frac{3}{\sqrt5}×\frac{\sqrt{5}}{\sqrt5}\\=\frac{3\sqrt{5}}{(\sqrt5)^2}\\=\frac{3\sqrt{5}}{5}$