平均自由程 – 定义、公式、推导、示例
引入动力学理论来解释分子相对于亚微观粒子的结构和组成。该理论讨论了由于亚微观粒子的不断运动和碰撞而导致的压力增加。它还讨论了气体的其他性质,例如温度、压力、体积、粘度、扩散、导热性等。该理论建立了微观粒子与宏观性质之间的关系。气体分子总是在不断地运动,并不断地相互碰撞并与容器壁碰撞,在这种情况下,学习气体的动力学既困难又重要。
物质的分子性质
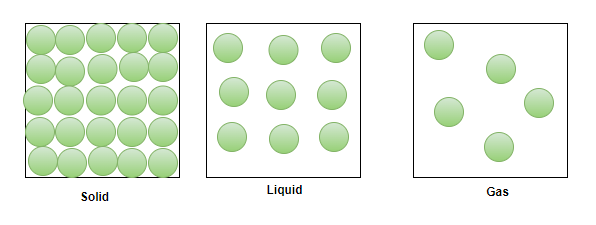
众所周知,物质存在三种形式,即固体、液体和气体。固体分子紧密堆积,它们之间的分子间空间非常小。在液体中,分子的堆积相对较不紧密,因此它们之间的分子间空间相对较大。另一方面,气体具有非常松散堆积的分子,与其他状态相比,它们之间的分子间距离最大。
自由路径
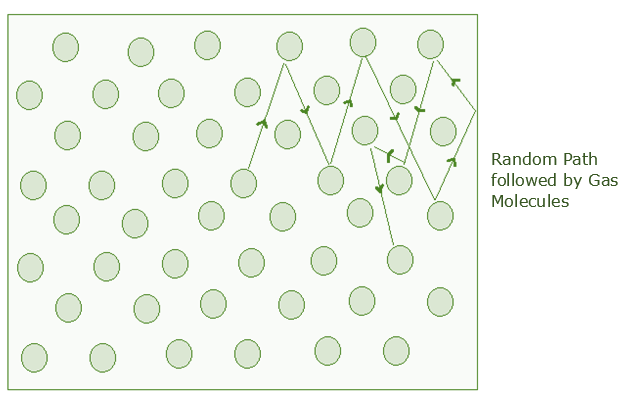
Let’s understand what is a free path. By definition, a free path is a distance between two consecutive collisions. As known through the behavior of gases, the molecules of a gas are always in constant motion.
它们相互碰撞以及与容器壁碰撞。假设分子 1 首先与分子 2 碰撞,然后与分子 3 碰撞,依此类推。当分子 1 与分子 2 碰撞时,称为第一次碰撞。当它与分子 3 碰撞时,称为第二次碰撞,以此类推。
第一次和第二次碰撞之间的距离称为自由路径,表示为λ1 ,第二次和第三次碰撞之间的距离称为λ2 ,以此类推。由于任何两个连续碰撞之间没有碰撞,因此,该路径被称为自由路径(λ 1 、λ 2 、λ 3等)。
平均自由路径
平均自由路径
The mean free path is the average path covered by the molecules between collisions. It is known that there are different free paths with different path lengths. Given below are the free paths,
λ1 = First free path
λ2 = Second free path
λ3 = Third free path
λn = nth free path
The average of these path lengths is known as the mean free path. Therefore, the mean free path can (denoted by λ) be calculated as,
λ = (λ1 + λ2 + λ3 + … λn)/n
平均自由程公式
让我们以直径为 d 的单个分子为例。想象它穿过其他分子,考虑到其他分子仍然没有碰撞,分子以圆柱形形式覆盖一定距离。截面积为πd2。圆柱体的体积是 πd2 × vt,其中 v 是分子的速度,t 是时间。让我们考虑每单位体积的分子数是 N/V。平均自由程可以写为,
λ = 路径长度/碰撞次数
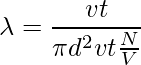

由于没有考虑其他分子的特性,分子和分母的速度不同。分子中的速度是平均的,分母中的速度是相对的。因此,在分母上加上了一个 √2 的因子。
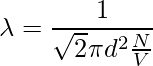
两次连续碰撞之间的时间,
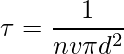
两次连续碰撞之间的平均距离,

示例问题
问题 1:求氧分子在 200K 和 1 个大气压下在空气中的平均自由程。氧分子的直径为1.5×10 -6 m。
解决方案:
As the formula for the mean free path is already known, that is,
λ = ![]()
N/V is the number density that can be equated to P/KT by ideal gas law,
Therefore,
λ = ![]()
λ = 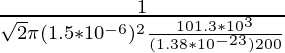
λ = 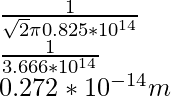
问题 2:求氧分子在 1 个大气压下以 100 K 在空气中的平均自由程。氧分子的直径为2×10 -6 m。
解决方案:
As the formula for the mean free path is already known, that is,
λ = ![]()
N/V is the number density that can be equated to P/KT by ideal gas law,
Therefore,
λ = ![]()
λ = 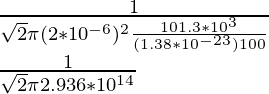
λ = 0.076 × 10-14
λ = 7.6 × 10-16m
问题 3:求氧分子在 1 个大气压下以 150 K 在空气中的平均自由程。氧分子的直径为1×10 -6 m。
解决方案:
As the formula for the mean free path is already known, that is,
λ = ![]()
N/V is the number density that can be equated to P/KT by ideal gas law,
Therefore,
λ = ![]()
λ = 
λ = 2.17 × 10-14
λ = 2.17 × 10-14m
问题 4:如果分子的平均自由程为 1.5 × 10 -10 m。找出当分子的直径为 1.2 × 10 -6 m 时,分子在 2 个大气压下以帕斯卡为单位的温度是多少。
解决方案:
As the formula for the mean free path is already known, that is,
λ = ![]()
N/V is the number density that can be equated to P/KT by ideal gas law,
Therefore,
λ = ![]()
1.5 × 10-10 = 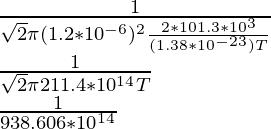
(1.5 × 10-10)(938.606 × 1014) = 1/T
1407.909 × 104 = 1/T
T = 7.10 K
问题 5:如果分子的平均自由程为 1 × 10 -6 m。找出当分子直径为 2 × 10 -9 m 时,分子以 1 个大气压行进的帕斯卡温度。
解决方案:
As the formula for the mean free path is already known, that is,
λ = ![]()
N/V is the number density that can be equated to P/KT by ideal gas law,
Therefore,
λ = ![]()
1 × 10-6 = 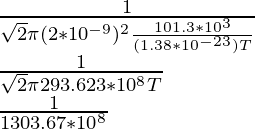
(1 × 10-6)(1303.67 × 108) = 1/T
1303.67 × 102 = 1/T
T = 7.6 × 10-6