高度和距离–三角| 10级数学

高度和距离是三角学的主要应用，在现实生活中被广泛使用。三角函数对于解决与高度和距离有关的问题时，对天文学家，导航员，建筑师和测量师等有用。

在解决问题之前，我们使用了一些术语来解决问题。他们是：

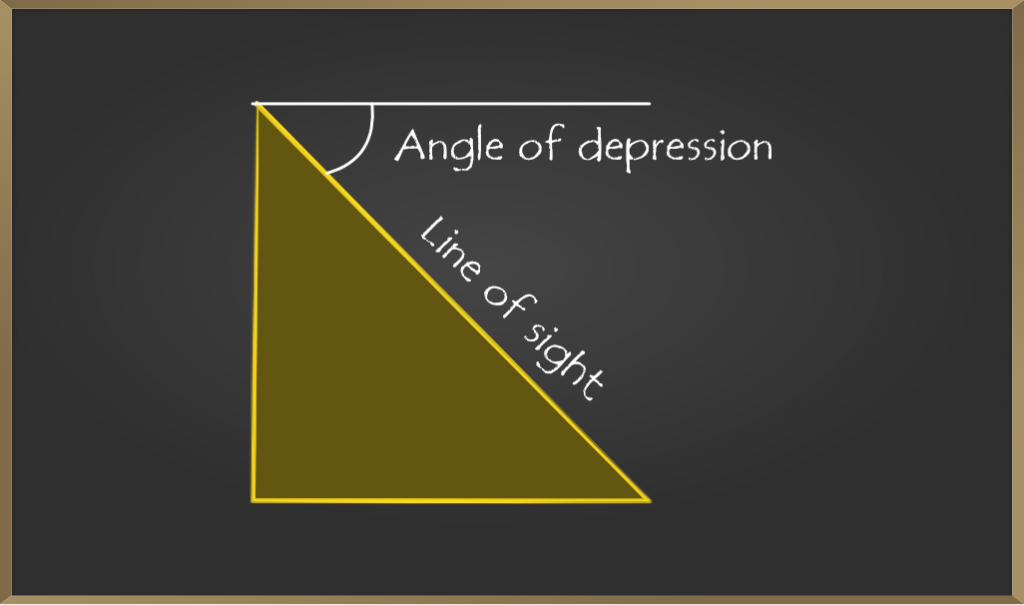
• 视线：这是从观察者的眼睛到观察者所观察的对象中的点所画的线。
• 印象角：水平线与将观察点连接到高架物体的视线之间的角度称为仰角。

• 俯角：水平与将观察点连接到水平线以下物体的视线之间的角度称为俯角。

高度和距离的样本问题

问题1：如果6 m高的杆在地面上投下2√3m长的阴影，请找出太阳的高程。

解决方案：

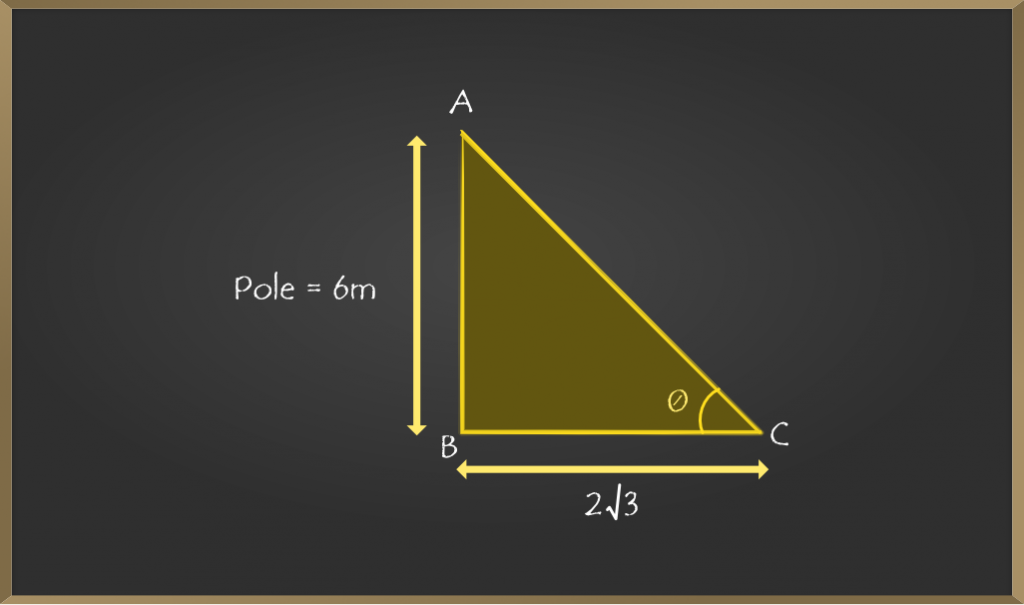
Let AB be the pole which is of height 6 m.

Let BC be the shadow of the building 2√3.

Now, in ∆ ABC,

tan θ = AB / BC

=> tan θ = 6 / 2√3

Now, simplifying using rationalization

=> tan θ = (3 / √3)*(√3 / √3)              

=> tan θ = 1 / √3

=> θ = tan^-1(1 / √3)

Hence, θ = 60^o

Therefore, sun’s elevation from the ground is 60^o.

问题2： 1.5 m高的观察者与22 m高的塔楼相距20.5 m。从观察者的角度确定塔顶的仰角。

解决方案：

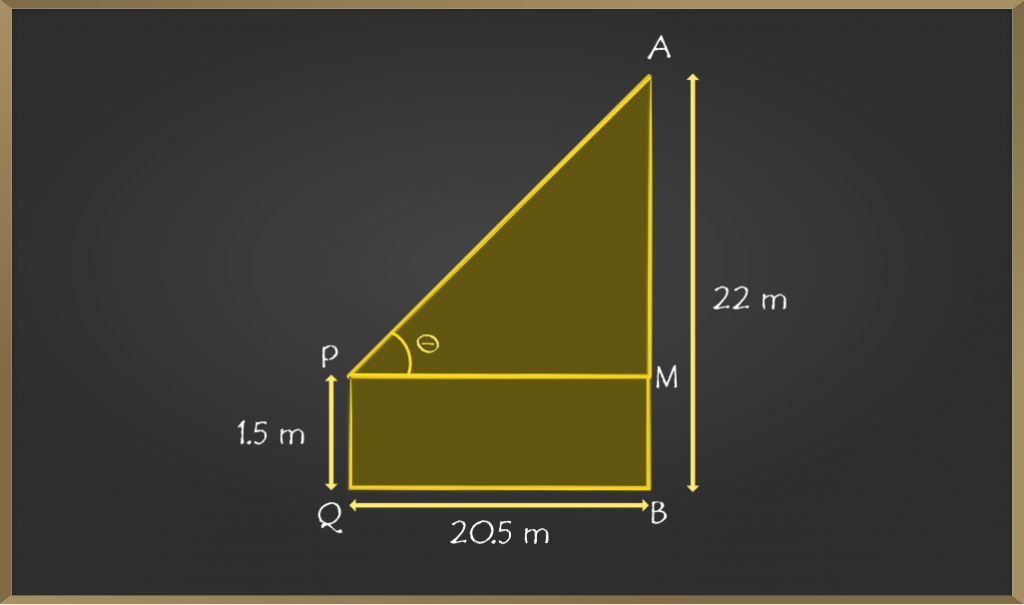
Let PQ be the height of the observer of 1.5 m.

Let AB be the height of the tower of 22 m.

And, let QB be the horizontal distance between the observer and the tower  

=> PQ = MB = 1.5 m

=> AM = AB – MB

=> AM = 22 – 1.5 = 20.5

Now, in ∆APM,

=> tan θ = AM / PM

=> tan θ = 20.5 / 20.5

=> tan θ = 1

=> θ = tan^-1(1 )

Hence, θ = 45^o

Therefore, the angle of elevation of the top of the tower from the eye of the observer is 45^o

问题3：一架飞机在地面上方飞行了几米。在特定瞬间，飞机从坐在地上的男孩的眼睛仰角为60°。一段时间后，仰角更改为30°。假设飞机沿直线行驶，求出该时间段内飞机所覆盖的距离。

解决方案：

Let x be the horizontal distance between the observer and plane at the first instant.

Let y be the horizontal distance between the observer and plane at the second instant.

And, BA = CD = h 

In ∆OAB,

=> tan 60° = AB / OA

=> √3 = h / x

=> x = h / √3

In ∆ OCD,

=> tan 30° = CD / OD

=> 1/√3 = h / (x+y)

=> x + y = √3h

Distance travelled by plane = AD = y

=> (x + y) − x = √3h − h / √3

=> y = (2 / √3)h

So, if the airplane is flying h meters above the ground, it would travel for (2/√3) h meters as the angle of elevation changes from 60° to 30°.

问题4：从塔顶的30 m高处，一个人正以30度的俯角观察一棵树的底部。找到树和塔之间的距离。

解决方案：

In the above diagram AB represents the height of the tower, BC represents the distance between the foot of the tower and the foot of the tree.

Now we need to find the distance between the foot of the tower and the foot of the tree (BC). For that as angle of depression is given so by vertically opposite angle property of triangle ∠CAD = ∠BCA

In ∆BCA,

=> tan θ = Opposite side / Adjacent side

=> tan 30° = AB / BC

=> 1/√3 = 30 / BC

=> BC = 30√3

=> BC = 30 (1.732)      [Approximately]

=> BC = 51.96 m

So, the distance between the tree and the tower is 51.96 m.

问题5：从高30 m的建筑物顶部看，塔的顶部和底部的俯角分别为30°和45°。找到塔的高度。

解决方案：

Let AB be the building and CD be the tower.

The angle of depressions is given 30° and 45° to the top and bottom of the tower. So by vertically opposite triangle property ∠FBD = ∠EDB and ∠FBC = ∠ACB.

Now, AB = 30 m. Let DC = x.

Draw DE perpendicular AB. Then AE = CD = x.

Therefore  BE = (30 – x) m.

In ∆ACB,

=> cot θ = Adjacent side / Opposite side

=> cot θ = AC / AB

=> cot 45° = AC / 30

=> AC = 30              [cot 45° = 1]

So, DE = AC = 30 m 

 In ∆EDB,

 => tan θ = Opposite side / Adjacent side

=> tan 30° = BE / DE

=> 1/√3 = BE / 30

=> BE = 30 / √3

=> CD = AE = AB – BE = 30 – (30 / √3)

=> 30[1 – (1 / √3) ] m

Height of the tower is 30[1 – (1 / √3) ] m