11类NCERT解决方案-第11章圆锥曲线部分-练习11.4

在练习1到6中的每个练习中，找到双曲线的焦点和顶点的坐标，偏心率和直肠的长度。

问题1 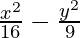$\frac{x^2}{16} - \frac{y^2}{9}$ = 1

解决方案：

Comparing the given equation with $\mathbf{\frac{x^2}{a^2} - \frac{y^2}{b^2}}$ = 1, 

we conclude that transverse axis is along x-axis.

a² = 16 and b² = 9

a = ±4 and b = ±3

Foci:

Foci = (c, 0) and (-c, 0)

c = √(a²+b²)

c = √(16+9)

c = √25 

c = 5

So the foci is (5, 0) and (-5, 0)

Vertices:

Vertices = (a, 0) and (-a, 0)

So the vertices is (4, 0) and (-4, 0)

Eccentricity:

Eccentricity = c/a = 5/4

Length of the latus rectum:

Length of the latus rectum = 2b²/a

= 2×9/4

= 9/2

问题2。 $\frac{y^2}{9} - \frac{x^2}{27}$ = 1

解决方案：

Comparing the given equation with $\mathbf{\frac{y^2}{a^2} - \frac{x^2}{b^2}}$ = 1, 

we conclude that transverse axis is along y-axis.

a² = 9 and b² = 27

a = ±3 and b = ±3√3

Foci: 

Foci = (0, c) and (0, -c) 

c = √(a² + b²)

c = √(9 + 27)

c = √36

c = 6

So the foci is (0,6) and (0,-6)

Vertices:

Vertices = (0,a) and (0,-a)

So the vertices is (0,3) and (0,-3)

Eccentricity:

Eccentricity = c/a

= 6/3

= 2

Length of the latus rectum:

Length of the latus rectum = 2b²/a

= 2×27/3

= 18

问题3. 9y ² – 4x ² = 36

解决方案：

9y² – 4x² = 36

On dividing LHS and RHS by 36,

9y²/36 – 4x²/36 = 36/36

$\mathbf{\frac{y^2}{4} - \frac{x^2}{9}}$ = 1 

Comparing the given equation with $\mathbf{\frac{y^2}{a^2} - \frac{x^2}{b^2}}$= 1, 

we conclude that transverse axis is along y-axis.

a² = 4 and b² = 9

a = ±2 and b = ±3

Foci:

Foci = (0, c) and (0, -c)

c = √(a² + b²)

c = √(4 + 9)

c = √13

So the foci is (0, √13) and (0, -√13)

Vertices:

Vertices = (0, a) and (0, -a)

So the vertices is (0, 2) and (0, -2)

Eccentricity:

Eccentricity = c/a

= √13/2

Length of the latus rectum:

Length of the latus rectum = 2b²/a

= 2×9/2

= 9

问题4. 16x ² – 9y ² = 576

解决方案：

16x² – 9y² = 576

On dividing LHS and RHS by 576,

16x²/576 – 9y²/576 = 576/576

$\mathbf{\frac{x^2}{36} - \frac{y^2}{64}}$ = 1 

Comparing the given equation with $\mathbf{\frac{x^2}{a^2} - \frac{y^2}{b^2}}$= 1, 

we conclude that transverse axis is along x-axis.

a² = 36 and b² = 64

a = ±6 and b = ±8

Foci:

Foci = (c,0) and (-c,0)

c = √(a² + b²)

c = √(36 + 64)

c = √100

c = 10

So the foci is (10, 0) and (-10, 0)

Vertices:

Vertices = (a, 0) and (-a, 0)

So the vertices is (6, 0) and (-6, 0)

Eccentricity:

Eccentricity = c/a

= 10/6 = 5/3

Length of the latus rectum:

Length of the latus rectum = 2b²/a

= 2×64/6

= 64/3

问题5. 5Y ² – 9X ² = 36

解决方案：

5y² – 9x² = 36

On dividing LHS and RHS by 36,

5y²/36 – 9x²/36 = 36/36

$\mathbf{\frac{y^2}{\frac{36}{5}} - \frac{x^2}{4}} = 1$

Comparing the given equation with $\mathbf{\frac{y^2}{a^2} - \frac{x^2}{b^2}}$= 1,

we conclude that transverse axis is along y-axis.

a² = 36/5 and b² = 4

a = ±6/√5 and b = ±2

Foci: 

Foci = (0, c) and (0, -c)

c = √(a² + b²)

c = √(36/5 + 4)

c = √56/5

c = 2√14/√5

So the foci is (0, 2√14/√5) and (0, -2√14/√5)

Vertices: 

Vertices = (0, a) and (0, -a)

So the vertices is (0,6/√5) and (0,-6/√5)

Eccentricity:

Eccentricity = c/a

= (2√14/√5)/6/√5

= √14/3

Length of the latus rectum:

Length of the latus rectum = 2b²/a

= 2×4/(6/√5)

= 4√5/3

问题6. 49y ² – 16x ² = 784

解决方案：

49y² – 16x² = 784

On dividing LHS and RHS by 784, we get

49y²/784 – 16x²/784 = 784/784

$\mathbf{\frac{y^2}{16} - \frac{x^2}{49}} = 1$

Comparing the given equation with $\mathbf{\frac{y^2}{a^2} - \frac{x^2}{b^2}}$= 1, 

we conclude that transverse axis is along y-axis.

a² = 16 and b² = 49

a = ±4 and b = ±7

Foci:

Foci = (0, c) and (0, -c)

c = √(a² + b²)

c = √(16 + 49)

c = √65

So the foci is (0, √65) and (0, -√65)

Vertices:

Vertices = (0, a) and (0, -a)

So the vertices is (0, 4) and (0, -4)

Eccentricity:

Eccentricity = c/a = √65/4

Length of the latus rectum:

Length of the latus rectum = 2b²/a

= 2×49/4

= 49/2

在练习7至15中，找到满足给定条件的双曲线方程。

问题7.顶点(±2，0)，焦点(±3，0)。

解决方案：

Since the foci is on x-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{x^2}{a^2} - \frac{y^2}{b^2}}$= 1

As, Vertices (± 2, 0) and foci (±3, 0)

So, a = ±2 and c = ±3

As, c = √(a² + b²)

b² = c² – a²

b² = 9 – 4

b² = 5

So, a² = 4 and b² = 5

Hence, the equation is 

$\mathbf{\frac{x^2}{4} - \frac{y^2}{5}}$ = 1

问题8.顶点(0，±5)，焦点(0，±8)。

解决方案：

Since the foci is on y-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{y^2}{a^2} - \frac{x^2}{b^2}}$= 1

As, Vertices (0, ±5) and foci (0, ±8)

So, a = ±5 and c = ±8

As, c = √(a² + b²)

b² = c² – a²

b² = 64 – 25

b² = 39

So, a² = 25 and b² = 39

Hence, the equation is

$\mathbf{\frac{y^2}{25} - \frac{x^2}{39}}$= 1

问题9.顶点(0，±3)，焦点(0，±5)。

解决方案：

Since the foci is on y-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{y^2}{a^2} - \frac{x^2}{b^2}}$= 1

As, Vertices (0, ± 3) and foci (0, ± 5)

So, a = ±3 and c = ±5

As, c = √(a² + b²)

b² = c² – a²

b² = 25 – 9

b² = 16

So, a² = 9 and b² = 16

Hence, the equation is

$\mathbf{\frac{y^2}{9} - \frac{x^2}{16}}$= 1

问题10。焦点(±5、0)，横轴长度为8。

解决方案：

Since the foci is on x-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{x^2}{a^2} - \frac{y^2}{b^2}}$= 1

As, Foci (±5, 0) ⇒ c = ±5

Since, the length of the transverse axis is 8,

2a = 8

a = 8/2

a = 4

As, c = √(a² + b²)

b² = c² – a²

b² = 25 – 16

b² = 9

So, a² = 16 and b² = 9

Hence, the equation is

$\mathbf{\frac{x^2}{16} - \frac{y^2}{9}}$= 1

问题11。焦点(0，±13)，共轭轴的长度为24。

解决方案：

Since the foci is on y-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{y^2}{a^2} - \frac{x^2}{b^2}}$= 1

As, Foci (0, ± 13) ⇒ c = ±13

Since, the length of the conjugate axis is 24,

2b = 24

b = 24/2

b = 12

As, c = √(a² + b²)

a² = c² – b²

a² = 169 – 144

a² = 25

So, a² = 25 and b² = 144

Hence, the equation is

$\mathbf{\frac{y^2}{25} - \frac{x^2}{144}}$= 1

问题12.焦点(±3√5，0)，胎儿直肠的长度为8。

解决方案：

Since the foci is on x-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{x^2}{a^2} - \frac{y^2}{b^2}}$= 1

As, Foci (±3√5, 0) ⇒ c = ±3√5

Since, the length of latus rectum is 8,

2b²/a = 8

b² = 8a/2

b² = 4a          -(1)

As, c = √(a² + b²)

b² = 45 – a²

4a = 45 – a²

a² + 4a – 45 = 0

a² + 9a – 5a – 45 = 0

(a + 9)(a – 5) = 0

a ≠ -9 (a has to be positive due to eq(1))

Hence, a = 5

From eq(1), we get

b² = 4(5)

b² = 20

So, a² = 25 and b² = 20

Hence, the equation is

$\mathbf{\frac{x^2}{25} - \frac{y^2}{20}}$= 1

问题13。焦点(±4，0)，胎儿直肠的长度为12。

解决方案：

Since the foci is on x-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{x^2}{a^2} - \frac{y^2}{b^2}}$= 1

As, Foci (±4, 0) ⇒ c=±4

Since, the length of latus rectum is 12,

2b²/a = 12

b² = 12a/2

b² = 6a            -(1)

As, c = √(a² + b²)

b² = 16 – a²

6a = 16 – a²

a² + 6a – 16 = 0

a² + 8a – 2a – 16 = 0

(a + 8)(a – 2) = 0

a ≠ -8 (a has to be positive due to eq(1))

Hence, a = 2

From eq(1), we get

b² = 6(2)

b² = 12

So, a² = 4 and b² = 12

Hence, the equation is

$\mathbf{\frac{x^2}{4} - \frac{y^2}{12}}$= 1

问题14.顶点(±7，0)，e = 4/3。

解决方案：

Since the vertex is on x-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{x^2}{a^2} - \frac{y^2}{b^2}}$= 1

As, Vertices (±7, 0) ⇒ a = ±7

As e = 4/3

c/a = 4/3

c = 4a/3

c = 28/3 

As, c = √(a² + b²)

b² = 784/9 – 49

b² = 343/9

So, a² = 49 and b² = 343/9

Hence, the equation is

x²/49 – y²/(343/9) = 1

$\mathbf{\frac{x^2}{49} - \frac{9y^2}{343}}$= 1

问题15.焦点(0，±√10)，经过(2，3)。

解决方案：

Since the foci is on y-axis, the equation of the hyperbola is of the form

$\mathbf{\frac{y^2}{a^2} - \frac{x^2}{b^2}}$= 1

As, Foci (0, ±√10) ⇒ c=±√10

As, c = √(a² + b²)

b² = c² – a²

b² = 10 – a²           -(1)

As (2, 3) passes through the curve, hence

3²/a² – 2²/b² = 1

9/a² – 4/b² = 1

9/a² – 4/(10 – a²) = 1

9(10 – a²) – 4a² = a²(10 – a²)

90  – 9a² – 4a² = 10a² – a⁴

a⁴ – 23a² + 90 = 0

a⁴ – 18a² – 5a² + 90 = 0

a²(a² – 18) – 5(a² – 18) = 0

(a² – 18)(a² – 5) = 0

a² = 18 or 5

As, a < c in hyperbola

So a² = 5

And, b² = 10 – 5            -(From eq(1))

b² = 5

So, a² = 5 and b² = 5

Hence, the equation is $\mathbf{\frac{y^2}{5} - \frac{x^2}{5}}$= 1